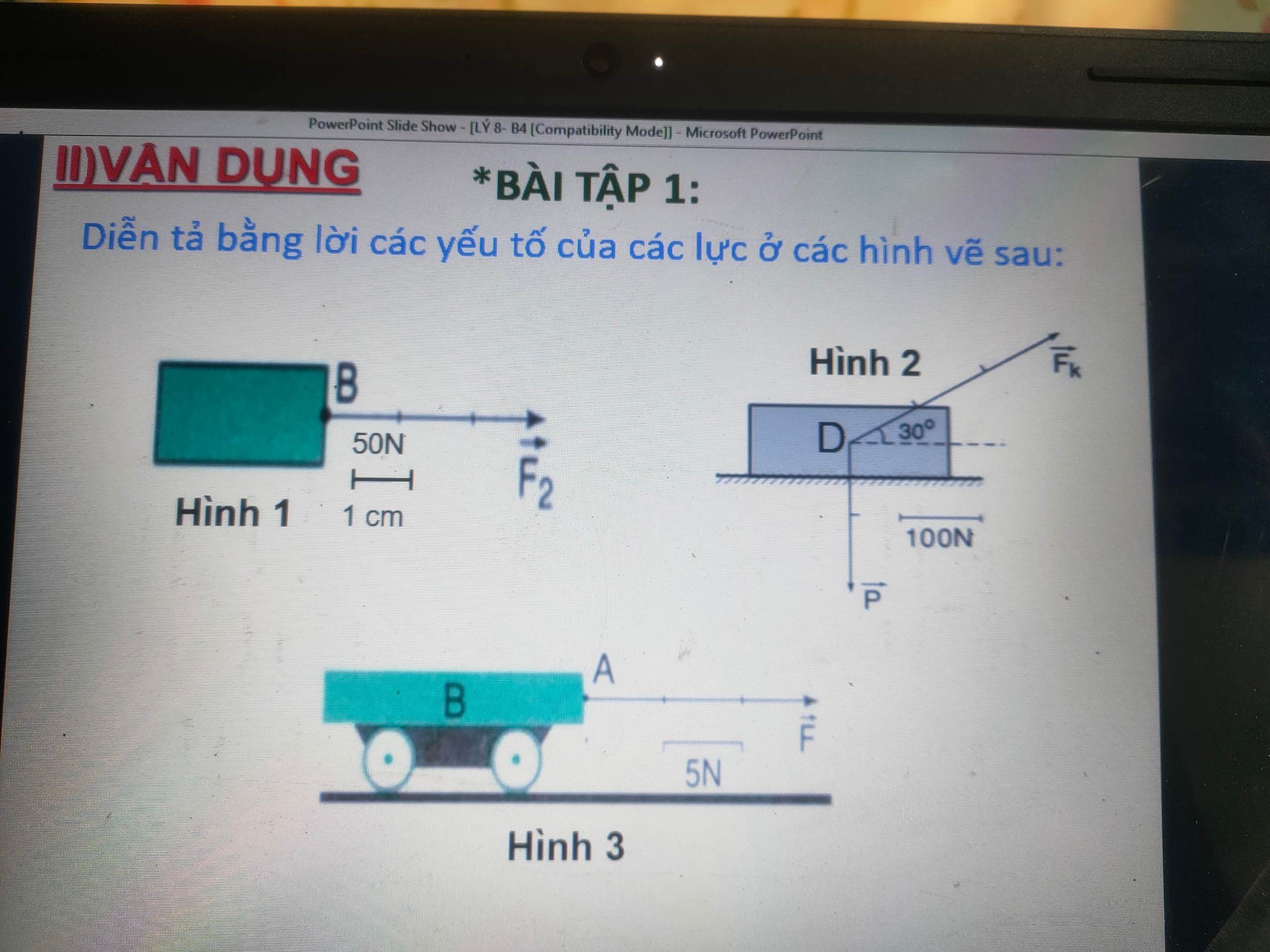
Giúp mik câu 5 vs, plss

Giúp mik vs plss
Giúp mik câu c với, plss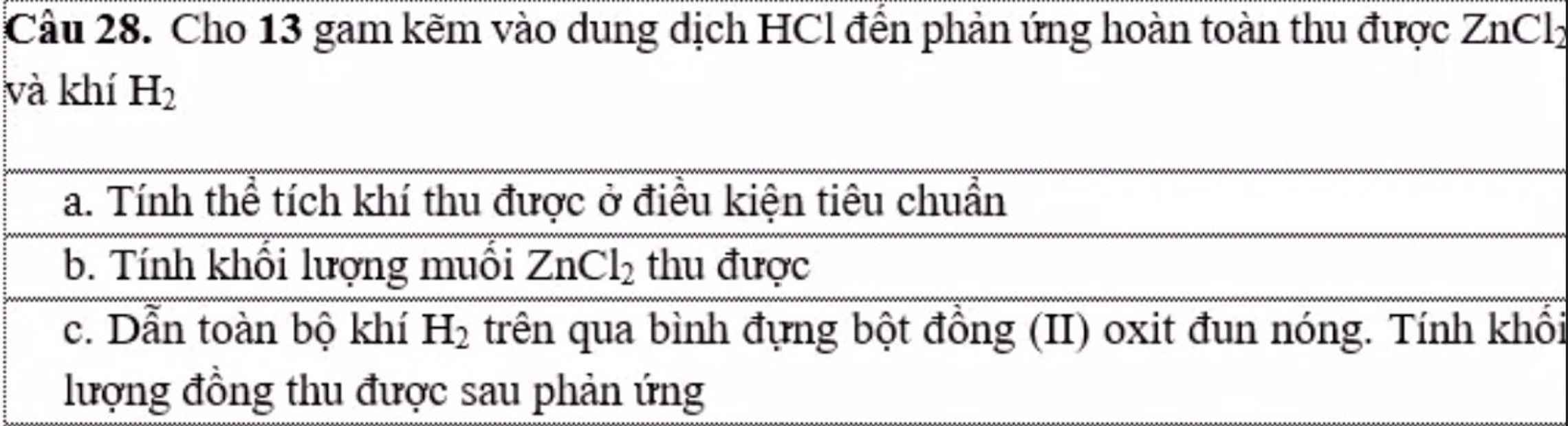
\(a,n_{Zn}=\dfrac{13}{65}=0,2\left(mol\right)\)
PTHH:
\(Zn+2HCl\rightarrow ZnCl_2+H_2\uparrow\)
0,2--------------->0,2------>0,2
\(H_2+CuO\xrightarrow[]{t^o}Cu+H_2O\)
0,2-------------->0,2
\(V_{H_2}=0,2.22,4=4,48\left(l\right)\\ b,m_{ZnCl_2}=0,2.136=27,2\left(g\right)\\ c,m_{Cu}=0,2.64=12,8\left(g\right)\)
\(n_{Zn}=\dfrac{13}{65}=0,2\left(mol\right)\\
pthh:Zn+2HCl\rightarrow ZnCl_2+H_2\)
0,2 0,2 0,2
\(V_{H_2}=0,2.22,4=4,48\left(L\right)\\
m_{ZnCl_2}=136.0,2=27,2\left(g\right)\)
\(pthh:CuO+H_2\underrightarrow{t^o}Cu+H_2O\)
0,2 0,2
\(m_{Cu}=0,2.64=12,8\left(g\right)\)
tìm x,y biết x+y=10 và x/y =1,5 ai giúp câu này vs plss.
\(\dfrac{x}{y}=1,5\Rightarrow x=1,5y\)
\(x+y=10\Rightarrow1,5y+y=10\Rightarrow2,5y=10\Rightarrow y=10:2,5=4\)
\(\Rightarrow x=10-4=6\)
Vậy \(\left\{{}\begin{matrix}x=6\\y=4\end{matrix}\right.\)
lim(3sinn-4cosn+5)/(2n^5+1) giúp mình vs chuẩn bị mình đi hc rr plss
Do \(\left\{{}\begin{matrix}-1\le sinn\le1\\-1\le cosn\le1\end{matrix}\right.\)
\(\Rightarrow-2\le3sinn-4cosn+5\le12\)
\(\Rightarrow\dfrac{-2}{2n^5+1}\le\dfrac{3sinn-4cosn+5}{2n^5+1}\le\dfrac{12}{2n^5+1}\)
Mà \(lim\dfrac{-2}{2n^5+1}=\lim\dfrac{12}{2n^5+1}=0\)
\(\Rightarrow\lim\dfrac{3sinn-4cosn+5}{2n^5+1}=0\)
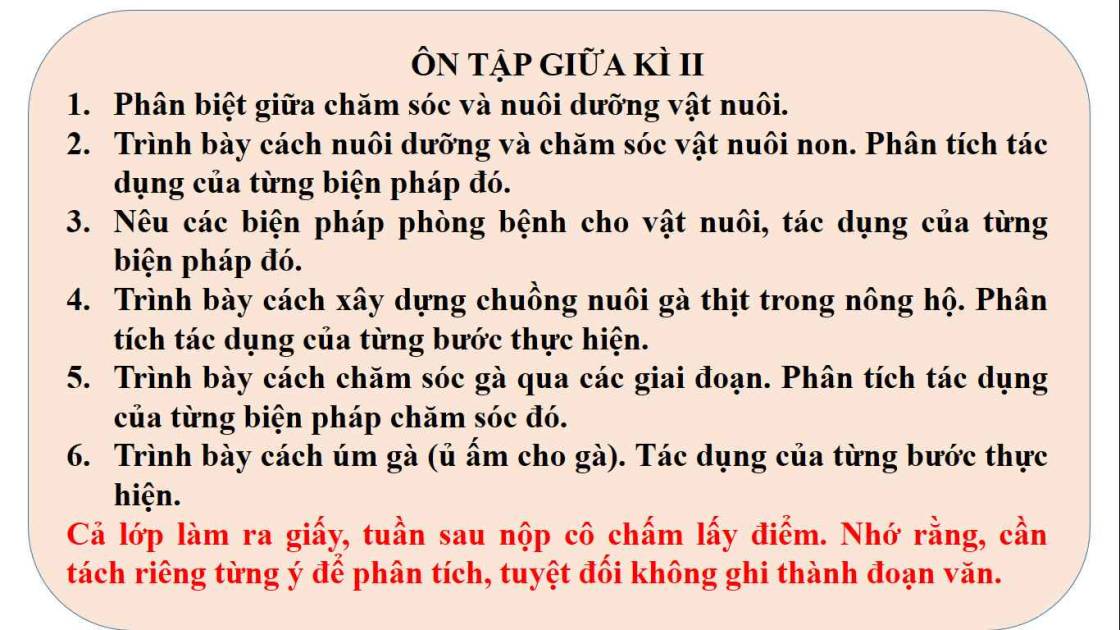
giúp vs ạ plss

Giúp mik với,plss
 giải dùm câu 3 vs plss
giải dùm câu 3 vs plss
giúp em 3 bài này gấp vs ạ!plss
Câu 5:
\(\left\{{}\begin{matrix}x^2+y^2=4\left('\right)\\x-y-xy=2\left(''\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y\right)^2+2xy=4\\x-y-xy=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y\right)^2+2xy=4\left(1\right)\\2\left(x-y\right)-2xy=4\left(2\right)\end{matrix}\right.\)
Lấy \(\left(1\right)+\left(2\right)\) ta được:
\(\left(x-y\right)^2+2\left(x-y\right)=8\)
\(\Leftrightarrow\left(x-y\right)^2+2\left(x-y\right)+1-9=0\)
\(\Leftrightarrow\left(x-y+1\right)^2-9=0\)
\(\Leftrightarrow\left(x-y-2\right)\left(x-y+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-y=2\\x-y=-4\end{matrix}\right.\)
Với \(x-y=2\) Thay vào \(\left(''\right)\) ta được:
\(2-xy=2\Rightarrow xy=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\Rightarrow y=-2\\y=0\Rightarrow x=2\end{matrix}\right.\)
Với \(x-y=4\Rightarrow x=4+y\) Thay vào \(\left('\right)\) ta được:
\(\left(4+y\right)^2+y^2=4\)
\(\Leftrightarrow y^2+8y+16+y^2-4=0\)
\(\Leftrightarrow2y^2+8y+12=0\)
\(\Leftrightarrow y^2+4y+6=0\)
\(\Leftrightarrow\left(y+2\right)^2+2=0\) (phương trình vô nghiệm).
Vậy hệ phương trình đã cho có nghiệm \(\left(x,y\right)\in\left\{\left(2;0\right),\left(0;-2\right)\right\}\)
Câu 6: \(\left\{{}\begin{matrix}2xy+y^2=3\left('\right)\\x^2+5xy=6\left(''\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4xy+2y^2=6\left(1\right)\\x^2+5xy=6\left(2\right)\end{matrix}\right.\)
Lấy \(\left(2\right)-\left(1\right)\) ta được:
\(x^2+xy-2y^2=0\)
\(\Leftrightarrow x^2-y^2+xy-y^2=0\)
\(\Leftrightarrow\left(x-y\right)\left(x+y\right)+y\left(x-y\right)=0\)
\(\Leftrightarrow\left(x-y\right)\left(x+2y\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=y\\x=-2y\end{matrix}\right.\)
Với \(x=y\) Thay vào \(\left('\right)\) ta được:
\(2y.y+y^2=3\)
\(\Leftrightarrow y=\pm1\Rightarrow x=\pm1\).
Với \(x=-2y\) Thay vào \(\left('\right)\) ta được:
\(2.\left(-2y\right).y+y^2=3\)
\(\Leftrightarrow y^2=-1\) (phương trình vô nghiệm)
Vậy hệ phương trình đã cho có nghiệm \(\left(x,y\right)\in\left\{\left(1;1\right),\left(-1;-1\right)\right\}\)
Câu 4: \(Đk:x>-1;y>-\dfrac{1}{2}\)
Đặt \(\left\{{}\begin{matrix}a=\dfrac{1}{\sqrt{x+1}}\left(a>0\right)\\b=\dfrac{1}{\sqrt{2y+1}}\left(b>0\right)\end{matrix}\right.\)
Hệ phương trình đã cho trở thành:
\(\left\{{}\begin{matrix}2a+b=5\\3a+2b=9\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4a+2b=10\\3a+2b=9\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2a+b=5\\a=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=1\\b=3\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{1}{\sqrt{x+1}}=1\\\dfrac{1}{\sqrt{2y+1}}=3\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\sqrt{x+1}=1\\\sqrt{2y+1}=\dfrac{1}{3}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x+1=1\\2y+1=\dfrac{1}{9}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=-\dfrac{4}{9}\end{matrix}\right.\left(nhận\right)\)
Mọi người giải giúp mik với ạ, plss 🥺