Tìm x
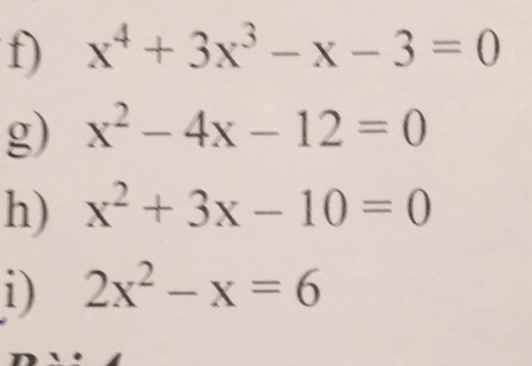
A=\(\dfrac{x}{x-4}+\dfrac{1}{\sqrt{x}-2}+\dfrac{1}{\sqrt{x}+2}\)
a) Rút gọn A ( tìm đkxđ )
b)Tìm A khi x = 36
c)Tìm x để A= -1/3
d) Tìm x để A>0
e)Tìm x thuộc Z để A thuộc Z
ĐKXĐ: \(x\ge0;x\ne4\)
\(A=\dfrac{x}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}+\dfrac{\sqrt{x}+2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}+\dfrac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{x+2\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
b. \(x=36\Rightarrow A=\dfrac{\sqrt{36}}{\sqrt{36}-2}=\dfrac{6}{6-2}=\dfrac{3}{2}\)
c. \(A=-\dfrac{1}{3}\Rightarrow\dfrac{\sqrt{x}}{\sqrt{x}-2}=-\dfrac{1}{3}\Rightarrow3\sqrt{x}=2-\sqrt{x}\)
\(\Rightarrow4\sqrt{x}=2\Rightarrow\sqrt{x}=\dfrac{1}{2}\Rightarrow x=\dfrac{1}{4}\)
d. \(A>0\Rightarrow\dfrac{\sqrt{x}}{\sqrt{x}-2}>0\Rightarrow\sqrt{x}-2>0\Rightarrow x>4\)
e. \(A=\dfrac{\sqrt{x}-2+2}{\sqrt{x}-2}=1+\dfrac{2}{\sqrt{x}-2}\in Z\Rightarrow\sqrt{x}-2=Ư\left(2\right)\)
\(\Rightarrow\sqrt{x}-2=\left\{-2;-1;1;2\right\}\)
\(\Rightarrow\sqrt{x}=\left\{0;1;3;4\right\}\Rightarrow x=\left\{0;1;9;16\right\}\)
a: Ta có: \(A=\dfrac{x}{x-4}+\dfrac{1}{\sqrt{x}-2}+\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{x+\sqrt{x}+2+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
b: Thay x=36 vào A, ta được:
\(A=\dfrac{6}{6-2}=\dfrac{6}{4}=\dfrac{3}{2}\)
c: Để \(A=-\dfrac{1}{3}\) thì \(3\sqrt{x}=-\sqrt{x}+2\)
\(\Leftrightarrow4\sqrt{x}=2\)
hay \(x=\dfrac{1}{4}\)
d: Để A>0 thì \(\sqrt{x}-2>0\)
hay x>4
e: Để A nguyên thì \(\sqrt{x}⋮\sqrt{x}-2\)
\(\Leftrightarrow\sqrt{x}-2\in\left\{-1;1;2;-2\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{1;3;4;0\right\}\)
hay \(x\in\left\{1;9;16;0\right\}\)
a, Cho `0<x<25`
Tìm GTLN:`(80-2x)(50-2x)x`
b, `0<x<2`. Tìm GTLN: `5x(2-x)`
c, `x≥2`. Tìm GTLN: `x + 1/x`
d, Cho `x,y>0, x+y≤1`. TÌm GTNN: `x + y + 1/x + 1/y`
d. Áp dụng BĐT Caushy Schwartz ta có:
\(x+y+\dfrac{1}{x}+\dfrac{1}{y}\le x+y+\dfrac{\left(1+1\right)^2}{x+y}=x+y+\dfrac{4}{x+y}\le1+\dfrac{4}{1}=5\)
-Dấu bằng xảy ra \(\Leftrightarrow x=y=\dfrac{1}{2}\)
c. Bạn kiểm tra lại đề nhé.
b. \(5x\left(2-x\right)=-5x\left(x-2\right)=-5\left(x^2-2x\right)=-5\left(x^2-2x+1-1\right)=-5\left(x-1\right)^2+5\le5\)-Dấu bằng xảy ra \(\Leftrightarrow x=1\)
a.
\(\left(80-2x\right)\left(50-2x\right)x=\dfrac{2}{3}\left(40-x\right)\left(50-2x\right)3x\le\dfrac{2}{3}\left(\dfrac{40-x+50-2x+3x}{3}\right)^3=18000\)
Dấu "=" xảy ra khi \(40-x=50-2x=3x\Leftrightarrow x=10\)
b.
\(5x\left(2-x\right)=5.x\left(2-x\right)\le\dfrac{5}{4}\left(x+2-x\right)^2=5\)
Dấu "=" xảy ra khi \(x=2-x\Rightarrow x=1\)
c.
Biểu thức này chỉ có min, ko có max
d.
\(x+y\le1\Rightarrow-\left(x+y\right)\ge-1\)
\(x+y+\dfrac{1}{x}+\dfrac{1}{y}=\left(4x+\dfrac{1}{x}\right)+\left(4y+\dfrac{1}{y}\right)-3\left(x+y\right)\ge2\sqrt{\dfrac{4x}{x}}+2\sqrt{\dfrac{4y}{y}}-3.1=5\)
Dấu "=" xảy ra khi \(x=y=\dfrac{1}{2}\)
(x-42)-17=127 (tìm x) x_x
23(x+1)=69(tìm x)x_x
2.x+5=120:2(tìm x) x_x
5.x-2=613(tìm x) x_x
(x-42) - 17 = 127
=> x - 42 = 127 + 17 = 144
=> x = 144 + 42 = 186
23(x+1) = 69
=> x + 1 = 69 : 23 = 3
x = 3 - 1 = 2
2x + 5 = 120 : 2 = 60
=> 2x = 60 - 5 = 55
x = 55 : 2 = 27,5
5x - 2 = 613
=> 5x = 613 + 2 = 615
x = 615 : 5 = 123
a)(x-42)-17=127
(x-42)=127+17
(x-42)=144
x=144+42
x=186
b)23(x+1)=69
(x+1)=69:23
(x+1)=3
x=3-1
x=2
c)2.x+5=120:2
2.x+5=60
2.x=60-5
2.x=55
x=55:2
x=27,5
d)5.x-2=613
5.x=613+2
5.x=615
x=615:5
x=123
(x-42)-17=127(tim x)xx
23(x+1)=69(timx)xx
2x+5=120:2(Timx)xx
5x-2=613(timx)xx
` P = ( (3+x)/(3-x) - (3-x)/(3+x) - (4x^2)/( x^2-9) ) . ( (5)/(3-x) - (4x+2)/(3x-x^2) ) `
a) Rút gọn
b) Tính P với `x^2 - 4x + 3 = 0 `
c) Tìm x để P > 0
d) Tìm x thuộc Z để P thuộc Z
e) Tìm x để P = -4
g) Tìm GTNN của P với x thuộc Z
h) Tìm x để P > 4x
a:
Sửa đề: \(P=\left(\dfrac{3+x}{3-x}-\dfrac{3-x}{3+x}-\dfrac{4x^2}{x^2-9}\right):\left(\dfrac{5}{3-x}-\dfrac{4x+2}{3x-x^2}\right)\)\(P=\left(\dfrac{-\left(x+3\right)}{x-3}+\dfrac{x-3}{x+3}-\dfrac{4x^2}{\left(x-3\right)\left(x+3\right)}\right):\dfrac{5x-4x-2}{x\left(3-x\right)}\)
\(=\dfrac{-x^2-6x-9+x^2-6x+9-4x^2}{\left(x-3\right)\left(x+3\right)}:\dfrac{x-2}{x\left(3-x\right)}\)
\(=\dfrac{-4x^2-12x}{\left(x-3\right)\left(x+3\right)}\cdot\dfrac{x\left(3-x\right)}{x-2}\)
\(=\dfrac{-4x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}\cdot\dfrac{-x\left(x-3\right)}{x-2}=\dfrac{4x^2}{x-2}\)
b: x^2-4x+3=0
=>x=1(nhận) hoặc x=3(loại)
Khi x=1 thì \(P=\dfrac{4\cdot1^2}{1-2}=-4\)
c: P>0
=>x-2>0
=>x>2
d: P nguyên
=>4x^2 chia hết cho x-2
=>4x^2-16+16 chia hết cho x-2
=>x-2 thuộc {1;-1;2;-2;4;-4;8;-8;16;-16}
=>x thuộc {1;4;6;-2;10;-6;18;-14}
Cho đa thức f(x) = 2x2 +3x
a) Tìm nghiệm của đa thức f(x)
b) Tìm x để f(x) >/ 0
c) Tìm x để f(x) < 0
d) Tìm x để f(x) = -1
e) Tìm x để f(x) > -1
f) Tìm x để f(x) /< -1
1. tìm GTNN của A= x(x+2)(x+4)(x+6)+8
2. tìm GTLN của B=5+(1-x)(x+2)(x+3)(x+6)3
3.tìm GTNN của C=(x+3)4 + (x-7)4
4. Cho x>0. Tìm GTNN của P=\(\dfrac{4x^2+1}{2x}\)
1.
$x(x+2)(x+4)(x+6)+8$
$=x(x+6)(x+2)(x+4)+8=(x^2+6x)(x^2+6x+8)+8$
$=a(a+8)+8$ (đặt $x^2+6x=a$)
$=a^2+8a+8=(a+4)^2-8=(x^2+6x+4)^2-8\geq -8$
Vậy $A_{\min}=-8$ khi $x^2+6x+4=0\Leftrightarrow x=-3\pm \sqrt{5}$
2.
$B=5+(1-x)(x+2)(x+3)(x+6)=5-(x-1)(x+6)(x+2)(x+3)$
$=5-(x^2+5x-6)(x^2+5x+6)$
$=5-[(x^2+5x)^2-6^2]$
$=41-(x^2+5x)^2\leq 41$
Vậy $B_{\max}=41$. Giá trị này đạt tại $x^2+5x=0\Leftrightarrow x=0$ hoặc $x=-5$
3.
Đặt $x+3=a; 7-x=b$ thì $a+b=10$
$C=a^4+b^4$
Áp dụng BĐT Bunhiacopxky:
$(a^4+b^4)(1+1)\geq (a^2+b^2)^2$
$\Rightarrow C\geq \frac{(a^2+b^2)^2}{2}$
$(a^2+b^2)(1+1)\geq (a+b)^2=100$
$\Rightarrow a^2+b^2\geq 50$
$\Rightarrow C\geq \frac{50^2}{2}=1250$
Vậy $C_{\min}=1250$
Giá trị này đạt tại $a=b=5\Leftrightarrow x=2$
4 .
tìm x thuộc N biết x ⋮ 13 và 20 < x < 70
5 .
a) tìm ước lớn hơn 4 và nhỏ hơn 17 và 32
b) tìm x ϵ N biết ⋮ x và x < 14
c) tìm x thuộc N biết x : 6 ; 30 ⋮ x
4) Ta có: \(x\) ⋮ 13 vậy \(x\in B\left(13\right)\)
\(B\left(13\right)=\left\{0;13;26;39;52;65;78;91\right\}\)
Mà: \(20< x< 70\Rightarrow x\in\left\{26;39;52;65\right\}\)
5)
a) Ta có: \(\text{Ư}\left(32\right)=\left\{1;2;4;8;16;32\right\}\)
Vậy ước lớn hơn 4 và nhỏ hơn 17 của 32 là 8;16
b) Bạn viết lại đề
c) Ta có: x ⋮ 6 và 30 ⋮ x
Vậy x thuộc bội của 6 và ước của 30
Mà: \(Ư\left(30\right)=\left\{1;2;3;5;6;10;15;30\right\}\)
\(B\left(6\right)=\left\{0;6;12;18;24;30;36;42;...\right\}\)
\(\Rightarrow x\in\left\{6;30\right\}\)
Bài 1: Cho 2 đa thức
M(x)=2,5x^2 -0,5x-x^3-1;1/2 N(x)=-x^3+2,5x^2-6+2x
a,Tìm A(x)=M(x) -N(x) .Rồi tìm nghiệm A(x)
b,Tìm đa thức B(x) biết B(x) =M(x)+N(x),tìm bậc của đa thức B(x)
Bài 3:Tìm nghiệm
a,f(x)=x^2-4x+3
b,f(x)=x^2-7x+12
c,f(x)=x^2+2x+1
d,f(x)=x^4+2
Ok help me pls ;-;
Bài 3:
a) Đặt f(x)=0
\(\Leftrightarrow x^2-4x+3=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=3\end{matrix}\right.\)
b) Đặt f(x)=0
\(\Leftrightarrow x^2-7x+12=0\)
\(\Leftrightarrow\left(x-3\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=4\end{matrix}\right.\)
Bài 3:
c) Đặt f(x)=0
\(\Leftrightarrow x^2+2x+1=0\)
\(\Leftrightarrow\left(x+1\right)^2=0\)
\(\Leftrightarrow x+1=0\)
hay x=-1
d) Đặt f(x)=0
\(\Leftrightarrow x^4+2=0\)
\(\Leftrightarrow x^4=-2\)(Vô lý)
Bài 1: Cho 2 đa thức
M(x)=2,5x^2 -0,5x-x^3-1;1/2 N(x)=-x^3+2,5x^2-6+2x
a,Tìm A(x)=M(x) -N(x) .Rồi tìm nghiệm A(x)
b,Tìm đa thức B(x) biết B(x) =M(x)+N(x),tìm bậc của đa thức B(x)
Bài 3:Tìm nghiệm
a,f(x)=x^2-4x+3
b,f(x)=x^2-7x+12
c,f(x)=x^2+2x+1
d,f(x)=x^4+2
Ok help me ;-;
Bài 1: Cho 2 đa thức
M(x)=2,5x^2 -0,5x-x^3-1
1/2 N(x)=-x^3+2,5x^2-6+2x
a,Tìm A(x)=M(x) -N(x) .Rồi tìm nghiệm A(x)
b,Tìm đa thức B(x) biết B(x) =M(x)+N(x),tìm bậc của đa thức B(x)
PLS HELP ME PLS ;-;
a) Ta có: A(x)=M(x)-N(x)
\(=\dfrac{5}{2}x^2-\dfrac{1}{2}x-x^3-1-\left(-2x^3+5x^2-12+4x\right)\)
\(=\dfrac{5}{2}x^2-\dfrac{1}{2}x-x^3-1+2x^3-5x^2+12-4x\)
\(=x^3-\dfrac{5}{2}x^2-\dfrac{9}{2}x+11\)