1 am going to send

2 will get

3 will do

4 will be

5 are going to visit

6 will win

7 am going to take

8 are going

9 will go

10 is going to defeat

11 is going to have

12 will never lie

13 will fly

14 won't tell

15 will like

(a) \(A=\dfrac{3}{x-2}\in Z\)

\(\Rightarrow\left(x-2\right)\inƯ\left(3\right)=\left\{\pm1;\pm3\right\}\)

\(\Rightarrow\left[{}\begin{matrix}x-1=1\\x-1=-1\\x-1=3\\x-1=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=0\\x=4\\x=-2\end{matrix}\right.\)

Vậy: \(x\in\left\{-2;0;2;4\right\}.\)

(b) \(B=-\dfrac{11}{2x-3}\in Z\)

\(\Rightarrow\left(2x-3\right)\inƯ\left(11\right)=\left\{\pm1;\pm3\right\}\)

\(\Rightarrow\left[{}\begin{matrix}2x-3=1\\2x-3=-1\\2x-3=11\\2x-3=-11\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=1\\x=7\\x=-4\end{matrix}\right.\)

Vậy: \(x\in\left\{-4;1;2;7\right\}.\)

(c) \(C=\dfrac{x+3}{x+1}=\dfrac{\left(x+1\right)+2}{x+1}=1+\dfrac{2}{x+1}\in Z\Rightarrow\dfrac{2}{x+1}\in Z\)

\(\Rightarrow\left(x+1\right)\inƯ\left(2\right)=\left\{\pm1;\pm2\right\}\)

\(\Rightarrow\left[{}\begin{matrix}x+1=1\\x+1=-1\\x+1=2\\x+1=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\\x=1\\x=-3\end{matrix}\right.\)

Vậy: \(x\in\left\{-3;-2;0;1\right\}.\)

(d) \(D=\dfrac{2x+10}{x+3}=\dfrac{2\left(x+3\right)+4}{x+3}=2+\dfrac{4}{x+3}\in Z\Rightarrow\dfrac{4}{x+3}\in Z\)

\(\Rightarrow\left(x+3\right)\inƯ\left(4\right)=\left\{\pm1;\pm2\pm4\right\}\)

\(\Rightarrow x\in\left\{-2;-4;-1;-5;1;-7\right\}\)

Câu 1.

Khi mở khóa K:

\(I_m=I_1=0,4A\)

Khi đóng khóa K:

\(I_m=I_1+I_2=0,6\Rightarrow I_2=0,2A\)

\(U_1=0,4\cdot5=2V\)

\(\Rightarrow U_2=U_1=2V\)

\(\Rightarrow U=U_1=U_2=2V\)

\(R_2=\dfrac{U_2}{I_2}=\dfrac{2}{0,2}=10\Omega\)

Bố cục của bài văn biểu cảm gồm 3 phần

gồm 3 phần 

1. English is more interesting than music.

2. Today they are not as happy as they were yesterday.

3. Ha Noi is not as small as Hai Duong.

4. Mai's sister is not as pretty as her.

6. You have got more money than me.

7. Art is not as difficult as French.

8. Nam's father is more careful than him.

9. No one in our town is as rich as Mr Ron.

10. He is the most intelligent in my class.

11. Everest is the highest mountain in the world.

12. Minh is the fattest person in my group.

13. I can't swim as far as Jan.

14B 15C 16A 17C 18B 19C 20B

bạn có thể chụp thẳng đc hong?????

Gọi tam giác ABC vuông tại A, trung tuyến AM, đường cao AH

\(\Rightarrow AM=5\left(cm\right);AH=4\left(cm\right)\)

Ta có AM là trung tuyến ứng với cạnh huyền BC

\(\Rightarrow BC=2AM=10\left(cm\right)\)

Áp dụng HTL tam giác \(AH\cdot BC=AB\cdot AC\Rightarrow AB\cdot AC=40\Rightarrow AB=\dfrac{40}{AC}\\ \dfrac{1}{AH^2}=\dfrac{1}{AB^2}+\dfrac{1}{AC^2}\\ \Rightarrow\dfrac{1}{16}=\dfrac{1}{\dfrac{1600}{AC^2}}+\dfrac{1}{AC^2}\\ \Rightarrow\dfrac{AC^4+1600}{1600AC^2}=\dfrac{100AC^2}{1600AC^2}\Rightarrow AC^4-100AC^2+1600=0\\ \Rightarrow\left(AC^2-80\right)\left(AC^2-20\right)=0\\ \Rightarrow\left[{}\begin{matrix}AC^2=80\\AC^2=20\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}AC=4\sqrt{5}\left(AC>0\right)\\AC=2\sqrt{5}\left(AC>0\right)\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}AB=2\sqrt{5}\\AB=4\sqrt{5}\end{matrix}\right.\)

Vậy với AB là cạnh góc vuông lớn thì \(\left(AB;AC;BC\right)=\left(4\sqrt{5};2\sqrt{5};10\right)\)

Em cần cả hình vẽ lẫn lời giải luôn nha :3

Akai Haruma giúp em với ạ

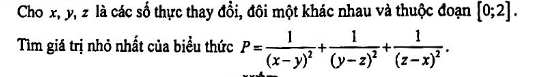
Do vai trò của 3 biến là như nhau, không mất tính tổng quát giả sử \(x>y>z\)

Ta có: \(x-z=\left(x-y\right)+\left(y-z\right)\)

Đặt \(\left\{{}\begin{matrix}x-y=a>0\\y-z=b>0\end{matrix}\right.\)  

Do \(x;z\in\left[0;2\right]\Rightarrow x-z\le2\) hay \(a+b\le2\)

Ta có:

\(P=\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{\left(a+b\right)^2}\ge\dfrac{1}{2}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)^2+\dfrac{1}{\left(a+b\right)^2}\ge\dfrac{1}{2}\left(\dfrac{4}{a+b}\right)^2+\dfrac{1}{\left(a+b\right)^2}\)

\(P\ge\dfrac{9}{\left(a+b\right)^2}\ge\dfrac{9}{2^2}=\dfrac{9}{4}\)

Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}a=b\\a+b=2\\\end{matrix}\right.\) \(\Rightarrow a=b=1\) hay \(\left(x;y;z\right)=\left(0;1;2\right)\) và các hoán vị