tìm gtln \(-x+\sqrt{x}\)

Những câu hỏi liên quan
Cho biểu thức A =\(\left(\frac{x+2}{x\sqrt{x}-1}+\frac{\sqrt{x}}{x+\sqrt{x}+1}+\frac{1}{1-\sqrt{x}}\right):\frac{\sqrt{x}-1}{2}\)
Tìm x để A đạt GTLN, tìm GTLN đó
Bài 1:
A=\(\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}+\dfrac{1}{1-\sqrt{x}}\right):\dfrac{\sqrt{x}-1}{2}\)
a) Tìm tập xác định của biểu thức A
b) Rút gọn biểu thức A
c) Chứng minh rằng A>0 với mọi x≠1
d) Tìm x để A đạt GTLN, tìm GTLN đó
a: ĐKXĐ: \(\left\{{}\begin{matrix}x\ge0\\x\ne1\end{matrix}\right.\)
b: Ta có: \(A=\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}+\dfrac{1}{1-\sqrt{x}}\right):\dfrac{\sqrt{x}-1}{2}\)
\(=\dfrac{x+2+x-\sqrt{x}-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{2}{\sqrt{x}-1}\)
\(=\dfrac{2}{x+\sqrt{x}+1}\)
Đúng 0
Bình luận (0)
c: Ta có: \(x+\sqrt{x}+1>0\forall x\) thỏa mãn ĐKXĐ
\(\Leftrightarrow\dfrac{2}{x+\sqrt{x}+1}>0\forall x\)
Đúng 0
Bình luận (0)
Tìm GTLN của \(\dfrac{\sqrt{x}-1}{x+\sqrt{x}+2}\)
Đặt A=\(\dfrac{\sqrt{x}-1}{x+\sqrt{x}+2}\)\(\Rightarrow Ax+A\sqrt{x}+2A-\sqrt{x}+1=0\)
\(\Leftrightarrow Ax+\sqrt{x}\left(A-1\right)+2A+1=0\)
\(\Delta=\left(A-1\right)^2-4A\left(2A+1\right)=A^2-2A+1-8A^2-4A\)\(=-7A^2-6A+1\ge0\)
\(\Rightarrow-1\le A\le\dfrac{1}{7}\)
Vậy Max A là \(\dfrac{1}{7}\)
Dâu"=" xảy ra \(\Leftrightarrow A=\dfrac{1}{7}\)
\(\Leftrightarrow7\sqrt{x}-7=x+\sqrt{x}+2\)
\(\Leftrightarrow x-6\sqrt{x}+9=0\)
\(\Leftrightarrow\left(\sqrt{x}-3\right)^2=0\Leftrightarrow x=9\)
Đúng 0
Bình luận (0)
Tìm GTLN :
\(M=\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}\)
ĐK: \(x\ge0\)
Mà: \(\left\{{}\begin{matrix}\sqrt{x}\ge0\forall x\left(xđ\right)\\x\sqrt{x}\ge0\forall x\left(xđ\right)\end{matrix}\right.\)
Nên: \(M=\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}\le\dfrac{1}{7}\forall x\left(xđ\right)\)
Dấu "=" xảy ra:
\(\dfrac{\sqrt{x}+1}{x\sqrt{x}+7}=\dfrac{1}{7}\)
\(\Leftrightarrow7\sqrt{x}+7=x\sqrt{x}+7\)
\(\Leftrightarrow x\sqrt{x}-7\sqrt{x}=0\)
\(\Leftrightarrow\sqrt{x}\left(x-7\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=7\end{matrix}\right.\left(tm\right)\)
Vậy: \(M_{max}=\dfrac{1}{7}\) khi \(\left[{}\begin{matrix}x=0\\x=7\end{matrix}\right.\)
Đúng 1
Bình luận (0)
tìm GTLN
\(M=\dfrac{\sqrt{x}+1}{x-\sqrt{x}+7}\)
Tìm GTLN
\(M=\dfrac{\sqrt{x}+1}{x-\sqrt{x}+7}\)
Lời giải:
Áp dụng BĐT Cô-si:
$x+4\geq 4\sqrt{x}$
$\Rightarrow x-\sqrt{x}+7=(x+4)-\sqrt{x}+3\geq 4\sqrt{x}-\sqrt{x}+3$
$=3(\sqrt{x}+1)$
$\Rightarrow M=\frac{\sqrt{x}+1}{x-\sqrt{x}+7}\leq \frac{\sqrt{x}+1}{3(\sqrt{x}+1)}=\frac{1}{3}$
Vậy $M_{\max}=\frac{1}{3}$
Giá trị này xác định tại $x=4$
Đúng 0
Bình luận (0)
Tìm GTNN của:
a)\(\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\)
b)\(\dfrac{x+\sqrt{x}+1}{\sqrt{x}}\)
Tìm GTLN của:
\(\dfrac{1}{\dfrac{\left(\sqrt{x}+1\right)^2}{\sqrt{x}}}\)
1:
a: \(A=\dfrac{\sqrt{x}+1-2}{\sqrt{x}+1}=1-\dfrac{2}{\sqrt{x}+1}\)
căn x+1>=1
=>2/căn x+1<=2
=>-2/căn x+1>=-2
=>A>=-2+1=-1
Dấu = xảy ra khi x=0
b: 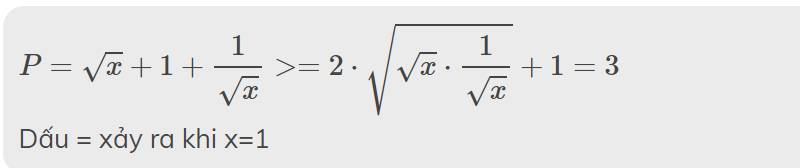
Đúng 0
Bình luận (0)
Bài 1: Tìm GTNN và GTLN của \(A=123+\sqrt{-x^2+6x+5}\)
Bài 2:Tìm GTNN và GTLN của \(A=\sqrt{-x^2+8x-12}-7\)
Bài 3: Tìm GTNN và GTLN của \(A=\sqrt{-x^2-x+4}\)
Tìm GTLN của \(A=\sqrt{x-2}+2\sqrt{x+1}+2019-x\)
Xét \(2A=2\sqrt{x-2}+4\sqrt{x+1}+4038-2x\) (Đk:\(x\ge2\))
\(2A=-\left[\left(x-2\right)-2\sqrt{x-2}+1\right]-\left[\left(x+1\right)-4\sqrt{x+1}+2\right]+4042\)
\(2A=-\left(\sqrt{x-2}-1\right)^2-\left(\sqrt{x+1}-2\right)^2+4042\le4042\)
\(\Leftrightarrow A\le2021\)
\(\Rightarrow Amax=2021\) khi x=3 (tm)Tự đăng câu hỏi xong tự trả lời (T-T)
Đúng 0
Bình luận (0)
tìm gtln,gtnn
E=11+\(\dfrac{6}{\sqrt{x}+3}\)
F=\(\dfrac{\sqrt{x}-2}{\sqrt{x}+3}\)
Lời giải:
Ta thấy: $\sqrt{x}\geq 0$ với mọi $x\geq 0$
$\Leftrightarrow \sqrt{x}+3\geq 3$
$\Rightarrow E=11+\frac{6}{\sqrt{x}+3}\leq 11+\frac{6}{3}=13$
Vậy GTLN của $E$ là $13$. Giá trị này đạt tại $x=0$
$E$ không có giá trị nhỏ nhất.
------------------------
$F=\frac{\sqrt{x}+3-5}{\sqrt{x}+3}=1-\frac{5}{\sqrt{x}+3}$
Ở trên ta chỉ ra được: $\sqrt{x}+3\geq 3$
$\Rightarrow \frac{5}{\sqrt{x}+3}\leq \frac{5}{3}$
$\Rightarrow F=1-\frac{5}{3}\geq 1-\frac{5}{3}=-\frac{2}{3}$
Vậy $F_{\min}=\frac{-2}{3}$ tại $x=0$
Đúng 1
Bình luận (0)















