giải hệ phương trình
{-2x-2y=5
{x-y=6
Những câu hỏi liên quan
Giải các hệ phương trình:
5
+
2
x
+
y
3
-
5
-...
Đọc tiếp
Giải các hệ phương trình: 5 + 2 x + y = 3 - 5 - x + 2 y = 6 - 2 5
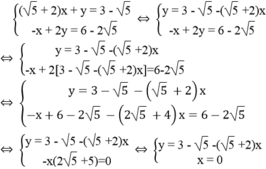
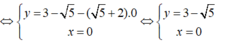
Vậy hệ phương trình có nghiệm duy nhất (x; y) = (0; 3 - 5 ).
Đúng 0
Bình luận (0)
giải hệ phương trình 2x+y=4 x+2y=5
$\begin{cases}2x+y=4\\x+2y=5\end{cases}$
`<=>` $\begin{cases}2x+y=4\\2x+4y=10\end{cases}$
`<=>` $\begin{cases}3y=6\\x=5-3y\end{cases}$
`<=>` $\begin{cases}y=2\\y=1\end{cases}$
Vậy `(x,y)=(2,1)`
Đúng 1
Bình luận (0)
$\begin{cases}2x+y=4\\x+2y=5\end{cases}$
`<=>` $\begin{cases}2x+y=4\\2x+4y=10\end{cases}$
`<=>` $\begin{cases}3y=6\\x=5-2y\end{cases}$
`<=>` $\begin{cases}y=2\\y=1\end{cases}$
Vậy `(x,y)=(2,1)`
Đúng 0
Bình luận (0)
Giải hệ phương trình:\(\hept{\begin{cases}x^2+y^2-2x-2y=6\\x+y-xy=5\end{cases}}\)
Hệ tương đương
\(\hept{\begin{cases}\left(x+y\right)^2-2xy-2\left(x+y\right)=6\\x+y-xy=5\end{cases}}\)
S = x + y, P = xy
=>
\(\hept{\begin{cases}S^2-2P-2S=6\\S-P=5\end{cases}}\)
Thay P = S - 5 vào PT trên
=> S2 - 2(S - 5) - 2S = 6
<=> S2 - 4S + 4 = 0
<=> S = 2
=> P = -3
=> x, y là 1 nghiệm của PT
X2 - 2X - 3 = 0
=>
x = -1, y = 3
Hoặc x = 3, y = -1
Đúng 0
Bình luận (0)
giải các hệ phương trình sau
a.{ x + 3y = -2
{ 5x - 4y = 11
b.{ 3xy = 5
{ 5x + 2y = 23
c.{ 3x +5y = 1
{ 2x - y = -8
d.{ x - 2y + 6 = 0
{ 5x - 3y - 5 = 0
e.{ 2(x + y) + 3(x - y) = 4
{ (x + y) + 2(x - y) = 5
\(a,\Leftrightarrow\left\{{}\begin{matrix}5x+15y=-10\\5x-4y=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}19y=-21\\5x-4y=11\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y=-\dfrac{21}{19}\\5x-4\left(-\dfrac{21}{19}\right)=11\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{25}{19}\\y=-\dfrac{21}{19}\end{matrix}\right.\)
\(c,\Leftrightarrow\left\{{}\begin{matrix}3x+5y=1\\10x-5y=-40\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x+5y=1\\13x=-39\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-3\\y=2\end{matrix}\right.\\ d,\Leftrightarrow\left\{{}\begin{matrix}5x-10y=-30\\5x-3y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5x-3y=5\\-7y=-35\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=5\end{matrix}\right.\\ e,\Leftrightarrow\left\{{}\begin{matrix}2\left(x+y\right)+3\left(x-y\right)=4\\2\left(x+y\right)+4\left(x-y\right)=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-y=6\\2\left(x+y\right)+3\cdot6=4\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x-y=6\\x+y=-7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{1}{2}\\y=-\dfrac{13}{2}\end{matrix}\right.\)
Đúng 3
Bình luận (0)
Giải hệ phương trình:
\(\left\{{}\begin{matrix}x^5=x^4-2x^2y+2\\y^5=y^4-2y^2z+2\\z^5=z^4-2z^2x+2\end{matrix}\right.\)
Giải hệ phương trình \(\hept{\begin{cases}\frac{3+2x-y}{2x-y}-\frac{6}{x+y}=0\\\frac{1-4x+2y}{2x-y}-\frac{1+2x+2y}{x+y}=0\end{cases}}\)
Hint: đặt \(\frac{1}{2x-y}=a;\frac{1}{x+y}=b\)
Giải hệ phương trình sau bằng phương pháp thế
a)
\(\left\{{}\begin{matrix}\sqrt{5}+2)x+y=3-\sqrt{5}\\-x+2y=6-2\sqrt{5}\end{matrix}\right.\)
b)
\(\left\{{}\begin{matrix}5\left(x+2y\right)=3x-1\\2x+4=3\left(x-5y\right)-12\end{matrix}\right.\)
Giải hệ phương trình:
2
x
-
y
5
x
+
y
+
2...
Đọc tiếp
Giải hệ phương trình:
2 x - y = 5 x + y + 2 x + 2 y - 5 = 0
2 x - y = 5 x + y + 2 x + 2 y - 5 = 0
Ta đưa về giải hai hệ phương trình:
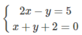
hoặc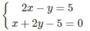 
Giải hệ:
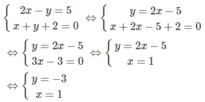
Giải hệ:
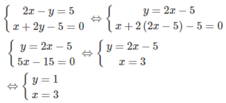
Vậy hệ phương trình đã cho có hai nghiệm
( x 1 ; y 1 ) = (1; -3) và ( x 2 ; y 2 ) = (3; 1)
Đúng 0
Bình luận (0)
giải hệ phương trình
\(\hept{\begin{cases}2\sqrt{2x+y}=3-2x-y\\\sqrt[3]{x+6}+\sqrt{1-y}=4\end{cases}}\)
\(\hept{\begin{cases}\sqrt{2x}+\sqrt{2y}=6\\\sqrt{2x+5}+\sqrt{2y+9}=9\end{cases}}\)