c) Ta có: \(\text{Δ}=\left[-2\left(m+1\right)\right]^2-4\cdot1\cdot\left(2m+1\right)\)

\(=\left(-2m-2\right)^2-4\left(2m+1\right)\)

\(=4m^2+8m+4-8m-4\)

\(=4m^2\ge0\forall m\)

Do đó, phương trình luôn có nghiệm

Áp dụng hệ thức Vi-et, ta có: 

\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2\left(m+1\right)}{1}=2m+2\\x_1\cdot x_2=2m+1\end{matrix}\right.\)

Ta có: \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1-2x_2=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x_2=2m-1\\x_1=2m+2+x_2\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x_2=\dfrac{2m-1}{3}\\x_1=2m+3+\dfrac{2m-1}{3}=\dfrac{8m+8}{3}\end{matrix}\right.\)

Ta có: \(x_1\cdot x_2=2m+1\)

\(\Leftrightarrow\dfrac{2m-1}{3}\cdot\dfrac{8m+8}{3}=2m+1\)

\(\Leftrightarrow\left(2m-1\right)\left(8m+8\right)=9\left(2m+1\right)\)

\(\Leftrightarrow16m^2+16m-8m-8-18m-9=0\)

\(\Leftrightarrow16m^2-10m-17=0\)

\(\text{Δ}=\left(-10\right)^2-4\cdot16\cdot\left(-17\right)=1188\)

Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:

\(\left\{{}\begin{matrix}m_1=\dfrac{10-6\sqrt{33}}{32}\\m_2=\dfrac{10+6\sqrt{33}}{32}\end{matrix}\right.\)

Tiếp tục với bài của bạn Nguyễn Lê Phước Thịnh 

b) Ta có: \(x_1^2+\left(x_1+x_2\right)x_2-2x_1x_2=7\)

              \(\Leftrightarrow x_1^2+x_2^2-x_1x_2=7\)

              \(\Leftrightarrow\left(x_1+x_2\right)^2-3x_1x_2=7\)

\(\Rightarrow\left(2m+1\right)^2- 3\left(2m+1\right)=7\)

\(\Leftrightarrow4m^2-2m-9=0\) \(\Leftrightarrow m=\dfrac{1\pm\sqrt{37}}{4}\)

  Vậy ...

\Delta'=1^2-m=1-mΔ′=12−m=1−m

phương trình có 2 nghiệm <=>\Delta&#x27;\ge0Δ′≥0

<=>1-m\ge01−m≥0

<=>m\le1m≤1

+ Theo vi-et\left\{{}\begin{matrix}x_1+x_2=-2\left(1\right)\\x_1x_2=m\left(2\right)\end{matrix}\right.{x1​+x2​=−2(1)x1​x2​=m(2)​

Theo bai ra: 3x_1+2x_2=1\left(3\right)3x1​+2x2​=1(3)

từ (1)và (3), ta có hệ phương trình\left\{{}\begin{matrix}x_1+x_2=-2\\3x_1+2x_2=1\end{matrix}\right.{x1​+x2​=−23x1​+2x2​=1​ <=>\left\{{}\begin{matrix}x_1=5\\x_2=-7\end{matrix}\right.{x1​=5x2​=−7​. Thay vào (2) : 5.(-7)= m <=> m= -35

b) Theo hệ thức Vi-et ta có:

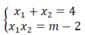
Theo bài ra:

3 x 1  - x 2  = 8

⇔ 3 x 1  - x 2  = 2( x 1  +  x 2 )

⇔  x 1 = 3 x 2

Khi đó:  x 1  +  x 2  = 4 ⇔ 3 x 2  + x 2  = 4 ⇔ 4 x 2  = 4 ⇔  x 2  = 1

⇒  x 1  = 3

⇒  x 1 x 2  = 3 ⇒ m - 2 = 3 ⇔ m = 5

Vậy với m = 5 thì phương trình có 2 nghiệm thỏa mãn yêu cầu đề bài.

PT có 2 nghiệm `<=> \Delta' >0 <=> 2^2-1.(m+1)>0<=> m<3`

Viet: `x_1+x_2=-4`

`x_1 x_2=m+1`

`(x_1)/(x_2)+(x_2)/(x_1)=10/3`

`<=> (x_1^2+x_2^2)/(x_1x_2)=10/3`

`<=> ((x_1+x_2)^2-2x_1x_2)/(x_1x_2)=10/3`

`<=> (4^2-2(m+1))/(m+1)=10/3`

`<=> m=2` (TM)

Vậy `m=2`.

\(pt:x^2-4x+m+1=0\)

\(\Delta=b^2-4ac=\left(-4\right)^2-4.1.\left(m+1\right)\)\(=16-4m-4=12-4m\)

Phương trình có hai nghiệm x₁ ,x₂ :

\(\Leftrightarrow\Delta\ge0\Leftrightarrow12-4m\ge0\Leftrightarrow m\le3\)(1)

Theo hệ thức Viet ta có :

\(\hept{\begin{cases}x_1+x_2=4\\x_1.x_2=m+1\end{cases}}\)

\(x_1^2+x_2^2=12\)

\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1.x_2=12\)

\(\Leftrightarrow16-2m-2=12\Leftrightarrow14-2m=12\Leftrightarrow2m=2\Leftrightarrow m=1\)( TMĐK (1))

Vậy m = 1

bạn đăng tách ra cho mn giúp nhé 

a, Để pt có 2 nghiệm pb 

\(\Delta'=1-m\ge0\Leftrightarrow m\le1\)

Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=-2\left(1\right)\\x_1x_2=m\left(2\right)\end{matrix}\right.\)

\(x_1-3x_2=0\)(3) 

Từ (1) ; (3) ta có hệ \(\left\{{}\begin{matrix}x_1+x_2=-2\\x_1-3x_2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x_1=-2\\x_2=-2-x_1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1=-\dfrac{1}{2}\\x_2=-\dfrac{3}{2}\end{matrix}\right.\)

Thay vào (2) ta được \(m=\left(-\dfrac{1}{2}\right)\left(-\dfrac{3}{2}\right)=\dfrac{3}{4}\)

\(b,\Delta=\left(m+5\right)^2-4\left(-m+6\right)\ge0\Leftrightarrow\left[{}\begin{matrix}m\le-7-4\sqrt{3}\\m\ge-7+4\sqrt{3}\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}x1+x2=m+5\\2x1+3x2=13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x1+2x2=2m+10\\2x1+3x2=13\end{matrix}\right.\)\(\)

\(\Rightarrow x2=13-2m-10=3-2m\Rightarrow x1=m+5-x2=m+5-3+2m=3m+2\)

\(x1x2=6-m\Rightarrow\left(3-2m\right)\left(3m+2\right)=6-m\Leftrightarrow\left[{}\begin{matrix}m=0\left(tm\right)\\m=1\left(tm\right)\end{matrix}\right.\)

\(c,\Delta'=\left(m+1\right)^2-\left(m^2-2m+29\right)\ge0\Leftrightarrow m\ge7\)

\(\Rightarrow\left\{{}\begin{matrix}x1+x2=2m+2\\x1=2x2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x2=\dfrac{2m+2}{3}\\x1=\dfrac{2\left(2m+2\right)}{3}\end{matrix}\right.\)

\(\Rightarrow x1.x2=\dfrac{\left(2m+2\right).2\left(2m+2\right)}{9}=m^2-2m+29\Leftrightarrow\left[{}\begin{matrix}m=11\left(tm\right)\\m=23\left(tm\right)\end{matrix}\right.\)

Áp dụng hệ thức vi-ét:

\(\left\{{}\begin{matrix}x_1+x_2=4\\x_1.x_2=m-1\end{matrix}\right.\)

Ta có:

\(x_1^2+x^2_2=30\)

\(\left(x_1+x_2\right)^2-2x_1.x_2=30\)

\(4^2-2\left(m-1\right)=30\)

\(2m-2=-14\)

\(m=-6\)

Để phương trình đã cho có hai nghiệm \(x_1,x_2\) thì 

\(\Delta'>0\Leftrightarrow2^2-\left(m-1\right)=5-m>0\Leftrightarrow m< 5\)

Khi \(m< 5\) phương trình đã cho có hai nghiệm \(x_1,x_2\).

Theo định lí Viete ta có: 

\(\left\{{}\begin{matrix}x_1+x_2=4\\x_1x_2=m-1\end{matrix}\right.\)

Ta có: 

\(x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2=4^2-2\left(m-1\right)=18-2m=30\)

\(\Leftrightarrow m=-6\) (thỏa mãn) 

\(\Delta=b^2-4ac=4^2-4m\)

Để pt có 2 nghiệm \(x_1,x_2\) thì \(\Delta\ge0\Rightarrow16-4m\ge0\Rightarrow m\le4\)

Theo Vi-ét, ta có :

\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{b}{a}=4\\x_1x_2=-\dfrac{c}{a}=-m\end{matrix}\right.\)

Ta có : \(x_1^2+x_2^2=10\)

\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=10\)

\(\Leftrightarrow4^2-2.\left(-m\right)=10\)

\(\Leftrightarrow16+2m=10\)

\(\Leftrightarrow m=-3\)

\(ac=-1< 0\Rightarrow\) pt luôn có 2 nghiệm pb trái dấu với mọi m

Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1x_2=-1\end{matrix}\right.\)

a.

\(x_1^2+x_2^2-x_1x_2=7\)

\(\Leftrightarrow\left(x_1+x_2\right)^2-3x_1x_2=7\)

\(\Leftrightarrow4m^2+3=7\)

\(\Rightarrow m^2=1\Rightarrow m=\pm1\)

b.

\(x_1-x_2=0\Rightarrow x_1=x_2\Rightarrow x_1x_2=x_2^2\ge0\) (vô lý do \(x_1x_2=-1< 0\) với mọi m)

Vậy ko tồn tại m thỏa mãn yêu cầu