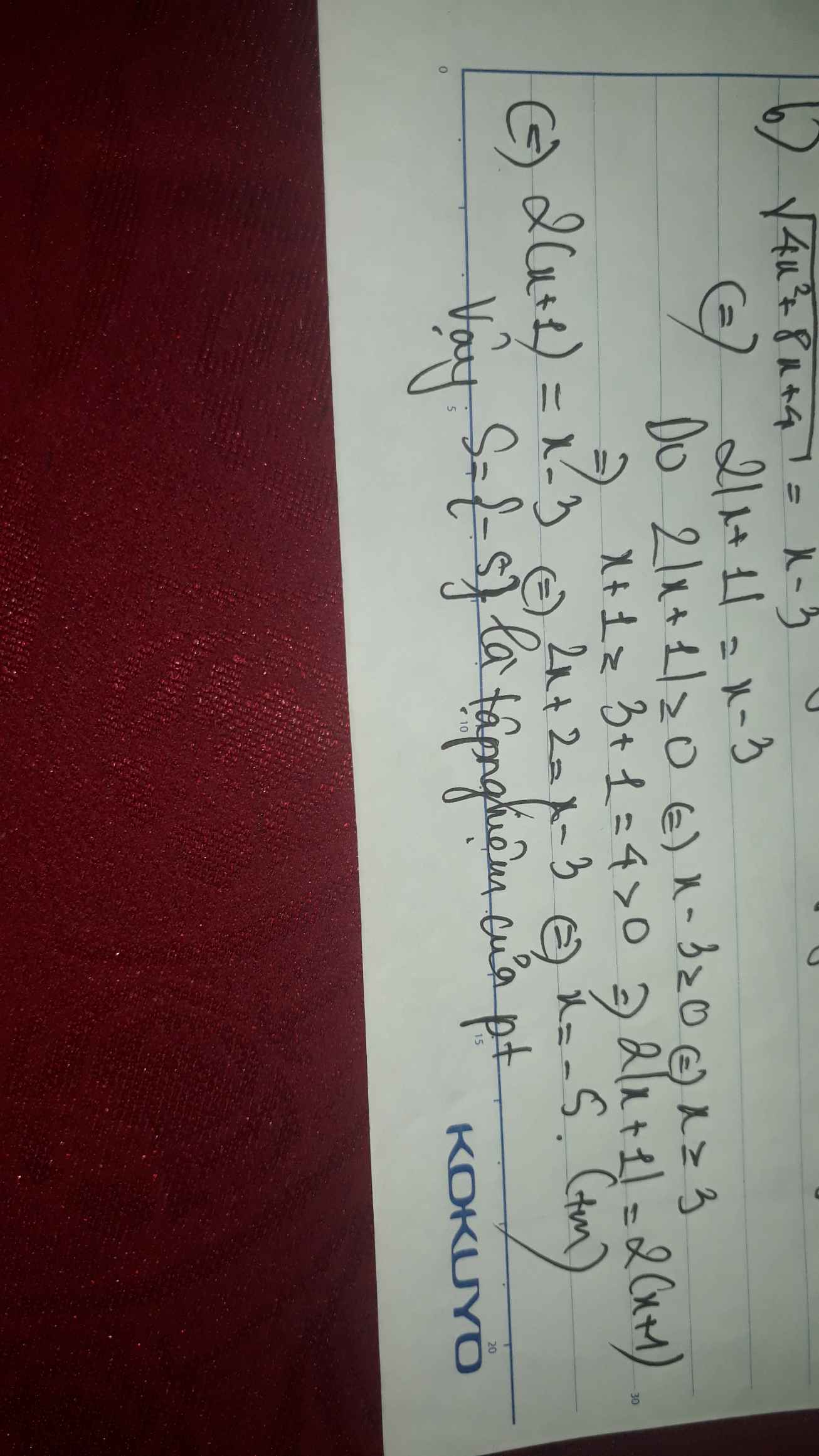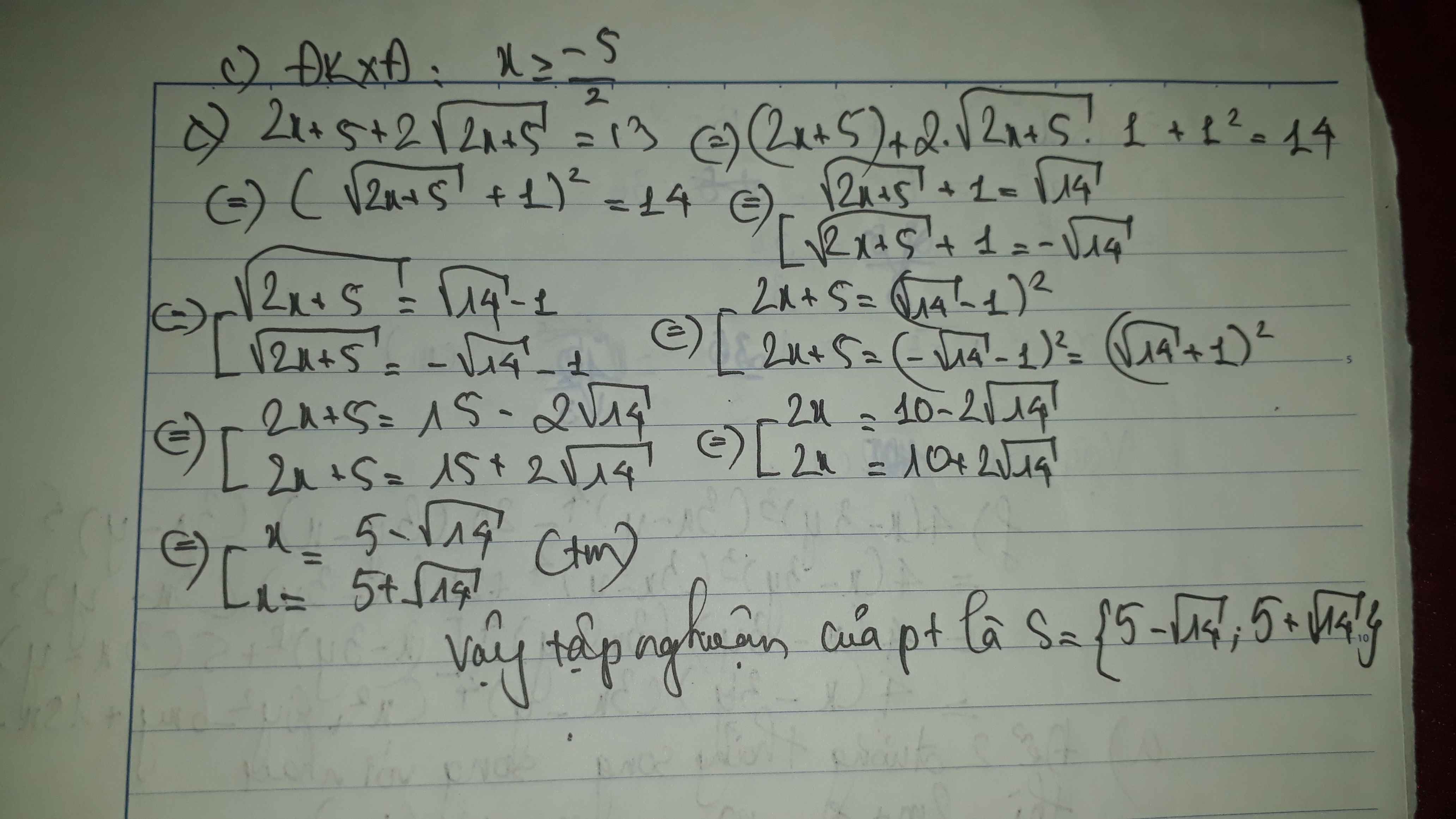giải phương trình: \(\sqrt{2x+3}=\frac{8x^3+4x}{2x+5}\)

Những câu hỏi liên quan
giải phương trình \(\sqrt{2x+3}=\frac{8x^3+4x}{2x+5}\)
https://dehocsinhgioi.com/de-thi-chon-hsg-tinh-lop-9-cap-thcs-vong-tinh-nam-2018-2019-tinh-nghe-an-bang-a-co-dap-an/
bạn tham khảo nhé
thich thì zô ko thích thì zô
Xem thêm câu trả lời
Giải phương trình :
\(\sqrt{\frac{8x^3-1}{2x+3}}+\sqrt{2x+3}=\sqrt{4x^2+2x+1}+\sqrt{2x-1}\)
Giải phương trình và bất phương trình
a) \(3\sqrt{-x^2+x+6}+2\left(2x-1\right)>0\)
b)\(\sqrt{2x^2+8x+5}+\sqrt{2x^2-4x+5}=6\sqrt{x}\)
a.
\(3\sqrt{-x^2+x+6}\ge2\left(1-2x\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}-x^2+x+6\ge0\\1-2x< 0\end{matrix}\right.\\\left\{{}\begin{matrix}1-2x\ge0\\9\left(-x^2+x+6\right)\ge4\left(1-2x\right)^2\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}-2\le x\le3\\x>\dfrac{1}{2}\end{matrix}\right.\\\left\{{}\begin{matrix}x\le\dfrac{1}{2}\\25\left(x^2-x-2\right)\le0\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{1}{2}< x\le3\\\left\{{}\begin{matrix}x\le\dfrac{1}{2}\\-1\le x\le2\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow-1\le x\le3\)
Đúng 0
Bình luận (0)
b.
ĐKXĐ: \(x\ge0\)
\(\Leftrightarrow\sqrt{2x^2+8x+5}-4\sqrt{x}+\sqrt{2x^2-4x+5}-2\sqrt{x}=0\)
\(\Leftrightarrow\dfrac{2x^2+8x+5-16x}{\sqrt{2x^2+8x+5}+4\sqrt{x}}+\dfrac{2x^2-4x+5-4x}{\sqrt{2x^2-4x+5}+2\sqrt{x}}=0\)
\(\Leftrightarrow\dfrac{2x^2-8x+5}{\sqrt{2x^2+8x+5}+4\sqrt{x}}+\dfrac{2x^2-8x+5}{\sqrt{2x^2-4x+5}+2\sqrt{x}}=0\)
\(\Leftrightarrow\left(2x^2-8x+5\right)\left(\dfrac{1}{\sqrt{2x^2+8x+5}+4\sqrt{x}}+\dfrac{1}{\sqrt{2x^2-4x+5}+2\sqrt{x}}\right)=0\)
\(\Leftrightarrow2x^2-8x+5=0\)
\(\Leftrightarrow x=\dfrac{4\pm\sqrt{6}}{2}\)
Đúng 0
Bình luận (0)
Câu b còn 1 cách giải nữa:
Với \(x=0\) không phải nghiệm
Với \(x>0\) , chia 2 vế cho \(\sqrt{x}\) ta được:
\(\sqrt{2x+8+\dfrac{5}{x}}+\sqrt{2x-4+\dfrac{5}{x}}=6\)
Đặt \(\sqrt{2x-4+\dfrac{5}{x}}=t>0\Leftrightarrow2x+8+\dfrac{5}{x}=t^2+12\)
Phương trình trở thành:
\(\sqrt{t^2+12}+t=6\)
\(\Leftrightarrow\sqrt{t^2+12}=6-t\)
\(\Leftrightarrow\left\{{}\begin{matrix}6-t\ge0\\t^2+12=\left(6-t\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}t\le6\\12t=24\end{matrix}\right.\)
\(\Rightarrow t=2\)
\(\Rightarrow\sqrt{2x-4+\dfrac{5}{x}}=2\)
\(\Leftrightarrow2x-4+\dfrac{5}{x}=4\)
\(\Rightarrow2x^2-8x+5=0\)
\(\Leftrightarrow...\)
Đúng 0
Bình luận (0)
giải phương trình
a) x - \(\sqrt{x-1}\) -3 = 0
b)\(\sqrt{4x^2+8x+4}\) = x - 3
c) 2x + 5 +\(2\sqrt{2x+5}\) = 13
Xem thêm câu trả lời
Giải phương trình: \(\sqrt{2x+3}=\frac{8x^3+4x}{2x+5}\)
ĐKXĐ: ...
\(\Leftrightarrow\left(2x+5\right)\sqrt{2x+3}=8x^3+4x\)
\(\Leftrightarrow\left(2x+3\right)\sqrt{2x+3}+2\left(\sqrt{2x+3}\right)=8x^3+4x\)
Đặt \(\left\{{}\begin{matrix}\sqrt{2x+3}=a\\2x=b\end{matrix}\right.\)
\(\Rightarrow a^3+2a=b^3+2b\)
\(\Leftrightarrow\left(a-b\right)\left(a^2+ab+b^2+2\right)=0\)
\(\Leftrightarrow a=b\Leftrightarrow2x=\sqrt{2x+3}\) (\(x\ge0\))
\(\Leftrightarrow4x^2-2x-3=0\)
Giải phương trình:
\(a,3\sqrt{x}+8=9x+\frac{1}{x}+\frac{1}{\sqrt{x}}\)
\(b,\frac{4x^2+8x+1}{2x+1}=5\sqrt{x}\)
giải phương trình
\(\sqrt{x-1}+\sqrt{x+3}+2\sqrt{x^3-4x^2+8x-5}=2x\)
giải phương trình :
a, \(\sqrt{x-3}+\sqrt[3]{x^2+1}+x^2+x-2=0\)
b,\(4x^2+\sqrt{2x+3}=8x+1\)
c, \(2x^2-6x+10-5\left(x-2\right)\sqrt{x+1=0}\)
a.
ĐKXĐ: \(x\ge3\)
(Tốt nhất bạn kiểm tra lại đề cái căn đầu tiên của \(\sqrt{x-3}\) là căn bậc 2 hay căn bậc 3). Vì nhìn ĐKXĐ thì thấy căn bậc 2 là không hợp lý rồi đó
Pt tương đương:
\(\sqrt{x-3}+\sqrt[3]{x^2+1}+\left(x+1\right)\left(x-2\right)=0\)
Do \(x\ge3\Rightarrow x-2>0\Rightarrow\left(x+1\right)\left(x-2\right)>0\)
\(\Rightarrow\sqrt{x-3}+\sqrt[3]{x^2+1}+\left(x+1\right)\left(x-2\right)>0\)
Pt vô nghiệm
Đúng 0
Bình luận (0)
b.
ĐKXĐ: \(x\ge-\dfrac{3}{2}\)
Pt: \(2x+3-\sqrt{2x+3}-\left(4x^2-6x+2\right)=0\)
Đặt \(\sqrt{2x+3}=t\ge0\) ta được:
\(t^2-t-\left(4x^2-6x+2\right)=0\)
\(\Delta=1+4\left(4x^2-6x+2\right)=\left(4x-3\right)^2\)
\(\Rightarrow\left[{}\begin{matrix}t_1=\dfrac{1+4x-3}{2}=2x-1\\t_2=\dfrac{1-4x+3}{2}=2-2x\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{2x+3}=2x-1\left(x\ge\dfrac{1}{2}\right)\\\sqrt{2x+3}=2-2x\left(x\le1\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+3=4x^2-4x+1\left(x\ge\dfrac{1}{2}\right)\\2x+3=4x^2-8x+4\left(x\le1\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3+\sqrt{17}}{4}\\x=\dfrac{5-\sqrt{21}}{4}\end{matrix}\right.\)
Đúng 0
Bình luận (0)
c.
ĐKXĐ: \(x\ge-1\)
\(\Leftrightarrow2\left(x^2-4x+4\right)+2\left(x+1\right)-5\left(x-2\right)\sqrt{x+1}=0\)
\(\Leftrightarrow2\left(x-2\right)^2-5\left(x-2\right)\sqrt{x+1}+2\left(x+1\right)=0\)
Đặt \(\left\{{}\begin{matrix}x-2=a\\\sqrt{x+1}=b\end{matrix}\right.\) ta được:
\(2a^2-5ab+2b^2=0\)
\(\Leftrightarrow\left(a-2b\right)\left(2a-b\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}a=2b\\2a=b\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2\sqrt{x+1}=x-2\left(x\ge2\right)\\\sqrt{x+1}=2x-4\left(x\ge2\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}4x+4=x^2-4x+4\\x+1=4x^2-16x+16\end{matrix}\right.\) (\(x\ge2\))
\(\Leftrightarrow\left[{}\begin{matrix}x=8\\x=3\end{matrix}\right.\) (đã loại nghiệm)
Đúng 0
Bình luận (0)
Giải phương trình:
\(\sqrt{-x^2+4x-2}+\sqrt{-2x^2+8x-5}=\sqrt{2}+\sqrt{3}\)
\(vt=\sqrt{-\left(x-2\right)^2+2}+\sqrt{-2\left(x-2\right)^2+3}\)
=>\(VT=< \sqrt{2}+\sqrt{3}\)
xảy ra dấu = khi và chỉ khi x=2
Đúng 0
Bình luận (0)
Xem thêm câu trả lời