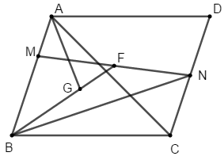
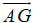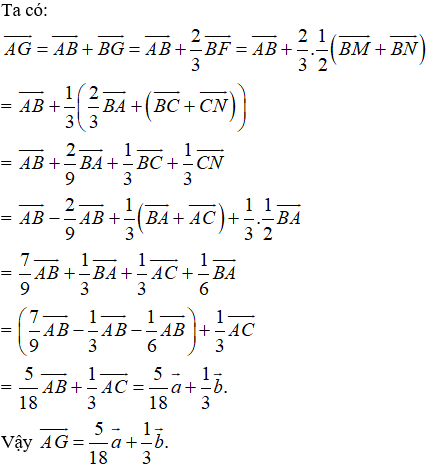1. Cho hbh ABCD. Đặt vecto AB=a, AD=b. Gọi I là trung điểm của CD, G là trọng tâm của tam giác BCI. Phân tích các vecto BI, CG theo vecto a,b
2. Cho tam giác ABC có trọng tâm G. Gọi D là điểm đối xứng của A qua B và E là điểm trên đoạn AC sao cho AE =2/5 AC
a) phân tích vecto DE, DG theo vecto AB và AC
b) cmr D,G,E thẳng hàng
c) xét K là điểm thỏa vecto KA + KB + 3KC = 2KD. CMR KG//CD