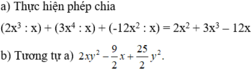ptđttnt: 2x3-12x2+18x

Những câu hỏi liên quan
Phân tích đa thúc thành nhân tử : 2x3 -12x2 + 18x
\(2x^3-12x^2+18x=2x\left(x^2-6x+9\right)=2x\left(x-3\right)^2\)
Đúng 0
Bình luận (0)
\(=2x\left(x^2-6x+9\right)=2x\left(x-3\right)^2\)
Đúng 0
Bình luận (0)
2x3- 12x2 + 18x = 2x (x2 - 6x + 9 ) = 2x ( x - 3 )2
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
Câu 1
Rút gọn các biểu thức sau:
a. 2x(3x + 2) - 3x(2x + 3)
b. (x + 2)3 + (x - 3)2 - x2(x + 5)
c. (3x3 - 4x2 + 6x) : 3x
Câu 2
Phân tích đa thức sau thành nhân tử: 2x3 - 12x2 + 18x
Câu 3
Tìm x, biết: 3x(x - 5) - x2 + 25 0
Câu 4 Cho hình bình hành ABCD (AB AD). Gọi E và K lần lượt là trung điểm của CD và AB. BD cắt AE, AC, CK lần lượt tại N, O và I. Chứng minh rằng:
a. Tứ giắc AECK là hình bình hành.
b. Ba điểm E, O, K thẳng hàng.
c. DN NI IB
d. AE...
Đọc tiếp
Câu 1
Rút gọn các biểu thức sau:
a. 2x(3x + 2) - 3x(2x + 3)
b. (x + 2)3 + (x - 3)2 - x2(x + 5)
c. (3x3 - 4x2 + 6x) : 3x
Câu 2
Phân tích đa thức sau thành nhân tử: 2x3 - 12x2 + 18x
Câu 3
Tìm x, biết: 3x(x - 5) - x2 + 25 = 0
Câu 4 Cho hình bình hành ABCD (AB > AD). Gọi E và K lần lượt là trung điểm của CD và AB. BD cắt AE, AC, CK lần lượt tại N, O và I. Chứng minh rằng:
a. Tứ giắc AECK là hình bình hành.
b. Ba điểm E, O, K thẳng hàng.
c. DN = NI = IB
d. AE = 3KI
Câu 5 Cho x, y là hai số thực tùy ý, tìm giá trị nhỏ nhất của biểu thức sau:
P = x2 + 5y2 + 4xy + 6x + 16y + 32
Câu 1:
a) 2x(3x+2) - 3x(2x+3) = 6x^2+4x - 6x^2-9x = -5x
b) \(\left(x+2\right)^3+\left(x-3\right)^2-x^2\left(x+5\right)\)
\(=x^3+6x^2+12x+8+x^2-6x+9-x^3-5x^2\)
\(=2x^2+6x+17\)
c) \(\left(3x^3-4x^2+6x\right)\div\left(3x\right)=x^2-\dfrac{4}{3}x+2\)
Đúng 2
Bình luận (0)
Câu 2:
\(2x^3-12x^2+18x=2x\left(x^2-6x+9\right)=2x\left(x^2-2.x.3+3^2\right)=2x\left(x-3\right)^2\)
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
PTĐTTNT:
\(x^2-3xy+6y-18x\)
2x-x2=2
x3+15x2+75x+125=0
x3+48x=12x2+64
\(2x-x^2=2\\ \Leftrightarrow x^2-2x+2=0\\ \Leftrightarrow\left(x^2-2x+1\right)+1=0\\ \Leftrightarrow\left(x-1\right)^2+1=0\\ Mà:\left(x-1\right)^2\ge0\forall x\in R\\ \Rightarrow\left(x-1\right)^2+1\ge1\forall x\in R\\ Vậy:Pt.vô.nghiệm\\ x^3+15x^2+75x+125=0\\ x^3+3.x^2.5+3.x.5^2+5^3=0\\ \left(x+5\right)^3=0\\ \Leftrightarrow x+5=0\\ \Leftrightarrow x=-5\\ x^3+48x=12x^2+64\\ \Leftrightarrow x^3-12x^2+48x-64=0\\ \Leftrightarrow x^3-3.x^2.4+3.x.4^2-4^2=0\\ \Leftrightarrow\left(x-4\right)^3=0\\ \Leftrightarrow x-4=0\\ \Leftrightarrow x=4\)
Đúng 4
Bình luận (0)
Làm tính chia:a)
(
2
x
3
+
3
x
4
-
12
x
2
)
: x;b)
(
4
x
2
y
3
-
9
x
2
y
2
+...
Đọc tiếp
Làm tính chia:
a) ( 2 x 3 + 3 x 4 - 12 x 2 ) : x;
b) ( 4 x 2 y 3 - 9 x 2 y 2 + 25 xy 4 ) : 2 xy 2 .
Tìm nghiệm đa thức:
a) f(x) = x3 - 2x -4;
b) f(x) = 2x3 -12x2+ 17x - 2
a: x^3-2x-4=0
=>x^3-2x^2+2x^2-4x+2x-4=0
=>(x-2)(x^2+2x+2)=0
=>x-2=0
=>x=2
b: 2x^3-12x^2+17x-2=0
=>2x^3-4x^2-8x^2+16x+x-2=0
=>(x-2)(2x^2-4x+1)=0
=>x=2; \(x=\dfrac{4\pm\sqrt{14}}{2}\)
Đúng 0
Bình luận (0)
PTĐTTNT:
\(x^8+x+1\)
b)\(\left(x^2+2x\right)^2+9x^2+18x+20\)
\(x^8+x+1\)
\(=\left(x^8+x^7+x^6\right)-\left(x^7+x^6+x^5\right)+\left(x^5+x^4+x^3\right)-\left(x^4+x^3+x^2\right)+\left(x^2+x+1\right)\)
\(=x^6\left(x^2+x+1\right)-x^5\left(x^2+x+1\right)+x^3\left(x^2+x+1\right)-x^2\left(x^2+x+1\right)+\left(x^2+x+1\right)\)
\(=\left(x^2+x+1\right)\left(x^6-x^5+x^3-x^2+1\right)\)
Chúc bạn học tốt!!!
Đúng 0
Bình luận (0)
Phân tích đa thức thành nhân tử
1) 2x3–x2+5x+3
2) 27x3−27x2+18x–427x3−27x2+18x–4
3) x2+2xy+y2−x−y–12x2+2xy+y2−x−y–12
4) (x+2)(x+3)(x+4)(x+5)–24(x+2)(x+3)(x+4)(x+5)–24
5) 4x4−32x2+14x4−32x2+1
6) 3(x4+x2+1)−(x2+x+1)23(x4+x2+1)−(x2+x+1)2
7) 64x4+y4
tìm nghiệm của B(x)
B(x)=2x3 -18x
\(B\left(x\right)=2x^3-18x=0\)
\(\Leftrightarrow2x\left(x^2-9\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=0\\x^2-9=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\pm3\end{matrix}\right.\)
Đúng 3
Bình luận (0)
Xét B(x)=0
⇒ 2x3- 18x = 0
⇒2x (x2 - 9 ) = 0
⇒\(\left\{{}\begin{matrix}2x=0\\x^2-9=0\end{matrix}\right.\)
⇒\(\left\{{}\begin{matrix}x=0:2\\x^2=0+9\end{matrix}\right.\)
⇒\(\left\{{}\begin{matrix}x=0\\x^2=9\end{matrix}\right.\)
⇒\(\left\{{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)
Đúng 0
Bình luận (0)