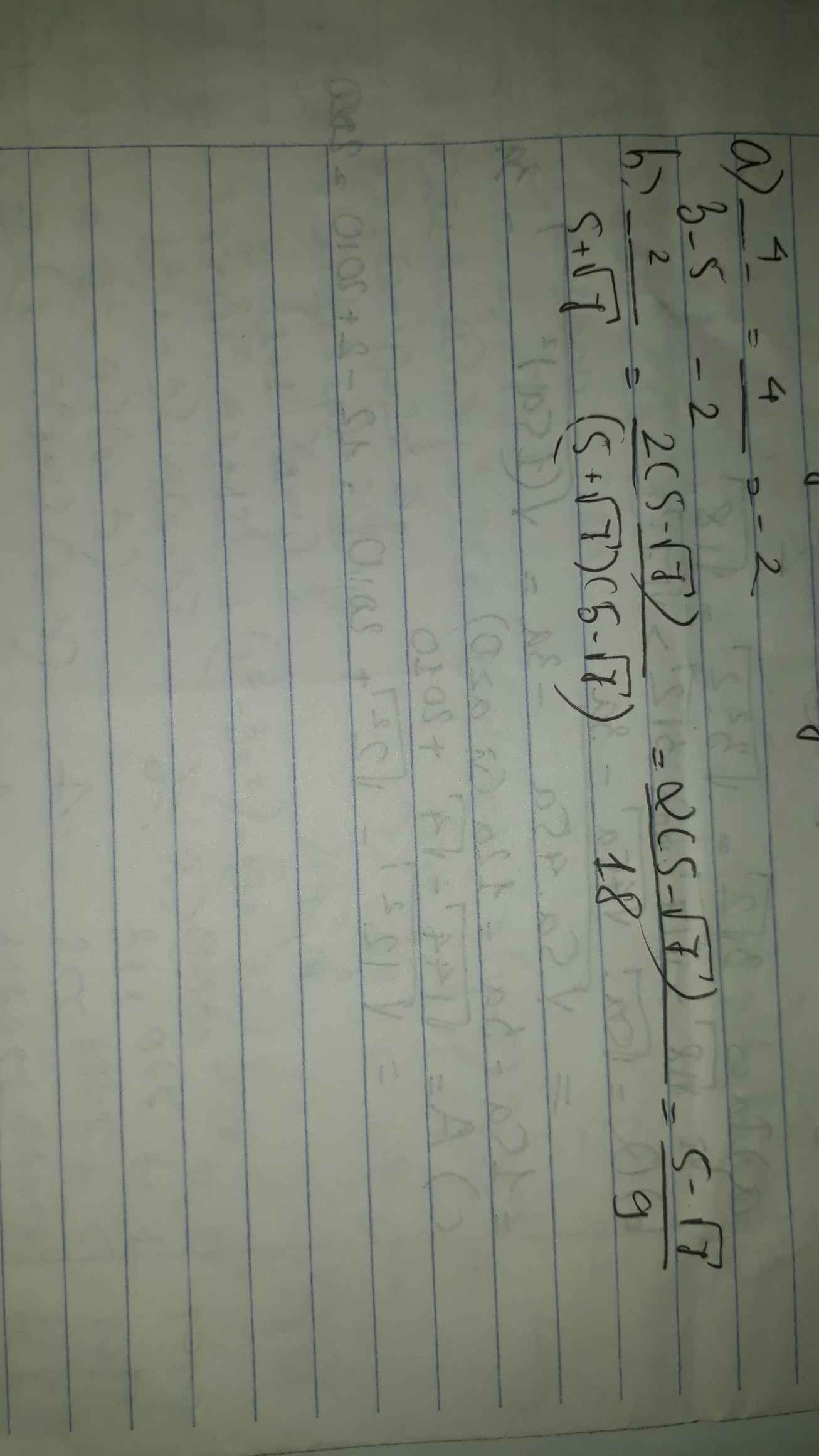\(\dfrac{\sqrt{5}-\sqrt{3}}{\sqrt{2}}=\dfrac{\sqrt{2}\left(\sqrt{5}-\sqrt{3}\right)}{\sqrt{2}.\sqrt{2}}=\dfrac{\sqrt{10}-\sqrt{6}}{2}\)

\(\dfrac{1}{\sqrt{3}+\sqrt{2}+1}=\dfrac{\sqrt{3}-\sqrt{2}-1}{\left(\sqrt{3}+\sqrt{2}+1\right)\left(\sqrt{3}-\sqrt{2}-1\right)}\)

\(=\dfrac{\sqrt{3}-\sqrt{2}-1}{3-\left(\sqrt{2}+1\right)^2}=\dfrac{\sqrt{3}-\sqrt{2}-1}{-2\sqrt{2}}=\dfrac{\left(\sqrt{3}-\sqrt{2}-1\right)\sqrt{2}}{-2\sqrt{2}.\sqrt{2}}=\dfrac{\sqrt{6}-2-\sqrt{2}}{-4}\)

\(=\dfrac{2+\sqrt{2}-\sqrt{6}}{4}\)

\(\dfrac{\sqrt{5}-\sqrt{3}}{\sqrt{2}}=\dfrac{\sqrt{10}-\sqrt{6}}{2}\)

\(\dfrac{1}{\sqrt{3}+\sqrt{2}+1}=\dfrac{2+\sqrt{2}-\sqrt{6}}{4}\)

1) Ta có: \(3\sqrt{12}+\dfrac{1}{2}\sqrt{48}-\sqrt{27}\)

\(=3\cdot2\sqrt{3}+\dfrac{1}{2}\cdot4\sqrt{3}-3\sqrt{3}\)

\(=6\sqrt{3}+2\sqrt{3}-3\sqrt{3}\)

\(=5\sqrt{3}\)

2) Ta có: \(\dfrac{2}{\sqrt{3}-5}\)

\(=\dfrac{2\left(\sqrt{3}+5\right)}{\left(\sqrt{3}-5\right)\left(\sqrt{3}+5\right)}\)

\(=\dfrac{2\left(\sqrt{3}+5\right)}{3-25}\)

\(=\dfrac{-2\left(\sqrt{3}+5\right)}{22}\)

\(=\dfrac{-\sqrt{3}-5}{11}\)

3) Ta có: \(\sqrt{\dfrac{2}{5}}\)

\(=\dfrac{\sqrt{2}}{\sqrt{5}}\)

\(=\dfrac{\sqrt{2}\cdot\sqrt{5}}{5}\)

\(=\dfrac{\sqrt{10}}{5}\)

ĐS:

Bài 1:
a.

\(\frac{1}{2\sqrt{2}-3\sqrt{3}}=\frac{2\sqrt{2}+3\sqrt{3}}{(2\sqrt{2}-3\sqrt{3})(2\sqrt{2}+3\sqrt{3})}=\frac{2\sqrt{2}+3\sqrt{3}}{(2\sqrt{2})^2-(3\sqrt{3})^2}=\frac{2\sqrt{2}+3\sqrt{3}}{-19}\)

b.

\(=\sqrt{\frac{(3-\sqrt{5})^2}{(3-\sqrt{5})(3+\sqrt{5})}}=\sqrt{\frac{(3-\sqrt{5})^2}{3^2-5}}=\sqrt{\frac{(3-\sqrt{5})^2}{4}}=\sqrt{(\frac{3-\sqrt{5}}{2})^2}=|\frac{3-\sqrt{5}}{2}|=\frac{3-\sqrt{5}}{2}\)

Bài 2.

a. 

\(=\frac{\sqrt{8}(\sqrt{5}+\sqrt{3})}{(\sqrt{5}-\sqrt{3})(\sqrt{5}+\sqrt{3})}=\frac{2\sqrt{2}(\sqrt{5}+\sqrt{3})}{5-3}=\sqrt{2}(\sqrt{5}+\sqrt{3})=\sqrt{10}+\sqrt{6}\)

b.

\(=\sqrt{\frac{(2-\sqrt{3})^2}{(2-\sqrt{3})(2+\sqrt{3})}}=\sqrt{\frac{(2-\sqrt{3})^2}{2^2-3}}=\sqrt{(2-\sqrt{3})^2}=|2-\sqrt{3}|=2-\sqrt{3}\)

Bài 3:

a.

\(M=\left[\frac{15(\sqrt{6}-1)}{(\sqrt{6}+1)(\sqrt{6}-1)}+\frac{4(\sqrt{6}+2)}{(\sqrt{6}-2)(\sqrt{6}+2)}-\frac{12(3+\sqrt{6})}{(3-\sqrt{6})(3+\sqrt{6})}\right](\sqrt{6}+11)\)

\(=\left[\frac{15(\sqrt{6}-1)}{6-1}+\frac{4(\sqrt{6}+2)}{6-2^2}-\frac{12(3+\sqrt{6})}{3^2-6}\right](\sqrt{6}+11)\)

\(=[3(\sqrt{6}-1)+2(\sqrt{6}+2)-4(3+\sqrt{6})](\sqrt{6}+11)=(\sqrt{6}-11)(\sqrt{6}+11)=6-11^2=-115\)

b.

\(N=\left[1-\frac{\sqrt{5}(\sqrt{5}+1)}{\sqrt{5}+1}\right].\left[\frac{\sqrt{5}(\sqrt{5}-1)}{1-\sqrt{5}}-1\right]\)

\(=(1-\sqrt{5})(-\sqrt{5}-1)=(\sqrt{5}-1)(\sqrt{5}+1)=5-1=4\)

\(\dfrac{1}{\sqrt{2}}=\dfrac{\sqrt{2}}{2}\)

\(\dfrac{2+\sqrt{3}}{2-\sqrt{3}}=\left(2+\sqrt{3}\right)^2=7+4\sqrt{3}\)

\(\dfrac{1}{\sqrt[3]{16}+\sqrt[3]{12}+\sqrt[3]{9}}=\dfrac{1}{\left(\sqrt[3]{4}\right)^2+\sqrt[3]{4}.\sqrt[3]{3}+\left(\sqrt[3]{3}\right)^2}\)

\(=\dfrac{\left(\sqrt[3]{4}-\sqrt[3]{3}\right)}{\left(\sqrt[3]{4}-\sqrt[3]{3}\right)\left(\sqrt[3]{4}\right)^2+\sqrt[3]{4}.\sqrt[3]{3}+\left(\sqrt[3]{3}\right)^2}\)

\(=\dfrac{\sqrt[3]{4}-\sqrt[3]{3}}{\left(\sqrt[3]{4}\right)^3-\left(\sqrt[3]{3}\right)^3}=\dfrac{\sqrt[3]{4}-\sqrt[3]{3}}{4-3}=\sqrt[3]{4}-\sqrt[3]{3}\)

33+1=3(3−1)(3+1)(3−1)=33−3.1(3)2−12" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">33+1=3(3−1)(3+1)(3−1)=33−3.1(3)2−12

33−33−1=33−32" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">33−33−1=33−32.

23−1=2(3+1)(3−1)(3+1)=2(3+1)(3)2−12" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">23−1=2(3+1)(3−1)(3+1)=2(3+1)(3)2−12

2(3+1)3−1=2(3+1)2=3+1" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">2(3+1)3−1=2(3+1)2=3+1.

2+32−3=(2+3).(2+3)(2−3)(2+3)=(2+3)222−(3)2" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">2+32−3=(2+3).(2+3)(2−3)(2+3)=(2+3)222−(3)2

22+2.2.3+(3)24−3" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">22+2.2.3+(3)24−3

7+431=7+43" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">7+431=7+43.

b3+b=b(3−b)(3+b)(3−b)" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">b3+b=b(3−b)(3+b)(3−b)

b(3−b)32−(b)2=b(3−b)9−b;(b≠9)" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">b(3−b)32−(b)2=b(3−b)9−b;(b≠9).

p2p−1=p(2p+1)(2p−1)(2p+1)" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">p2p−1=p(2p+1)(2p−1)(2p+1)

p(2p+1)(2p)2−12=p(2p+1)4p−1" role="presentation" style="border:0px; direction:ltr; display:inline-block; float:none; font-size:19.36px; line-height:0; margin:0px; max-height:none; max-width:none; min-height:0px; min-width:0px; overflow-wrap:normal; padding:1px 0px; position:relative; white-space:nowrap; word-spacing:normal" class="MathJax_CHTML mjx-chtml">p(2p+1)(2p)2−12=p(2p+1)4p−1

#Ye Chi-Lien

\(\frac{3}{\sqrt{3}+1}=\frac{3\left(\sqrt{3}-1\right)}{\left(\sqrt{3}+1\right)\left(\sqrt{3}-1\right)}=\frac{3\sqrt{3}-3}{3-1}=\frac{3\sqrt{3}-3}{2}\)

\(\frac{2}{\sqrt{3}-1}=\frac{2\left(\sqrt{3}+1\right)}{\left(\sqrt{3}-1\right)\left(\sqrt{3}+1\right)}=\frac{2\left(\sqrt{3}+1\right)}{3-1}=\sqrt{3}-1\)

\(\frac{2+\sqrt{3}}{2-\sqrt{3}}=\frac{\left(2+\sqrt{3}\right)^2}{\left(2-\sqrt{3}\right)\left(2+\sqrt{3}\right)=4-3}=\left(2+\sqrt{3}\right)^2=4+4\sqrt{3}+3=7+4\sqrt{3}\)

\(\frac{b}{3+\sqrt{b}}=\frac{b\left(3-\sqrt{b}\right)}{\left(3+\sqrt{b}\right)\left(3-\sqrt{b}\right)}=\frac{b\left(3-\sqrt{b}\right)}{9-b}\)

\(\frac{p}{2\sqrt{p}-1}=\frac{p\left(2\sqrt{p}+1\right)}{\left(2\sqrt{p}-1\right)\left(2\sqrt{b}+1\right)}=\frac{p\left(2\sqrt{b}+1\right)}{4p-1}\)

+) \dfrac{3}{\sqrt{3}+1}=\dfrac{3(\sqrt{3}-1)}{(\sqrt{3}+1)(\sqrt{3}-1)}=\dfrac{3(\sqrt{3}-1)}{3-1}=\dfrac{3(\sqrt{3}-1)}{2}3​+13​=(3​+1)(3​−1)3(3​−1)​=3−13(3​−1)​=23(3​−1)​.

+) \dfrac{2}{\sqrt{3}-1}=\dfrac{2(\sqrt{3}+1)}{(\sqrt{3}-1)(\sqrt{3}+1)}=\dfrac{2(\sqrt{3}+1)}{3-1}=\sqrt{3}+13​−12​=(3​−1)(3​+1)2(3​+1)​=3−12(3​+1)​=3​+1.

+) \dfrac{2+\sqrt{3}}{2-\sqrt{3}}=\dfrac{(2+\sqrt{3})(2+\sqrt{3)}}{(2-\sqrt{3})(2+\sqrt{3})}=\dfrac{4+4 \sqrt{3}+3}{2^{2}-(\sqrt{3})^{2}}=7+4 \sqrt{3}2−3​2+3​​=(2−3​)(2+3​)(2+3​)(2+3)​​=22−(3​)24+43​+3​=7+43​.

+) \dfrac{b}{3+\sqrt{b}}=\dfrac{b(3-\sqrt{b})}{(3+\sqrt{b})(3-\sqrt{b})}=\dfrac{b(3-\sqrt{b})}{3^{2}-(\sqrt{b})^{2}}=\dfrac{b(3-\sqrt{b})}{9-b}3+b​b​=(3+b​)(3−b​)b(3−b​)​=32−(b​)2b(3−b​)​=9−bb(3−b​)​.

+) \dfrac{p}{2 .\sqrt{p}-1}=\dfrac{p(2 .\sqrt{p}+1)}{(2 .\sqrt{p}-1)(2 .\sqrt{p}+1)}=\dfrac{p(2 \sqrt{p}+1)}{(2 \sqrt{p})^{2}-1^{2}}=\dfrac{2 p.\sqrt{p}+p}{4 p-1}2.p​−1p​=(2.p​−1)(2.p​+1)p(2.p​+1)​=(2p​)2−12p(2p​+1)​=4p−