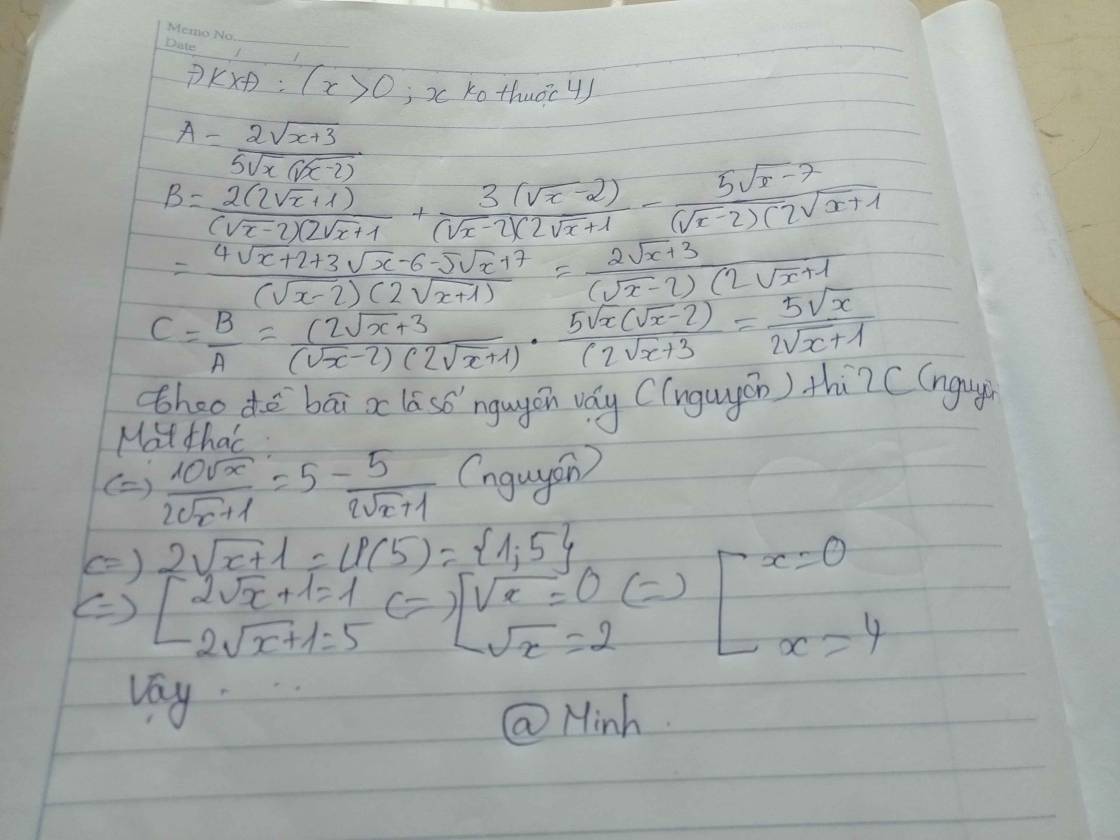Cho biểu thức A= 5x+ 7 - \(\sqrt{x^2-4x+4}\) . Tìm x để A=5

Những câu hỏi liên quan
Bài 1 : Cho biểu thức R left[frac{2sqrt{x}}{sqrt{x}+3}+frac{sqrt{x}}{sqrt{x}-3}-frac{3cdotleft(sqrt{x}+3right)}{x-9}right]:left(frac{2sqrt{x}-2}{sqrt{x}-3}-1right)a/ Rút gọn Rb/ Tìm các giá trị của x để R -1Bài 2 : Cho sqrt{x^2-5x+14}-sqrt{x^2-5x+10}2Tính giá trị biểu thức M sqrt{x^2-5x+14}+sqrt{x^2-5x+10}Bài 3 : Tìm GTNN của : Q sqrt{x^2+4x+4}+sqrt{x^2-4x+4}
Đọc tiếp
Bài 1 : Cho biểu thức R = \(\left[\frac{2\sqrt{x}}{\sqrt{x}+3}+\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3\cdot\left(\sqrt{x}+3\right)}{x-9}\right]:\left(\frac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\)
a/ Rút gọn R
b/ Tìm các giá trị của x để R < -1
Bài 2 : Cho \(\sqrt{x^2-5x+14}-\sqrt{x^2-5x+10}=2\)Tính giá trị biểu thức M =\(\sqrt{x^2-5x+14}+\sqrt{x^2-5x+10}\)
Bài 3 : Tìm GTNN của : Q = \(\sqrt{x^2+4x+4}+\sqrt{x^2-4x+4}\)
\(R=\left[\frac{2\sqrt{x}}{\sqrt{x}+3}+\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3\left(\sqrt{x}+3\right)}{x-9}\right]:\left(\frac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\)
a/ \(R=\left[\frac{2\sqrt{x}}{\sqrt{x}+3}+\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3\left(\sqrt{x}+3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt[]{x-3}\right)}\right]:\left(\frac{2\sqrt{x}-2-\sqrt{x}+3}{\sqrt{x}-3}\right)\)
=> \(R=\left[\frac{2\sqrt{x}}{\sqrt{x}+3}+\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3}{\sqrt[]{x-3}}\right]:\frac{\sqrt{x}+1}{\sqrt{x}-3}\)
=> \(R=\left[\frac{2\sqrt{x}}{\sqrt{x}-3}+1\right]:\frac{\sqrt{x}+1}{\sqrt{x}-3}\)
=> \(R=\left[\frac{2\sqrt{x}+\sqrt{x}-3}{\sqrt{x}-3}\right].\frac{\sqrt{x}-3}{\sqrt{x}+1}\)
=> \(R=\frac{3\sqrt{x}-3}{\sqrt{x}-3}.\frac{\sqrt{x}-3}{\sqrt{x}+1}=\frac{3\left(\sqrt{x}-1\right)}{\sqrt{x}+1}\)
b/ Để R<-1 => \(\frac{3\left(\sqrt{x}-1\right)}{\sqrt{x}+1}< -1\)
<=> \(3\sqrt{x}-3< -\sqrt{x}-1\)
<=> \(4\sqrt{x}< 2\)=> \(\sqrt{x}< \frac{1}{2}\) => \(-\frac{1}{4}< x< \frac{1}{4}\)
Đúng 0
Bình luận (0)
Chỗ => R = \(\left(\frac{2\sqrt{x}}{\sqrt{x}-3}+1\right):\frac{\sqrt{x}+1}{\sqrt{x}-3}\) là sao vậy ạ?
Đúng 0
Bình luận (0)
Thì \(\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3}{\sqrt{x}-3}=\frac{\sqrt{x}-3}{\sqrt{x}-3}=1\)
Đúng 0
Bình luận (0)
tìm x để biểu thức sau có nghĩa
a, \(\sqrt{2-4x}\)
b, \(\sqrt{4\left(x-5\right)}\)
\(a,ĐK:2-4x\ge0\Leftrightarrow x\le\dfrac{1}{2}\\ b,ĐK:4\left(x-5\right)\ge0\Leftrightarrow x-5\ge0\left(4>0\right)\Leftrightarrow x\ge5\)
Đúng 1
Bình luận (0)
Bài 1: * Cho biểu thức A 5x - sqrt{4x^2-4x+1}
a, Rút gọn A
b, Tính giá trị của biểu thức A với x -2
* Cho biểu thức B 2x - sqrt{x^2+4x+4}
a, Rút gọn B
b, Tính giá trị của biểu thức B để B -10
* Cho biểu thức C frac{sqrt{4x^2-4x+1}}{4x^2-1}
a, Rút gọn C
b, Tính giá trị của x để C frac{-1}{5}
c, Tính giá trị của x để C 0
Đọc tiếp
Bài 1: * Cho biểu thức A = 5x - \(\sqrt{4x^2-4x+1}\)
a, Rút gọn A
b, Tính giá trị của biểu thức A với x = -2
* Cho biểu thức B = 2x - \(\sqrt{x^2+4x+4}\)
a, Rút gọn B
b, Tính giá trị của biểu thức B để B = -10
* Cho biểu thức C = \(\frac{\sqrt{4x^2-4x+1}}{4x^2-1}\)
a, Rút gọn C
b, Tính giá trị của x để C = \(\frac{-1}{5}\)
c, Tính giá trị của x để C > 0
Cho biểu thức: \(A\) = \(\left(\dfrac{3+\sqrt{x}}{3-\sqrt{x}}-\dfrac{3-\sqrt{x}}{3+\sqrt{x}}-\dfrac{4x}{x-9}\right)\) : \(\left(\dfrac{5}{3-\sqrt{x}}-\dfrac{4\sqrt{x}+2}{3\sqrt{x}-x}\right)\) . Tìm đk của x để |A| > - A
ĐKXĐ: x>0; x<>9
\(A=\left(\dfrac{-\left(\sqrt{x}+3\right)}{\sqrt{x}-3}+\dfrac{\sqrt{x}-3}{\sqrt{x}+3}-\dfrac{4x}{x-9}\right):\left(\dfrac{5\sqrt{x}-4\sqrt{x}-2}{\sqrt{x}\left(3-\sqrt{x}\right)}\right)\)
\(=\dfrac{-x-6\sqrt{x}-9+x-6\sqrt{x}+9-4x}{x-9}:\dfrac{-\sqrt{x}+2}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
\(=\dfrac{-4x-12\sqrt{x}}{x-9}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{-\left(\sqrt{x}-2\right)}\)
\(=\dfrac{4x\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}{\left(x-9\right)\left(\sqrt{x}-2\right)}=\dfrac{4x}{\sqrt{x}-2}\)
|A|>-A
=>A>=0
=>4x>0
=>x>0 và x<>9
Đúng 0
Bình luận (0)
Cho biểu thức A=\(\dfrac{x\sqrt{x}-4x-\sqrt{x}+4}{2x\sqrt{x}-14x+28\sqrt{x}-16}\)
a/ Tìm x để A có nghĩa, từ đó rút gọn A.
b/ Tìm các giá trị nguyên của x để biểu thức A nhận giá trị nguyên
Lời giải:
a.
\(A=\frac{(x\sqrt{x}-4x)-(\sqrt{x}-4)}{2(\sqrt{x}-4)(\sqrt{x}-2)(\sqrt{x}-1)}\)
ĐKXĐ: \(\left\{\begin{matrix} x\geq 0\\ \sqrt{x}-4\neq 0\\ \sqrt{x}-2\neq 0\\ \sqrt{x}-1\neq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ x\neq 16\\ x\neq 4\\ x\neq 1\end{matrix}\right.\)
\(A=\frac{x(\sqrt{x}-4)-(\sqrt{x}-4)}{2(\sqrt{x}-4)(\sqrt{2}-2)(\sqrt{x}-1)}=\frac{(x-1)(\sqrt{x}-4)}{2(\sqrt{x}-4)(\sqrt{x}-2)(\sqrt{x}-1)}\)
\(=\frac{(\sqrt{x}-1)(\sqrt{x}+1)(\sqrt{x}-4)}{2(\sqrt{x}-4)(\sqrt{x}-2)(\sqrt{x}-1)}=\frac{\sqrt{x}+1}{2(\sqrt{x}-2)}\)
b.
Với $x$ nguyên, để $A\in\mathbb{Z}$ thì $\sqrt{x}+1\vdots 2(\sqrt{x}-2)}$
$\Rightarrow \sqrt{x}+1\vdots \sqrt{x}-2$
$\Leftrightarrow \sqrt{x}-2+3\vdots \sqrt{x}-2$
$\Leftrightarrow 3\vdots \sqrt{x}-2$
$\Rightarrow \sqrt{x}-2\in\left\{\pm 1;\pm 3\right\}$
$\Rightarrow x\in\left\{1;9;25\right\}$
Thử lại thấy đều thỏa mãn.
Đúng 1
Bình luận (0)
Cho biểu thức \(A=\dfrac{x\sqrt{x}-4x-\sqrt{x}+4}{2x\sqrt{x}-14x+28\sqrt{x}-16}\)
a)Tìm x để A có nghĩa,từ đó rút gọn A.
b)Tìm giá trị nguyên của x để biểu thức A nhận giá trị nguyên.
a: \(A=\dfrac{x\left(\sqrt{x}-4\right)-\left(\sqrt{x}-4\right)}{2x\sqrt{x}-8x-6x+24\sqrt{x}+4\sqrt{x}-16}\)
\(=\dfrac{\left(\sqrt{x}-4\right)\left(x-1\right)}{\left(\sqrt{x}-4\right)\left(2x-6\sqrt{x}+4\right)}=\dfrac{x-1}{2x-6\sqrt{x}+4}\)
\(=\dfrac{x-1}{2\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}+1}{2\sqrt{x}-4}\)
b: Để A nguyên thì \(2\sqrt{x}+2⋮2\sqrt{x}-4\)
\(\Leftrightarrow2\sqrt{x}-4\in\left\{2;-2;6\right\}\)
hay \(x\in\left\{9;1;25\right\}\)
Đúng 0
Bình luận (0)
Cho biểu thứcA=\(\left(4x^5+4x^4-5x^3+5x-2\right)^{2014}+2015\) Tính giá trị biểu thức A khi
x=\(\frac{1}{2}\sqrt{\frac{\sqrt{2}-1}{\sqrt{2}+1}}\)
1 Cho biểu thức Bfrac{xsqrt{x}-4x-sqrt{x}+4}{2xsqrt{x}-14x+28sqrt{x}-16}a) Tìm x để A có nghĩa, từ đó rút gọn biểu thức Bb) Tìm các giá trị nguyên của x để biểu thức B nhận giá trị nguyên2 cho biểu thức Pleft(frac{4sqrt{x}}{2+sqrt{x}}+frac{8x}{4-x}right)divleft(frac{sqrt{x}-1}{x-2sqrt{x}}-frac{2}{sqrt{x}}right)a) Rút gọn Pb) Tìm giá trị của x để P-13 Rút gọn Qfrac{2sqrt{4-sqrt{5+21+sqrt{80}}}}{sqrt{10}-sqrt{2}}
Đọc tiếp
1 Cho biểu thức B=\(\frac{x\sqrt{x}-4x-\sqrt{x}+4}{2x\sqrt{x}-14x+28\sqrt{x}-16}\)
a) Tìm x để A có nghĩa, từ đó rút gọn biểu thức B
b) Tìm các giá trị nguyên của x để biểu thức B nhận giá trị nguyên
2 cho biểu thức P=\(\left(\frac{4\sqrt{x}}{2+\sqrt{x}}+\frac{8x}{4-x}\right)\div\left(\frac{\sqrt{x}-1}{x-2\sqrt{x}}-\frac{2}{\sqrt{x}}\right)\)
a) Rút gọn P
b) Tìm giá trị của x để P=-1
3 Rút gọn Q=\(\frac{2\sqrt{4-\sqrt{5+21+\sqrt{80}}}}{\sqrt{10}-\sqrt{2}}\)
Cho hai biểu thức \(A=\dfrac{2\sqrt{x}+3}{5x-10\sqrt{x}}\) và \(B=\dfrac{2}{\sqrt{x}-2}+\dfrac{3}{2\sqrt{x}+1}-\dfrac{5\sqrt{x}-7}{2x-3\sqrt{x}-2}\) với x>0, x≠4. Tìm x sao cho \(\dfrac{B}{A}\)nhận giá trị là một số nguyên.