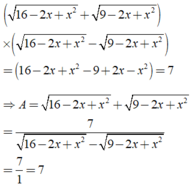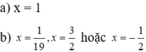2x-1=16

Những câu hỏi liên quan
Cho
16
-
2
x
+
x
2
-
9
-
2
x
+
x
2
1
.Tính giá trị của biểu thức
A
16
-
2
x
+
x
2
+
9
-
2
x...
Đọc tiếp
Cho 16 - 2 x + x 2 - 9 - 2 x + x 2 = 1 .Tính giá trị của biểu thức A = 16 - 2 x + x 2 + 9 - 2 x + x 2
A. A = 6
B. A = 3
C. A = 5
D. A = 7
cmr:1-2/x-(2x+x^2/4+2x+x^2 + 2x-x^2/4-2x+x^2):(16-8x/4-2x+x^2 -16+8x/4+2x+x^2)=(x-1/x)^2
Giải các phương trình sau:a)
2
x
+
1
2
−
x
+
2
2
+
3
x
1
−
x
0
;
b)
2
7...
Đọc tiếp
Giải các phương trình sau:
a) 2 x + 1 2 − x + 2 2 + 3 x 1 − x = 0 ;
b) 2 7 x − 1 6 + 2 x = 1 − 2 x 7 x − 1 6 + 2 x .
2^x-26=6
64.4^x=16^8
(2x-1)tất cả mũ4=16
(2x+1)tất cả mũ 3=125
1. \(2^x-26=6\)
\(\Rightarrow2^x=6+26\)
\(\Rightarrow2^x=32\)
\(\Rightarrow2^x=2^5\)
\(\Rightarrow x=5\)
2. \(64\cdot4^x=16^8\)
\(\Rightarrow4^3\cdot4^x=4^{16}\)
\(\Rightarrow4^x=4^{16}:4^3\)
\(\Rightarrow4^x=4^{13}\)
\(\Rightarrow x=13\)
3. \(\left(2x-1\right)^4=16\)
\(\Rightarrow\left(2x-1\right)^4=2^4\)
\(\Rightarrow2x-1=2\)
\(\Rightarrow2x=3\)
\(\Rightarrow x=\dfrac{3}{2}\)
4. \(\left(2x+1\right)^3=125\)
\(\Rightarrow\left(2x+1\right)^3=5^3\)
\(\Rightarrow2x+1=5\)
\(\Rightarrow2x=4\)
\(\Rightarrow x=2\)
Đúng 1
Bình luận (0)
2x + 2x+1 + 2x+2 +......+2x+2021 =22026 -16
đặt A=2^x +2^x+1 +.....+2^x+2021=2^x+2026-16
đặt 2A = 2^x+1 +2^x+2 +......+2^x+2022=2^x+2027-32
lấy 2A-A =2^x+2022-2^x=2^2026-16
vậy,ta suy ra x=4
Đúng 0
Bình luận (0)
2x + 2x+1 + 2x+2 +......+2x+2021 =22026 -16
=>\(2^x\left(1+2+2^2+...+2^{2021}\right)=2^4\left(2^{2022}-1\right)\)
=>2^x=2^4
=>x=4
Đúng 1
Bình luận (0)
2x + 2x+1+ 2x+2 + .... + 2x+2021 = 22026 - 16
Đặt \(A=2^x+2^{x+1}+...+2^{x+2021}=2^{x+2026-16}\)
Đặt \(2A=2^{x+1}+2^{x+2}+...+2^{x+2022}=2^{x+2027+32}\)
Ta lấy \(2A-A=2^{x+2022}-2^x=2^{2026-16}\)
\(\Rightarrow x=4\)
Vậy \(x=4\)
Đúng 3
Bình luận (0)
\(2VT=2^{x+1}+2^{x+2}+2^{x+3}+...+2^{x+2022}\)
\(VT=2VT-VT=2^{x+2022}-2^x\)
\(\Rightarrow2^{x+2022}-2^x=2^{2026}-16\)
\(\Leftrightarrow2^{2022}.2^x-2^x=2^{2026}-2^4\)
\(\Leftrightarrow2^x\left(2^{2022}-1\right)=2^4\left(2^{2022}-1\right)\)
\(\Leftrightarrow2^x=2^4\Rightarrow x=4\)
Đúng 3
Bình luận (0)
\(2^x+2^{x+1}+2^{x+2}+...+2^{x+2021}=2^{2026}-16\)
\(\Rightarrow2^x\left(1+2^x+2^{x+1}+...+2^{x+2020}\right)=2^{2026}-2^4\)
\(\Rightarrow2^x.\dfrac{2^{x+2020+1}-1}{2-1}=2^4.\left(2^{2022}-1\right)\)
\(\Rightarrow2^x.\left(2^{x+2021}-1\right)=2^4.\left(2^{2022}-1\right)\)
\(\Rightarrow x\in\varnothing\)
Đúng 2
Bình luận (0)
Xem thêm câu trả lời
2x-1*1-2x=-16
2x-1*1-2x=-16
2x-1-2x=-16
2x-2x-1=-16
0-1=-16
suy ra ko có x thỏa mãn
Đúng 0
Bình luận (0)
3(2x^2+1)(2x^4+1)(2x^8+1)(2x^16+1)+1
Rút gọn
Giải các phương trình sau:
1) \(2^{x^2-5x+6} + 2^{1-x^2} = 2. 2^{6-5x} + 1\)
2) \(16^{\sin^2x} + 16^{\cos^2x} = 10\)
1.
PT $\Leftrightarrow 2^{x^2-5x+6}+2^{1-x^2}-2^{7-5x}-1=0$
$\Leftrightarrow (2^{x^2-5x+6}-2^{7-5x})-(1-2^{1-x^2})=0$
$\Leftrightarrow 2^{7-5x}(2^{x^2-1}-1)-(2^{x^2-1}-1)2^{1-x^2}=0$
$\Leftrightarrow (2^{x^2-1}-1)(2^{7-5x}-2^{1-x^2})=0$
$\Rightarrow 2^{x^2-1}-1=0$ hoặc $2^{7-5x}-2^{1-x^2}=0$
Nếu $2^{x^2-1}=1\Leftrightarrow x^2-1=0$
$\Leftrightarrow x^2=1\Leftrightarrow x=\pm 1$
$2^{7-5x}-2^{1-x^2}=0$
$\Leftrightarrow 7-5x=1-x^2\Leftrightarrow x^2-5x+6=0$
$\Leftrightarrow (x-2)(x-3)=0\Leftrightarrow x=2; x=3$
Đúng 1
Bình luận (0)
2. Đặt $\sin ^2x=a$ thì $\cos ^2x=1-a$. PT trở thành:
$16^a+16^{1-a}=10$
$\Leftrightarrow 16^a+\frac{16}{16^a}=10$
$\Leftrightarrow (16^a)^2-10.16^a+16=0$
Đặt $16^a=x$ thì:
$x^2-10x+16=0$
$\Leftrightarrow (x-2)(x-8)=0$
$\Leftrightarrow x=2$ hoặc $x=8$
$\Leftrightarrow 16^a=2$ hoặc $16^a=8$
$\Leftrightarrow 2^{4a}=2$ hoặc $2^{4a}=2^3$
$\Leftrightarroww 4a=1$ hoặc $4a=3$
$\Leftrightarrow a=\frac{1}{4}$ hoặc $a=\frac{3}{4}$
Nếu $a=\frac{1}{4}\Leftrightarrow \sin ^2x=\frac{1}{4}$
$\Leftrightarrow \sin x=\pm \frac{1}{2}$
Nếu $a=\sin ^2x=\frac{3}{4}\Rightarrow \sin x=\pm \frac{\sqrt{3}}{2}$
Đến đây thì đơn giản rồi.
Đúng 1
Bình luận (0)