ABCD là hình vuông

\(\Rightarrow\Delta ABD\&\Delta ACD\) là tam vuông cân

\(\Rightarrow\left\{{}\begin{matrix}\left|\overrightarrow{AC}\right|=\left|\overrightarrow{AD}\right|.\sqrt[]{2}\\\left|\overrightarrow{BD}\right|=\left|\overrightarrow{AB}\right|.\sqrt[]{2}\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}\left|\overrightarrow{AC}\right|=\dfrac{\sqrt[]{2}}{2}.\sqrt[]{2}=1\\\left|\overrightarrow{BD}\right|=\dfrac{\sqrt[]{2}}{2}.\sqrt[]{2}=1\end{matrix}\right.\)

\(\left|\overrightarrow{OA}\right|=\left|\overrightarrow{AO}\right|=\dfrac{1}{2}.\left|\overrightarrow{AC}\right|\)  (O là trung điểm AC)

\(\Rightarrow\left|\overrightarrow{OA}\right|=\left|\overrightarrow{AO}\right|=\dfrac{1}{2}.1=\dfrac{1}{2}\)

Ta có: \(AC = BD = \sqrt {A{D^2} + D{C^2}}  = \sqrt {{{\left( {\frac{{\sqrt 2 }}{2}} \right)}^2} + {{\left( {\frac{{\sqrt 2 }}{2}} \right)}^2}}  = 1\)

\(OA = \frac{1}{2}AC = \frac{1}{2}\)

Suy ra: \(\left| {\overrightarrow {AC} } \right| = 1\), \(\left| {\overrightarrow {BD} } \right| = 1\), \(\left| {\overrightarrow {OA} } \right| = 1\), \(\left| {\overrightarrow {AO} } \right| = 1\)

a) Do ABCD cũng là một hình bình hành nên \(\overrightarrow {DA}  + \overrightarrow {DC}  = \overrightarrow {DB} \)

\( \Rightarrow \;|\overrightarrow {DA}  + \overrightarrow {DC} |\; = \;|\overrightarrow {DB} |\; = DB = a\sqrt 2 \)

b) Ta có: \(\overrightarrow {AD}  + \overrightarrow {DB}  = \overrightarrow {AB} \) \( \Rightarrow \overrightarrow {AB}  - \overrightarrow {AD}  = \overrightarrow {DB} \)

\( \Rightarrow \left| {\overrightarrow {AB}  - \overrightarrow {AD} } \right| = \left| {\overrightarrow {DB} } \right| = DB = a\sqrt 2 \)

c) Ta có: \(\overrightarrow {DO}  = \overrightarrow {OB} \)

\( \Rightarrow \overrightarrow {OA}  + \overrightarrow {OB}  = \overrightarrow {OA}  + \overrightarrow {DO}  = \overrightarrow {DO}  + \overrightarrow {OA}  = \overrightarrow {DA} \)

\( \Rightarrow \left| {\overrightarrow {OA}  + \overrightarrow {OB} } \right| = \left| {\overrightarrow {DA} } \right| = DA = a.\)

Ta có: \(AB = BC = CD = DA = 1;\)

            \(AC = BD = \sqrt {A{B^2} + B{C^2}}  = \sqrt {{1^2} + {1^2}}  = \sqrt 2 \)

a) \(\overrightarrow a  = \overrightarrow {OB}  - \overrightarrow {OD}  = \overrightarrow {OB}  + \overrightarrow {DO}  = \left( {\overrightarrow {DO}  + \overrightarrow {OB} } \right) = \overrightarrow {DB} \)

\( \Rightarrow \left| {\overrightarrow a } \right| = \left| {\overrightarrow {DB} } \right| = DB = \sqrt 2 \)

b)  \(\overrightarrow b = \left( {\overrightarrow {OC}  - \overrightarrow {OA} } \right) + \left( {\overrightarrow {DB}  - \overrightarrow {DC} } \right)\)

   \( = \left( {\overrightarrow {OC}  + \overrightarrow {AO} } \right) + \left( {\overrightarrow {DB}  + \overrightarrow {CD} } \right) = \left( {\overrightarrow {AO}  + \overrightarrow {OC} } \right) + \left( {\overrightarrow {CD}  + \overrightarrow {DB} } \right)\)

   \( = \overrightarrow {AC}  + \overrightarrow {CB}  = \overrightarrow {AB} \)

\( \Rightarrow \left| {\overrightarrow b } \right| = \left| {\overrightarrow {AB} } \right| = AB = 1\)

Chú ý khi giải:

Khi có dấu trừ phía trước ta thường thay bằng vectơ đối của nó và ngược lại

a)       \(\begin{array}{l}\overrightarrow a  = \left( {\overrightarrow {AC}  + \overrightarrow {BD} } \right) + \overrightarrow {CB}  = \left( {\overrightarrow {AC}  + \overrightarrow {CB} } \right) + \overrightarrow {BD} \\ = \overrightarrow {AB}  + \overrightarrow {BD}  = \overrightarrow {AD}\\  \Rightarrow |{\overrightarrow a}|= \left| {\overrightarrow {AD} } \right| = AD = 1\end{array}\)

b)       \(\begin{array}{l}\overrightarrow a  = \overrightarrow {AB}  + \overrightarrow {AD}  + \overrightarrow {BC}  + \overrightarrow {DA}  = \left( {\overrightarrow {AB}  + \overrightarrow {BC} } \right) + \left( {\overrightarrow {AD}  + \overrightarrow {DA} } \right)\\ = \overrightarrow {AC}  + \overrightarrow {AA}  = \overrightarrow {AC}  + \overrightarrow 0  = \overrightarrow {AC} \end{array}\)

\(AC = \sqrt {A{B^2} + B{C^2}}  = \sqrt {{1^2} + {1^2}}  = \sqrt 2 \)

\(\Rightarrow |{\overrightarrow a}|= \left| {\overrightarrow {AC} } \right| = \sqrt 2 \)

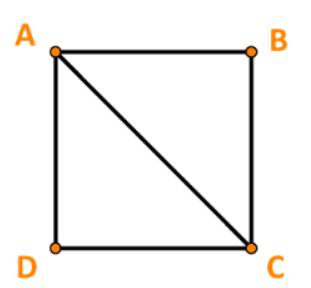
Ta có: \(|\overrightarrow {AB} | = AB\) và \(|\overrightarrow {AC} |\; = AC.\)

Mà \(AB = 3,\;AC = \sqrt {A{B^2} + B{C^2}}  = \sqrt {{3^2} + {3^2}}  = 3\sqrt 2  \)

\( \Rightarrow \;|\overrightarrow {AB} |\, = 3;\;\;|\overrightarrow {AC} |\, = 3\sqrt 2 \)

Tham khảo:

\(\overrightarrow {CD}  = \overrightarrow {BA} \) do hai vectơ \(\overrightarrow {CD} ,\;\overrightarrow {BA} \) cùng hướng và \(CD = BA\).

\(\begin{array}{l} \Rightarrow \overrightarrow {CB}  + \overrightarrow {CD}  = \overrightarrow {CB}  + \overrightarrow {BA}  = \overrightarrow {CA} \\ \Leftrightarrow \left| {\overrightarrow {CB}  + \overrightarrow {CD} } \right| = \left| {\overrightarrow {CA} } \right| = CA\end{array}\)

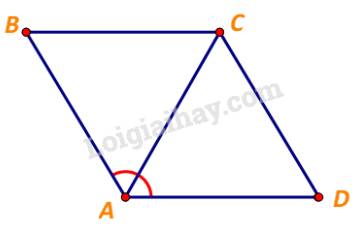
Xét tam giác ABC, ta có:

\(BA = BC\) và \(\widehat {BAC} = \frac{1}{2}.\widehat {BAD} = {60^o}\)

\( \Rightarrow \Delta ABC\) đều, hay \(CA = BC = 1\)

Vậy \(\left| {\overrightarrow {CB}  + \overrightarrow {CD} } \right| = 1.\)

Dựa vào tính chất kết hợp, ta có:

\(\begin{array}{l}\overrightarrow {DB}  + \overrightarrow {CD}  + \overrightarrow {BA}  = \left( {\overrightarrow {DB}  + \overrightarrow {CD} } \right) + \overrightarrow {BA} \\ = \left( {\overrightarrow {CD}  + \overrightarrow {DB} } \right) + \overrightarrow {BA}  = \overrightarrow {CB}  + \overrightarrow {BA}  = \overrightarrow {CA} .\\ \Rightarrow \left| {\overrightarrow {DB}  + \overrightarrow {CD}  + \overrightarrow {BA} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1.\end{array}\)

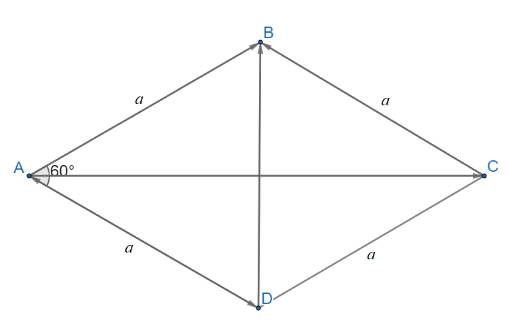
+) ABCD là hình thoi nên cũng là hình bình hành

 Áp dụng quy tắc hình bình hành ta có:

 \(\overrightarrow p  = \overrightarrow {AB}  + \overrightarrow {AD}  = \overrightarrow {AC} \)

 \(\Rightarrow  |\overrightarrow p|  = | \overrightarrow {AC}| =AC \)

+) \(\overrightarrow u  = \overrightarrow {AB}  - \overrightarrow {AD}  = \overrightarrow {DB} \)

 \(\Rightarrow  |\overrightarrow u|  = | \overrightarrow {DB}| =DB\)

+) \(\overrightarrow v  = 2\overrightarrow {AB}  - \overrightarrow {AC}  = \overrightarrow {AB}  + \left( {\overrightarrow {AB}  - \overrightarrow {AC} } \right) = \overrightarrow {AB}  + \overrightarrow {CB} \)\( = \overrightarrow {AB}  + \overrightarrow {DA}  = \overrightarrow {DB} \)

 \(\Rightarrow  |\overrightarrow v|  = | \overrightarrow {DB}| =DB\)

+ Tính \(AC, DB\)

Tam giác ABD có \(AB=AD=a, \widehat A = 60^o\) nên nó là tam giác đều. Do đó DB = a.

Gọi O là giao điểm hai đường chéo.

Ta có: \(AO = AB. \sin B = a. \sin 60^o = \frac {a \sqrt 3}{2} \Rightarrow  AC = a \sqrt 3\)

Vậy \(|\overrightarrow p|  =  a \sqrt 3 ,|\overrightarrow u|  =  a, |\overrightarrow v|  =  a.\)

5

Áp dụng định lý Pi-ta-go trong tam giác vuông ABC ta có:
\(AC=\sqrt{AB^2+BC^2}=\sqrt{3^2+4^2}=5\).
Vậy \(\left|\overrightarrow{AC}\right|=5\).