Giai các bpt sau :
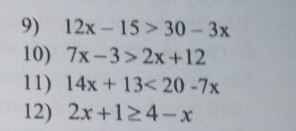
Giai bpt sau x2+4x+3<0
x2+x+3x+3<0
<=> x(x+1) + 3(x+1)<0
<=> (x+3)(x+1)<0
Vì tích 2 số trái dấu nhân với nhau ra kết quả âm nên x+3 và x+1 trái dấu
Trường hợp 1: x+3>0 thì x+1<0
<=> x>-3 và x<-1
<=> -3<x<-1
Trường hợp 2: x+3<0 thì x+1>0
<=> x<-3 và x>-1 (Vô lý)
Vậy -3<x<-1
Ta có x2+4x+3<0 (1)
<=>(x2+4x+4)-1<0
<=>(x-2)^2-1<0 mà (x-2)^2=<0
Vậy BPT(1) đúng
x2+4x+3<0 <=>x2+4x+4-1<0 <=> (x+2)2<1 <=> -1<x+2<1 <=> x+2=0<=> x= -2
làm lụi sai thôi nha
Giai bpt sau (x+1)(x+3)<0 m.n giup minh nhanh nhe
ĐỂ (x+1)(x+3)< 0 khi x+1>0, x+3< 0 hoặc x+1<0,x+3> 0
x>-1,x>-3 => x>-1
hoặc x<-1,x<-3 => x<-3
vậy với x>-1 hoặc x<-3 thi (x+1)(x+3) <0
Vì tích trên < 0 => x+1 và x+3 trái dấu.mà x+3-(x+1)=2=>x+3>x+1=>x+3 mang dấu + và x+1 ngược lại=>x+3>0 và x+1 cũng ngược lại
=>nếu x+3>0=>x>3(1)
x+1<0=>x<1(2)
Từu 1 và 2 => 3<x<1
Ủa, vô lí, hì vậy x ko có gt nhé
Nhưng đây là toán nâng cao lớp 6 đó bn ơi
Giai các bpt sau
a,(x+2)(x-3)>0
b,(x-1)(x+4)≤0
giúp mk vs ạ ai nhanh mk tick nka
a: (x+2)(x-3)>0
nên x+2;x-3 cùng dấu
=>x>3 hoặc x<-2
b: (x-1)(x+4)<=0
nên x-1 và x+4 khác dấu
=>-4<=x<=1
1.giai bpt sau voi m la tham so:mx+1>=m^2+x
2
a.x^2-2x+1<9
b.1-5x/x-1>=1
Giải các bpt sau:
Giải các bpt sau:
e, ĐK: \(x\ne2\)
\(\dfrac{3}{x-2}>1\Leftrightarrow\dfrac{5-x}{x-2}>0\)
\(\Leftrightarrow\left\{{}\begin{matrix}5-x>0\\x-2>0\end{matrix}\right.\left(1\right)\) hoặc \(\left\{{}\begin{matrix}5-x< 0\\x-2< 0\end{matrix}\right.\left(2\right)\)
\(\left(1\right)\Leftrightarrow2< x< 5\)
\(\left(2\right)\Leftrightarrow\) vô nghiệm
Vậy \(2< x< 5\)
f, ĐK: \(x\ne\dfrac{1}{2}\)
\(\dfrac{2x^2+x}{1-2x}\ge1-x\)
\(\Leftrightarrow\dfrac{2x^2+x+\left(x-1\right)\left(1-2x\right)}{\left(1-2x\right)\left(x-1\right)}\ge0\)
\(\Leftrightarrow\dfrac{4x-1}{\left(1-2x\right)\left(x-1\right)}\ge0\)
\(\Leftrightarrow\left\{{}\begin{matrix}4x-1\ge0\\\left(1-2x\right)\left(x-1\right)>0\end{matrix}\right.\left(1\right)\) hoặc \(\left\{{}\begin{matrix}4x-1\le0\\\left(1-2x\right)\left(x-1\right)< 0\end{matrix}\right.\left(2\right)\)
\(\left(1\right)\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{4}\\\dfrac{1}{2}< x< 1\end{matrix}\right.\Leftrightarrow\dfrac{1}{2}< x< 1\)
\(\left(2\right)\Leftrightarrow\left\{{}\begin{matrix}x\le\dfrac{1}{4}\\\left[{}\begin{matrix}x>1\\x< \dfrac{1}{2}\end{matrix}\right.\end{matrix}\right.\Leftrightarrow x\le\dfrac{1}{4}\)
Vậy ...
Giải các pt, bpt sau:
\(\dfrac{x+1}{x+2}-\dfrac{5}{x-2}=\dfrac{20}{4-x^2}\) (\(ĐK:x\)≠\(2;-2\))
⇔ \(\dfrac{\left(x+1\right)\left(x-2\right)-5\left(x+2\right)}{x^2-4}=\dfrac{20}{4-x^2}\)
⇔ \(-\left(x+1\right)\left(x-2\right)+5\left(x+2\right)=20\)
⇔ \(-\left(x^2-2x+x-2\right)+5x+10=20\)
⇔ \(-x^2+x+2+5x+10-20=0\)
⇔ \(-x^2+6x-8=0\)
⇔ \(-\left(x^2-6x+9\right)=-1\)
⇔ \(\left(x-3\right)^2=1\)
⇔ \(\left[{}\begin{matrix}x-3=1\\x-3=-1\end{matrix}\right.\)
⇔ \(\left[{}\begin{matrix}x=4\\x=2\end{matrix}\right.\)
Vậy ...
b: \(\Leftrightarrow20-5\left(3x+2\right)>4\left(x+7\right)\)
=>20-15x-10>4x+28
=>-15x+10-4x-28>0
=>-19x-18>0
=>-19x>18
hay x<-18/19
giai bpt (x+3)/3 căn (1-x) < (2 căn (x+9))/x
Giai các bpt sau
a,\(\left(x-1^{ }\right)^2+x^2\le\left(x+1\right)^2+\left(x+2^{ }\right)^2\)
b,\(\left(x^2+1\right)\left(x-6\right)\le\left(x-2\right)^3\)
\(a,\left(x-1\right)^2+x^2\le\left(x+1\right)^2+\left(x+2\right)^2\\ \Leftrightarrow x^2-2x+1+x^2\le x^2+2x+1+x^2+4x+4\\ \Leftrightarrow2x^2-2x+1\le2x^2+6x+5\\ \Leftrightarrow-8x-6\le0\\ \Leftrightarrow x\ge\dfrac{3}{4}\)
\(b,\left(x^2+1\right)\left(x-6\right)\le\left(x-2\right)^3\\ \Leftrightarrow x^3+x-6x^2-6\le x^3-6x^2+12x-8\\ \Leftrightarrow11x-2\ge0\\ \Leftrightarrow x\ge\dfrac{2}{11}\)
a: \(\Leftrightarrow x^2-2x+1+x^2< =x^2+2x+1+x^2+4x+4\)
=>-2x+1<=6x+5
=>-7x<=4
hay x>=-4/7
b: \(\Leftrightarrow x^3-6x^2+x-6-x^3+6x^2-12x+8< =0\)
=>-11x+2<=0
=>-11x<=-2
hay x>=2/11