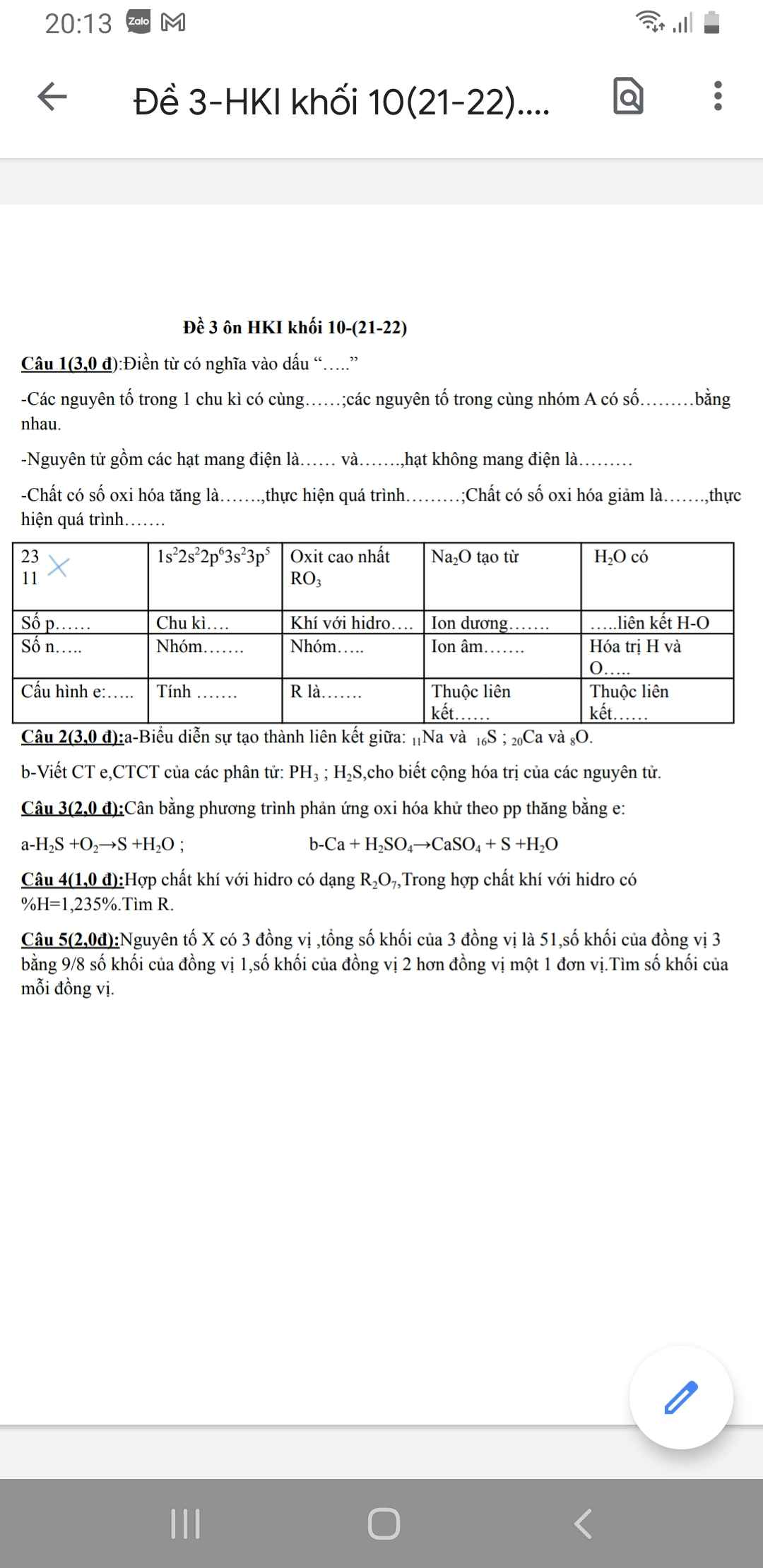
Làm dùm e câu 6 với ạ.

Những câu hỏi liên quan
Câu 7,8,10 MN làm giúp e với ạ. Ghi rõ cách làm dùm e với ạ. 
7.
ĐKXĐ: \(3\le x\le7\)
\(x-5+\sqrt{7-x}-\sqrt{x-3}=0\)
Đặt \(\left\{{}\begin{matrix}\sqrt{x-3}=a\ge0\\\sqrt{7-x}=b\ge0\end{matrix}\right.\) \(\Rightarrow a^2-b^2=2\left(x-5\right)\)
Pt trở thành:
\(\dfrac{a^2-b^2}{2}+b-a=0\)
\(\Leftrightarrow\left(a-b\right)\left(a+b-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}a=b\\a+b=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-3}=\sqrt{7-x}\\\sqrt{x-3}+\sqrt{7-x}=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=7-x\\4+2\sqrt{\left(x-3\right)\left(7-x\right)}=4\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=5\\x=7\end{matrix}\right.\)
Đúng 1
Bình luận (0)
8.
ĐKXĐ: \(-2\le x\le2\)
Đặt \(\sqrt{x+2}+\sqrt{2-x}=t\Rightarrow2\le t\le2\sqrt{2}\)
\(t^2=4+2\sqrt{4-x^2}\Rightarrow2\sqrt{-x^2+4}=t^2-4\)
Pt trở thành:
\(t+t^2-4+2m+3=0\)
\(\Leftrightarrow t^2+t+1=-2m\)
Xét hàm \(f\left(t\right)=t^2+t+1\) trên \(\left[2;2\sqrt{2}\right]\)
\(a=1>0;-\dfrac{b}{2a}=-\dfrac{1}{2}< 2\Rightarrow f\left(t\right)\) đồng biến trên đoạn đã cho
\(\Rightarrow f\left(2\right)\le f\left(t\right)\le f\left(2\sqrt{2}\right)\Rightarrow7\le f\left(t\right)\le9+2\sqrt{2}\)
\(\Rightarrow7\le-2m\le9+2\sqrt{2}\Rightarrow-\dfrac{9+2\sqrt{2}}{2}\le m\le-\dfrac{7}{2}\)
\(\Rightarrow m=\left\{-5;-4\right\}\)
Đúng 1
Bình luận (0)
Xem thêm câu trả lời
Làm dùm e câu 6,7 với ạ.
Câu 6:
Tọa độ A là:
\(\left\{{}\begin{matrix}x+5y-7=0\\x+3y-3=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+5y=7\\x+3y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x+5y-x-3y=7-3\\x+3y=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=2\\x=3-3\cdot2=-3\end{matrix}\right.\)
Vậy: A(-3;2)
M(-1;0) là trung điểm của AB
=>\(\left\{{}\begin{matrix}x_A+x_B=2\cdot x_M\\y_A+y_B=2\cdot y_M\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x_B-3=2\cdot\left(-1\right)=-2\\y_B+2=0\end{matrix}\right.\Leftrightarrow B\left(1;-2\right)\)
Phương trình đường cao kẻ từ A xuống BC là x+3y-3=0
=>VTPT là (1;3)
=>BC nhận vecto (-3;1) làm vecto pháp tuyến
Phương trình đường thẳng CB là:
-3(x-1)+1(y+2)=0
=>-3x+3+y+2=0
=>-3x+y+5=0
Gọi N là trung điểm của BC
=>N là giao điểm của hai đường thẳng -3x+y+5=0 và x+5y-7=0
Tọa độ N là:
\(\left\{{}\begin{matrix}-3x+y+5=0\\x+5y-7=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-3x+y=-5\\x+5y=7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-3x+y=-5\\3x+15y=21\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}-3x+y+3x+15y=-5+21\\x+5y=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}16y=16\\x=7-5y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=1\\x=7-5=2\end{matrix}\right.\)
vậy: N(2;1)
Xét ΔABC có
N,M lần lượt là trung điểm của BC,BA
=>NM là đường trung bình
=>NM//AC
N(2;1); M(-1;0)
=>\(\overrightarrow{NM}=\left(-3;-1\right)=\left(3;1\right)\)
=>AC nhận vecto (3;1) làm vecto chỉ phương
=>VTPT là (-1;3)
Phương trình đường thẳng AC là:
-1(x+3)+3(y-2)=0
=>-x-3+3y-6=0
=>-x+3y-9=0
Đúng 0
Bình luận (0)
Câu 12 làm sao v ạ. Ghi rõ cách làm dùm e với ạ. 
Pt bậc 2 có 2 nghiệm trái dấu khi \(ac< 0\)
\(\Leftrightarrow\left(m^2-4\right)m< 0\)
\(\Leftrightarrow m\in\left(-\infty;-2\right)\cup\left(0;2\right)\)
Đúng 1
Bình luận (0)
Làm dùm e bài 4 với ạ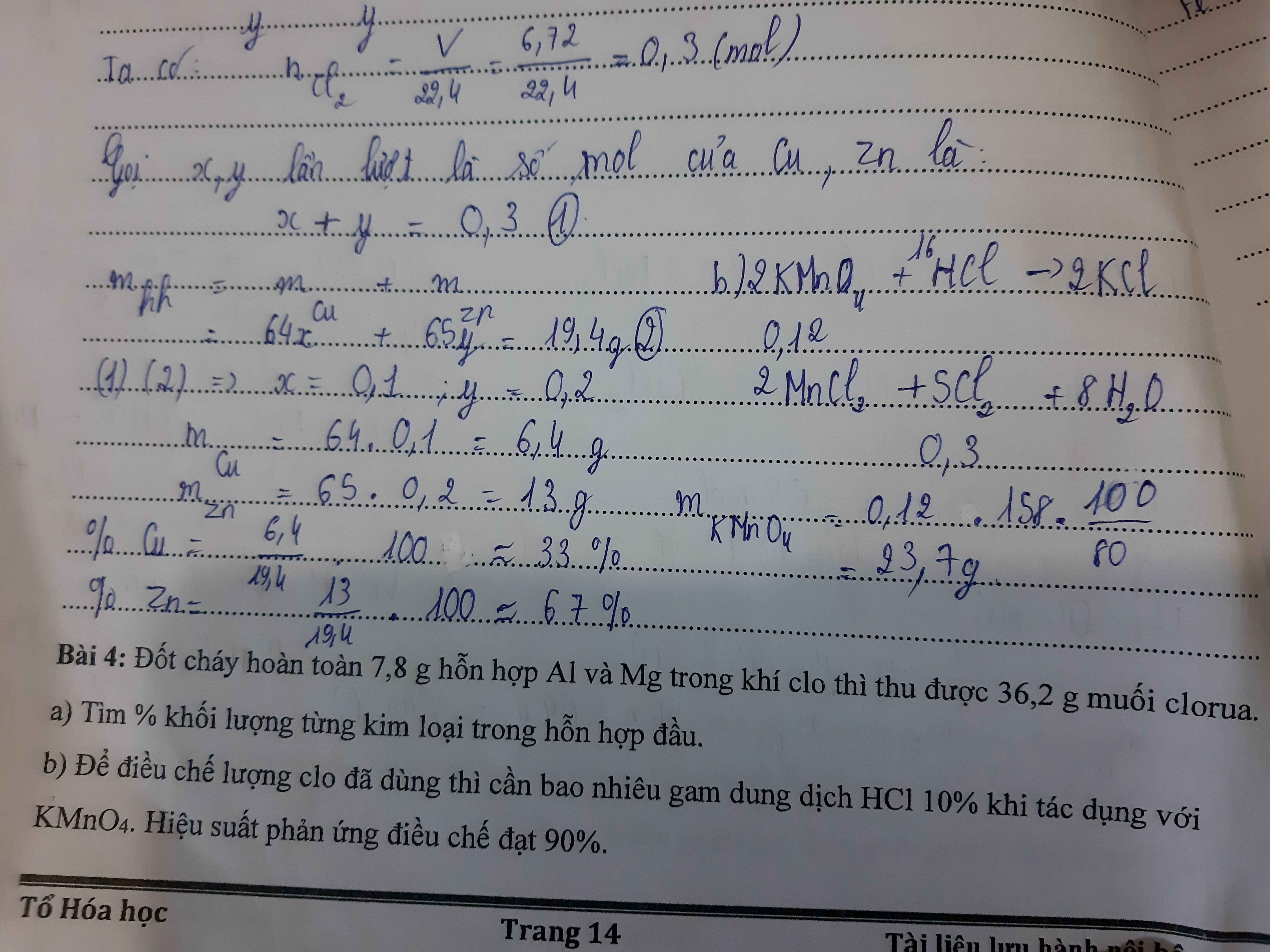
a.Theo định luật bảo toàn khối lượng, ta có:
\(m_{Cl_2}=36,2-7,8=28,4g\)
\(n_{Cl_2}=\dfrac{m}{M}=\dfrac{28,4}{71}=0,4mol\)
\(2Al+3Cl_2\rightarrow\left(t^o\right)2AlCl_3\)
2 3 2 ( mol )
\(2Mg+Cl_2\rightarrow\left(t^o\right)2MgCl_2\)
2 1 2 ( mol )
Gọi \(n_{Al}=x\)
\(n_{Mg}=y\)
\(\Rightarrow m_{Al}=27x\)
\(\Rightarrow m_{Mg}=24y\)
Ta có:
\(\left\{{}\begin{matrix}m_{hh}=27x+24y=7,8\\\dfrac{3}{2}x+\dfrac{1}{2}y=0,4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{19}{75}\\y=\dfrac{1}{25}\end{matrix}\right.\)
\(\Rightarrow m_{Al}=27.\dfrac{19}{75}=6,84g\)
\(\Rightarrow m_{Mg}=24.\dfrac{1}{25}=0,96g\)
\(\%m_{Al}=\dfrac{6,84.100}{7.8}=87,7\%\)
\(\%m_{Mg}=100\%-87,7\%=12,3\%\)
Đúng 1
Bình luận (0)
Làm dùm e bài 7 với ạ
ĐKXĐ: \(x\ge4\)
\(\sqrt{x+11}\ge\sqrt{x-4}+\sqrt{2x-1}\)
\(\Leftrightarrow x+11\ge3x-5+2\sqrt{\left(x-4\right)\left(2x-1\right)}\)
\(\Leftrightarrow8-x\ge\sqrt{2x^2-9x+4}\)
\(\Leftrightarrow\left\{{}\begin{matrix}8-x\ge0\\\left(8-x\right)^2\ge2x^2-9x+4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\le8\\x^2+7x-60\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\le8\\-12\le x\le5\end{matrix}\right.\) \(\Rightarrow-12\le x\le5\)
Kết hợp ĐKXD ta được nghiệm của BPT là: \(4\le x\le5\)
Đúng 2
Bình luận (1)
Làm dùm e bài 27 với ạ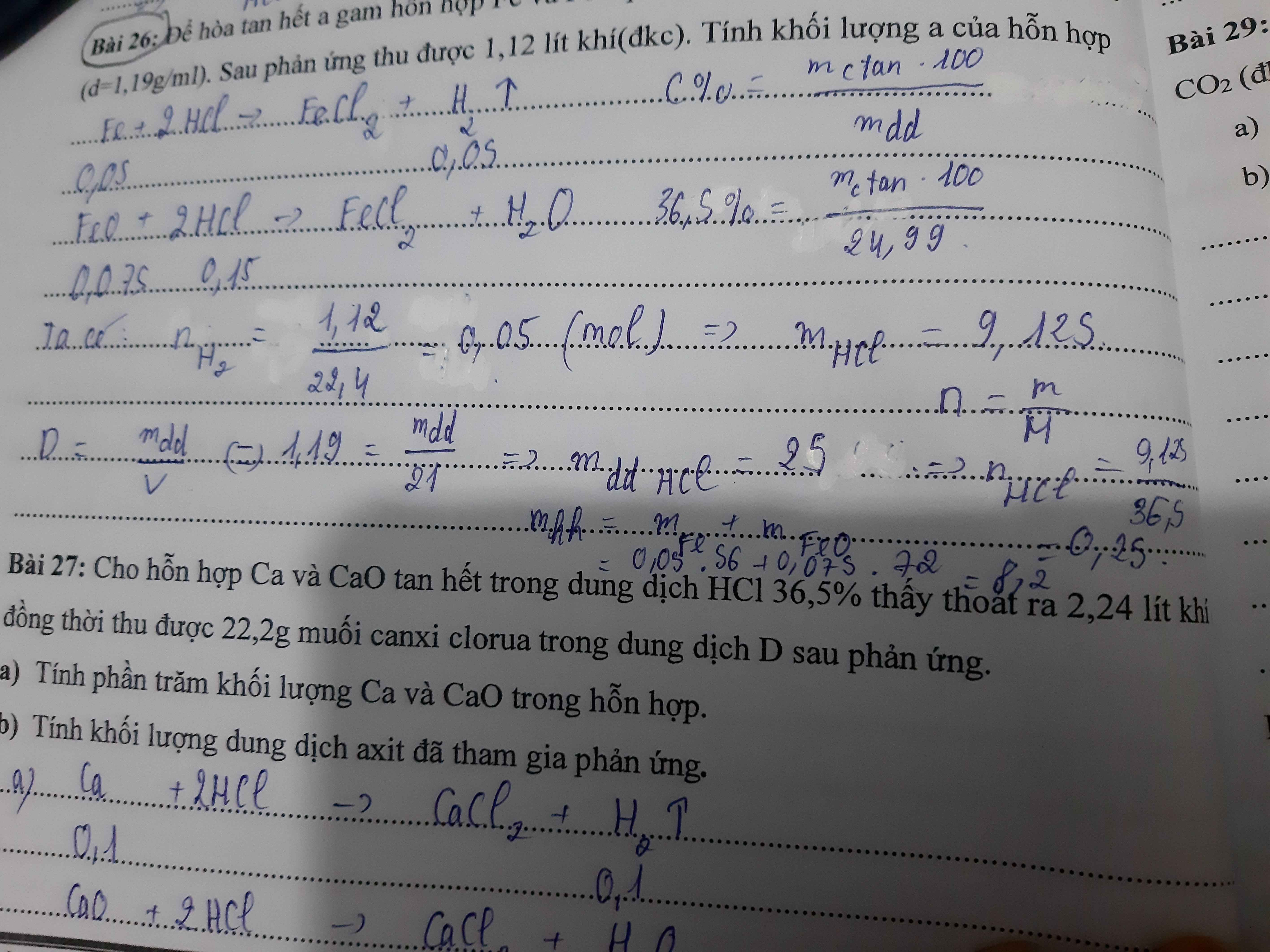
\(a,Ca+2HCl\rightarrow CaCl_2+H_2\left(1\right)\\ CaO+2HCl\rightarrow CaCl_2+H_2O\left(2\right)\\ Đặt:n_{Ca}=a\left(mol\right);n_{CaO}=b\left(mol\right)\\ \Rightarrow\left\{{}\begin{matrix}111a+111b=22,2\\22,4a=2,24\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=0,1\\b=0,1\end{matrix}\right.\\ \Rightarrow\%m_{CaO}=\dfrac{0,1.56}{0,1.56+0,1.40}.100\approx52,83\%\\ \Rightarrow\%m_{Ca}=47,17\%\\ b,n_{HCl}=2.\left(a+b\right)=0,4\left(mol\right)\\ \Rightarrow m_{ddHCl}=\dfrac{0,4.36,5.100}{36,5}=40\left(g\right)\)
Đúng 3
Bình luận (0)
Làm dùm e bài này với ạ
Bài 30:
\(Đặt:n_{KBr}=a\left(mol\right);n_{NaCl}=b\left(mol\right)\left(a,b>0\right)\\ KBr+AgNO_3\rightarrow AgBr\downarrow+KNO_3\\ NaCl+AgNO_3\rightarrow AgCl\downarrow+NaNO_3\\ \Rightarrow\left\{{}\begin{matrix}119a+58,5b=23,6\\188a+143,5b=47,5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=0,1\\b=0,2\end{matrix}\right.\\ a,m_{KBr}=0,1.119=11,9\left(g\right)\\ m_{NaCl}=0,2.58,5=11,7\left(g\right)\\ b,n_{AgNO_3}=a+b=0,3\left(mol\right)\\ \Rightarrow V_{ddAgNO_3}=\dfrac{0,3}{0,5}=0,6\left(l\right)=600\left(ml\right)\)
Đúng 2
Bình luận (0)
Làm dùm e câu 5 6 ạ 
Bài 5:
Vì \(ƯCLN\left(24,108\right)=12\) nên có thể chia nhiều nhất thành 12 tổ
Bài 6:
Vì \(BC\left(12,15,18\right)=B\left(180\right)=\left\{0;180;360;540;...\right\}\) nên khối 6 có 360 hs
Đúng 1
Bình luận (0)
Bài 6:
Gọi số học sinh là x
Theo đề, ta có: \(x\in BC\left(12;15;18\right)\)
mà 300<=x<=400
nên x=360
Đúng 1
Bình luận (0)
Hoàn thành câu 1 dùm e với ạ.
CÁC CHUYÊN GIA HÓA HỌC GIÚP E BÀI NÀY VỚI Ạ.