Cho góc nhọn x .Biết sin x = Tính cosx;tanx ;cotx

Những câu hỏi liên quan
Góc nhọn x có tanx = 3/5
Tính N = (sinx . cosx)/(sin2x - cos2x)
\(\tan x=\frac{\sin x}{\cos x}=\frac{3}{5}\Rightarrow\sin x=\frac{3}{5}\cos x\)
\(\Rightarrow N=\frac{\sin x.\cos x}{\sin^2x-\cos^2x}=\frac{\sin x.\cos x}{\left(\sin x-\cos x\right)\left(\sin x+\cos x\right)}\)
\(=\frac{\frac{3}{5}.\cos^2x}{\left(\frac{3}{5}\cos x-\cos x\right)\left(\frac{3}{5}\cos x+\cos x\right)}=\frac{\frac{3}{5}\cos^2x}{\frac{-16}{25}.\cos^2x}=\frac{-15}{16}\)
Đúng 0
Bình luận (0)
Dùng bảng lượng giác hoặc máy tính bỏ túi để tìm số đo của góc nhọn x (làm tròn đến phút), biết rằng:
a) sin x = 0,2368 ; b) cosx = 0,6224
c) tgx = 2,154 ; d) cotgx = 3,251
a) Dùng bảng lượng giác sinx = 0,2368 => x ≈ 13o42'
- Cách nhấn máy tính:

b) x ≈ 51o31'
- Cách nhấn máy tính:

c) x ≈ 65o6'
- Cách nhấn máy tính:

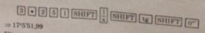
d) x ≈ 17o6'
- Cách nhấn máy tính:
Đúng 0
Bình luận (0)
Cho góc nhọn x . Biết sinx = 0,6 . Tính cosx
$\sin x=0,6\\\Leftrightarrow \sin^2 x=0,36\\\Rightarrow \cos^2 x=0,64\\\Leftrightarrow \cos x=0,8(x>0)$
Đúng 0
Bình luận (2)
Tính số đo góc nhọn .Biết tan x sin x = 3 sin (90°-x)
cho góc nhọn x biết
1. sinx=1/4 tính cosx. 2. tgx= 1/3, tính sinx.
mọi người giúp mình với ạ
1.
\(sin^2x+cos^2x=1\Rightarrow\left(\dfrac{1}{4}\right)^2+cos^2x=1\)
\(\Rightarrow cos^2x=\dfrac{15}{16}\Rightarrow cosx=\dfrac{\sqrt{15}}{4}\)
2.
\(tanx=\dfrac{1}{3}\Rightarrow tan^2x=\dfrac{1}{9}\Rightarrow\dfrac{sin^2x}{cos^2x}=\dfrac{1}{9}\)
\(\Rightarrow\dfrac{sin^2x}{1-sin^2x}=\dfrac{1}{9}\Rightarrow9sin^2x=1-sin^2x\)
\(\Rightarrow sin^2x=\dfrac{1}{10}\Rightarrow sinx=\dfrac{\sqrt{10}}{10}\)
Đúng 0
Bình luận (0)
1.tính cos a, tan a, cot a nếu biết a nhọn và sin a =3/5
2. tính sin x, cos x nếu biết x nhọn và tan x=12/35
3. cho góc a nhọn và cos a =5/13.tính sin a, tan a và cot a
giúp mình với gấp lắm rồi mình sẽ tick cho bạn nào giải được. cảm ơn trước nhé
Tính số đo góc x biết:
a) 5. sin.(\(90^o-x\)) -3.cosx=1,5
Cho góc nhọn x , biết cos x =\(\frac{\sqrt{2}}{2}\) . Tính sin x , cot x , tan x
Cho góc nhọn x có tgx= 3, tính sinx, cosx mong mn giúp mình
ĐK: \(x\ne\dfrac{\pi}{2}+k\pi\)
Ta có:
\(\left\{{}\begin{matrix}tanx=3\\sin^2x+cos^2x=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sinx=3cosx\\9cos^2x+cos^2x=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sinx=3cosx\\cos^2x=\dfrac{1}{10}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sinx=3cosx\\cosx=\pm\dfrac{1}{\sqrt{10}}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}sinx=\dfrac{3}{\sqrt{10}}\\cosx=\dfrac{1}{\sqrt{10}}\end{matrix}\right.\\\left\{{}\begin{matrix}sinx=-\dfrac{3}{\sqrt{10}}\\cosx=-\dfrac{1}{\sqrt{10}}\end{matrix}\right.\end{matrix}\right.\)
Đúng 0
Bình luận (0)

















