Bài 1: 

a: \(11x^2-6xy-5y^2\)

\(=11x^2-11xy+5xy-5y^2\)

\(=11x\left(x-y\right)+5y\left(x-y\right)\)

\(=\left(x-y\right)\left(11x+5y\right)\)

b: \(4x^3-16x^2+19x-6\)

\(=4x^3-8x^2-8x^2+16x+3x-6\)

\(=\left(x-2\right)\left(4x^2-8x+3\right)\)

\(=\left(x-2\right)\left(2x-1\right)\left(2x-3\right)\)

Bài 1: 

a: \(11x^2-6xy-5y^2\)

\(=11x^2-11xy+5xy-5y^2\)

\(=11x\left(x-y\right)+5y\left(x-y\right)\)

\(=\left(x-y\right)\left(11x+5y\right)\)

b: \(4x^3-16x^2+19x-6\)

\(=4x^3-8x^2-8x^2+16x+3x-6\)

\(=\left(x-2\right)\left(4x^2-8x+3\right)\)

\(=\left(x-2\right)\left(2x-3\right)\left(2x-1\right)\)

\(a,=11x^2-11xy+5xy-5y^2=\left(11x+5y\right)\left(x-y\right)\\ b,=4x^3-8x^2-8x^2+16x+3x-6\\ =\left(x-2\right)\left(4x^2-8x+3\right)\\ =\left(x-2\right)\left(4x^2-2x-6x+3\right)\\ =\left(x-2\right)\left(2x-1\right)\left(2x-3\right)\)

Bài 1: 

a: \(11x^2-6xy-5y^2\)

\(=11x^2-11xy+5xy-5y^2\)

\(=11x\left(x-y\right)+5y\left(x-y\right)\)

\(=\left(x-y\right)\left(11x+5y\right)\)

b: \(4x^3-16x^2+19x-6\)

\(=4x^3-8x^2-8x^2+16x+3x-6\)

\(=\left(x-2\right)\left(4x^2-8x+3\right)\)

\(=\left(x-2\right)\left(2x-1\right)\left(2x-3\right)\)

a) \(4x^2+16x+3=0\)

\(\Delta'=84-12=72\Rightarrow\sqrt[]{\Delta'}=6\sqrt[]{2}\)

Phương trình có 2 nghiệm

\(\left[{}\begin{matrix}x=\dfrac{-8+6\sqrt[]{2}}{4}\\x=\dfrac{-8-6\sqrt[]{2}}{4}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-2\left(4-3\sqrt[]{2}\right)}{4}\\x=\dfrac{-2\left(4+3\sqrt[]{2}\right)}{4}\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-\left(4-3\sqrt[]{2}\right)}{2}\\x=\dfrac{-\left(4+3\sqrt[]{2}\right)}{2}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3\sqrt[]{2}-4}{2}\\x=\dfrac{-3\sqrt[]{2}-4}{2}\end{matrix}\right.\)

b) \(7x^2+16x+2=1+3x^2\)

\(4x^2+16x+1=0\)

\(\Delta'=84-4=80\Rightarrow\sqrt[]{\Delta'}=4\sqrt[]{5}\)

Phương trình có 2 nghiệm

\(\left[{}\begin{matrix}x=\dfrac{-8+4\sqrt[]{5}}{4}\\x=\dfrac{-8-4\sqrt[]{5}}{4}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-4\left(2-\sqrt[]{5}\right)}{4}\\x=\dfrac{-4\left(2+\sqrt[]{5}\right)}{4}\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-\left(2-\sqrt[]{5}\right)\\x=-\left(2+\sqrt[]{5}\right)\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=-2+\sqrt[]{5}\\x=-2-\sqrt[]{5}\end{matrix}\right.\)

c) \(4x^2+20x+4=0\)

\(\Leftrightarrow4\left(x^2+5x+1\right)=0\)

\(\Leftrightarrow x^2+5x+1=0\)

\(\Delta=25-4=21\Rightarrow\sqrt[]{\Delta}=\sqrt[]{21}\)

Phương trình có 2 nghiệm

\(\left[{}\begin{matrix}x=\dfrac{-5+\sqrt[]{21}}{2}\\x=\dfrac{-5-\sqrt[]{21}}{2}\end{matrix}\right.\)

e: ta có: \(4x^2+4x-6=2\)

\(\Leftrightarrow4x^2+4x-8=0\)

\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)

f: Ta có: \(2x^2+7x+3=0\)

\(\Leftrightarrow\left(x+3\right)\left(2x+1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=-\dfrac{1}{2}\end{matrix}\right.\)

a) Xét tam thức \(f\left( x \right) = 7{x^2} - 19x - 6\) có \(\Delta  = 529 > 0\), có hai nghiệm phân biệt \({x_1} =  - \frac{2}{7},{x_2} = 3\) và có \(a = 7 > 0\)

Ta có bảng xét dấu như sau

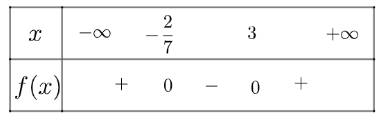
Vậy nghiệm của bất phương trình là đoạn \(\left[ { - \frac{2}{7};3} \right]\)

b) \( - 6{x^2} + 11x > 10 \Leftrightarrow  - 6{x^2} + 11x - 10 > 0\)

Xét tam thức \(f\left( x \right) =  - 6{x^2} + 11x - 10\) có \(\Delta  =  - 119 < 0\)và có \(a =  - 6 < 0\)

Ta có bảng xét dấu như sau

Vậy bất phương trình vô nghiệm

c) \(3{x^2} - 4x + 7 > {x^2} + 2x + 1 \Leftrightarrow 2{x^2} - 6x + 6 > 0\)

Xét tam thức \(f\left( x \right) = 2{x^2} - 6x + 6\) có \(\Delta  =  - 12 < 0\)và có \(a = 2 > 0\)

Ta có bảng xét dấu như sau

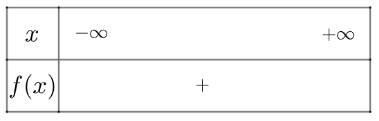
Vậy bất phương trình có vô số nghiệm

d) Xét tam thức \(f\left( x \right) = {x^2} - 10x + 25\) có \(\Delta  = 0\), có nghiệm kép \({x_1} = {x_2} = 5\) và có \(a = 1 > 0\)

Ta có bảng xét dấu như sau

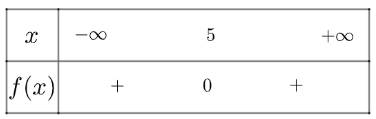
Vậy nghiệm của bất phương trình là \(x = 5\)

a, 5x - 7(3 - x) = 3

=> 5x - 21 + 7x = 3

=> 12x = 24

=> x = 2

b, 4x² + 3x = 0

=> x(4x + 3) = 0 

=> \(\orbr{\begin{cases}x=0\\4x+3=0\end{cases}}\)

=> \(\orbr{\begin{cases}x=0\\x=\frac{-3}{4}\end{cases}}\)

c, (x + 1)² - 4x² =0

=> (x + 1)² - (2x)² = 0

=> (x + 1 - 2x)(x + 1 + 2x) = 0

=> (1 - x)(3x+ 1) = 0

=> \(\orbr{\begin{cases}1-x=0\\3x+1=0\end{cases}}\)

=> \(\orbr{\begin{cases}x=1\\x=\frac{-1}{3}\end{cases}}\)

d, x³ - 19x - 30 = 0

=> x³ - 5x² + 5x² - 25x + 6x - 30 = 0

=> x²(x - 5) + 5x(x - 5) + 6(x - 5) = 0

=> (x² + 5x + 6)(x - 5) = 0

=> (x² + 2x + 3x + 6)(x - 5) = 0

=> (x + 2)(x + 3)(x - 5) = 0

=> x + 2 = 0 hoặc x + 3 = 0 hoặc x - 5 = 0

=> x = -2 hoặc x = -3 hoặc x = 5

=> x thuộc {-2; -3; 5}

a, x=2

b, x=0

c, x=1

d, x=2

kb nha

Tìm x, biết 

a) 5x-7(3-x)=3 nghi đề sai

b) 4x²+3x=0 \(\Leftrightarrow x\left(4x+3\right)=0=>\orbr{\begin{cases}x=0\\x=-\frac{3}{4}\end{cases}}\)

c) (x+1)²-4x²=0\(\Leftrightarrow\left(x+1\right)^2-\left(2x\right)^2\Leftrightarrow\orbr{\begin{cases}x+1-2x=0\Rightarrow x=1\\x+1+2x=0\Rightarrow x=-\frac{1}{3}\end{cases}}\)

d) x³-19x-30=0 nghi đe sai lớp 8 khó thế

Tích mình đi rồi mình nói thề bạn