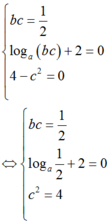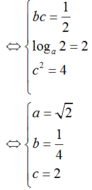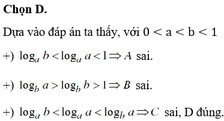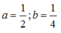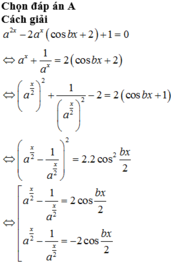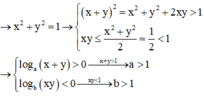1, cho a,b là số thực thỏa 0<a<1 ; 0<b<1 ; a khác b ; và \(a-b=\sqrt{1-b^2}-\sqrt{1-a^2}\)
tính giá trị biểu thức Q= \(\sqrt{a^2+b^2}+2019\)
2 Tìm nghiệm nguyen pt \(x^2-4xy+5y^2=2\left(x-y\right)\)
3. cho a,b,c>0 chứng minh \(\frac{bc}{a^2\left(b+c\right)}+\frac{ca}{b^2\left(c+a\right)}+\frac{ab}{c^2\left(a+b\right)}\ge\frac{1}{2a}+\frac{1}{2b}+\frac{1}{2c}\)