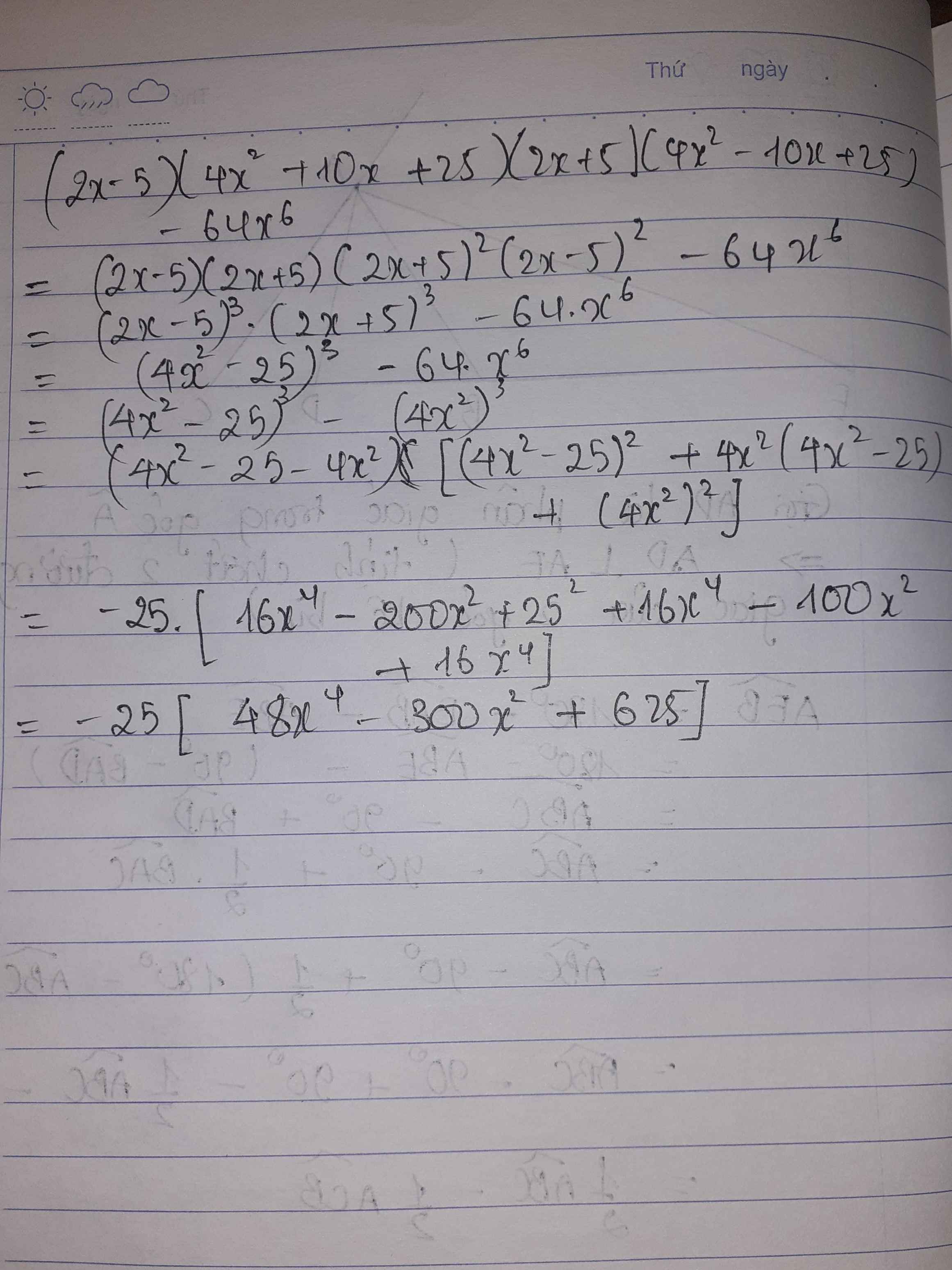\(4x^2-3\left(2x-5\right)-25=0\Leftrightarrow4x^2-6x-10=0\)

\(\Leftrightarrow2\left(2x^2-3x-5\right)=0\Leftrightarrow2\left(x+1\right)\left(2x-5\right)=0\Leftrightarrow x=-1;x=\dfrac{5}{2}\)

4x²-3(2x-5)-25=0

⇒(2x)²-5²-3(2x-5)=0

⇒(2x-5)(2x+5)-3(2x-5)=0

⇒(2x-5)(2x+2)=0

⇒hoặc 2x-5=0⇒x=2,5

hoặc 2x+2=0⇒x=-1

vậy x={2,5;-1}

Khi k = 0 ta có phương trình: 4 x 2 - 25 = 0

⇔ (2x + 5)(2x – 5) = 0

⇔ 2x + 5 = 0 hoặc 2x – 5 = 0

2x + 5 = 0 ⇔ x = - 5/2

2x – 5 = 0 ⇔ x = 5/2

Vậy phương trình có nghiệm x = - 5/2 hoặc x = 5/2

g) \(4x^2-25-\left(2x-5\right)\left(2x+7\right)=0\)

  \(\Rightarrow\left(2x-5\right)\left(2x+5\right)-\left(2x-5\right)\left(2x+7\right)=0\)

  \(\Rightarrow\left(2x-5\right)\left(2x+5-2x-7\right)=0\)

  \(\Rightarrow-2\left(2x-5\right)=0\Rightarrow x=\dfrac{5}{2}\)

i) \(x^3+27+\left(x+3\right)\left(x-9\right)=0\)

  \(\Rightarrow\left(x+3\right)\left(x^2-3x+9\right)+\left(x+3\right)\left(x-9\right)=0\)

  \(\Rightarrow\left(x+3\right)\left(x^2-3x+9+x-9\right)=0\)

  \(\Rightarrow\left(x+3\right)\left(x^2-2x\right)=0\Rightarrow x\left(x+3\right)\left(x-2\right)=0\)

  \(\Rightarrow\left[{}\begin{matrix}x=0\\x=-3\\x=2\end{matrix}\right.\)

a,Thay k=0 vào pt ta có:
\(4x^2-25+0^2+4.0.x=0\\ \Leftrightarrow4x^2-25=0\\ \Leftrightarrow x^2=\dfrac{25}{4}\\ \Leftrightarrow x=\pm\dfrac{5}{2}\)

b, Thay x=-2 vào pt ta có:
\(4.\left(-2\right)^2-25+k^2+4k.\left(-2\right)=0\\ \Leftrightarrow4.4-25+k^2-8k=0\\ \Leftrightarrow k^2-8k+16-25=0\\ \Leftrightarrow k^2-8k-9=0\\ \Leftrightarrow\left(k^2+k\right)-\left(9k+9\right)=0\\ \Leftrightarrow k\left(k+1\right)-9\left(k+1\right)=0\\ \Leftrightarrow\left(k+1\right)\left(k-9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}k=-1\\k=9\end{matrix}\right.\)

Khi k = - 3 ta có phương trình: 4 x 2  – 25 + - 3 2  + 4(-3)x = 0

⇔ 4 x 2  – 25 + 9 – 12x = 0

⇔ 4 x 2  – 12x – 16 = 0

⇔  x 2  – 3x – 4 = 0

⇔  x 2  – 4x + x – 4 = 0

⇔ x(x – 4) + (x – 4) = 0

⇔ (x + 1)(x – 4) = 0

⇔ x + 1 = 0 hoặc x – 4 = 0

x + 1 = 0 ⇔ x = -1

x – 4 = 0 ⇔ x = 4

Vậy phương trình có nghiệm x = -1 hoặc x = 4.

a) \(\text{5x(x-2)+(2-x)=0}\)

\(\Rightarrow5x\left(x-2\right)-\left(x-2\right)=0\\ \Rightarrow\left(x-2\right)\left(5x-1\right)=0\\ \Rightarrow\left[{}\begin{matrix}x-2=0\\5x-1=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{1}{5}\end{matrix}\right.\)

b) \(\text{x(2x-5)-10x+25=0}\)

\(\Rightarrow x\left(2x-5\right)-5\left(2x-5\right)=0\\ \Rightarrow\left(x-5\right)\left(2x-5\right)=0\\ \Rightarrow\left[{}\begin{matrix}x-5=0\\2x-5=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=5\\x=2,5\end{matrix}\right.\)

c) \(\dfrac{25}{16}-4x^2+4x-1=0\)

\(\Rightarrow\dfrac{9}{16}-4x^2+4x=0\)

\(\Rightarrow-4x^2+4x+\dfrac{9}{16}=0\)

\(\Rightarrow-4x^2-\dfrac{1}{2}x+\dfrac{9}{2}x+\dfrac{9}{16}=0\)

\(\Rightarrow\left(-4x^2-\dfrac{1}{2}x\right)+\left(\dfrac{9}{2}x+\dfrac{9}{16}\right)=0\)

\(\Rightarrow-\dfrac{1}{2}x\left(8x+1\right)+\dfrac{9}{16}\left(8x+1\right)=0\)

\(\Rightarrow\left(-\dfrac{1}{2}x+\dfrac{9}{16}\right)\left(8x+1\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}-\dfrac{1}{2}x+\dfrac{9}{16}=0\\8x+1=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{9}{8}\\x=\dfrac{-1}{8}\end{matrix}\right.\)

a) \(5x\left(x-2\right)+\left(2-x\right)=0\)

\(\Rightarrow5x\left(x-2\right)-\left(x-2\right)=0\)

\(\Rightarrow\left(x-2\right)\left(5x-1\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x-2=0\\5x-1=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{1}{5}\end{matrix}\right.\)

b) \(x\left(2x-5\right)-10x+25=0\)

\(\Rightarrow x\left(2x-5\right)-5\left(2x-5\right)=0\)

\(\Rightarrow\left(x-5\right)\left(2x-5\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x-5=0\\2x-5=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{5}{2}\end{matrix}\right.\)

c) \(\dfrac{25}{16}-4x^2+4x-1=0\)

\(\Rightarrow-4x^2+4x+\dfrac{9}{16}=0\)

\(\Rightarrow\left(x-\dfrac{9}{8}\right)\left(x+\dfrac{1}{8}\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x-\dfrac{9}{8}=0\\x+\dfrac{1}{8}=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\dfrac{9}{8}\\x=-\dfrac{1}{8}\end{matrix}\right.\)

d) \(x^4+2x^2-8=0\)

\(\Rightarrow\left(x^4+2x^2+1\right)-9=0\)

\(\Rightarrow\left(x^2+1\right)^2-3^2=0\)

\(\Rightarrow\left(x^2+1-3\right)\left(x^2+1+3\right)=0\)

\(\Rightarrow\left(x^2-2\right)\left(x^2+4\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x^2-2=0\\x^2+4=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x^2=2\\x^2=-4\end{matrix}\right.\) \(\Rightarrow x^2=2\) \(\Rightarrow x=\pm\sqrt{2}\)

a ) 28 + x = − 59 x = − 59 − 28 x = − 87

b ) x − 4 x 2 − 25 = 0 ⇔ x − 4 = 0 x 2 − 25 = 0 ⇔ x = 4 x 2 = 25 ⇔ x = 4 x = ± 5

x + 2 = 17 ⇒ x + 2 = 17 x + 2 = − 17 ⇒ x = 15 x = − 19

d ) − 19 + 4 ( 2 − x ) = 25 − x − 19 + 8 − 4 x = 25 − x 4 x − x = − 19 + 8 − 25 3 x = − 36 = > x = − 12  

Ta có: \(\left(2x-5\right)\left(4x^2+10x+25\right)\left(2x+5\right)\left(4x^2-10x+25\right)-64x^6\)

\(=\left(8x^3-125\right)\left(8x^3+125\right)-64x^6\)

\(=64x^6-15625-64x^6\)

=-15625

a) \(\left|4x^2-25\right|=0\)

\(\Leftrightarrow4x^2-25=0\)

\(\Leftrightarrow\left(2x+5\right)\left(2x-5\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}2x-5=0\\2x+5=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=\frac{5}{2}\\x=-\frac{5}{2}\end{cases}}}\)

a) \(7x\left(2x-3\right)-\left(4x^2-9\right)=0\Rightarrow7x\left(2x-3\right)-\left(2x-3\right)\left(2x+3\right)=0\Rightarrow\left(2x-3\right)\left(7x-2x+3\right)=0\Rightarrow\left[{}\begin{matrix}2x-3=0\\5x+3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=-\dfrac{3}{5}\end{matrix}\right.\)

b) \(\left(2x-7\right).\left(x-2\right)\left(x^2-4\right)=0\Rightarrow\left(2x-7\right)\left(x-2\right)^2\left(x+2\right)=0\Rightarrow\left[{}\begin{matrix}2x-7=0\\\left(x-2\right)^2=0\\x+2=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\\x=-2\end{matrix}\right.\)

c)\(\left(9x^2-25\right)-\left(6x-10\right)=0\Rightarrow\left(3x-5\right)\left(3x+5\right)-2\left(3x-5\right)=0\Rightarrow\left(3x-5\right)\left(3x+5-2\right)=0\Rightarrow\left[{}\begin{matrix}3x-5=0\\3x+3=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=\dfrac{5}{3}\\x=1\end{matrix}\right.\)

a: Ta có: \(7x\left(2x-3\right)-\left(4x^2-9\right)=0\)

\(\Leftrightarrow7x\left(2x-3\right)-\left(2x-3\right)\left(2x+3\right)=0\)

\(\Leftrightarrow\left(2x-3\right)\left(5x-3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=\dfrac{3}{5}\end{matrix}\right.\)

b: Ta có: \(\left(2x-7\right)\left(x-2\right)\left(x^2-4\right)=0\)

\(\Leftrightarrow\left(2x-7\right)\left(x-2\right)^2\cdot\left(x+2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\\x=-2\end{matrix}\right.\)

c: Ta có: \(\left(9x^2-25\right)-\left(6x-10\right)=0\)

\(\Leftrightarrow\left(3x-5\right)\left(3x+5-2\right)=0\)

\(\Leftrightarrow\left(3x-5\right)\left(3x+3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{3}\\x=-1\end{matrix}\right.\)