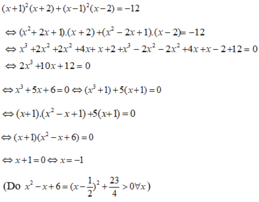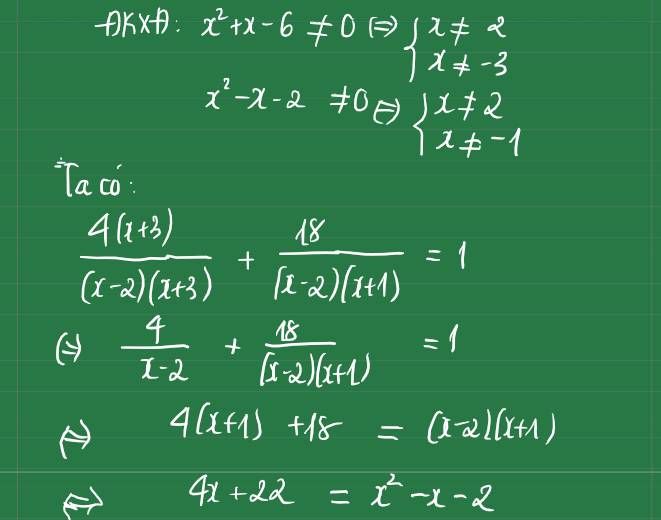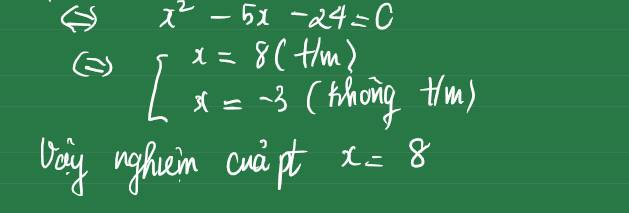Ta có

Vậy phương trình có nghiệm x = - 1

minh biet

ta có : 

\(\left|x+1\right|+\left|x-1\right|=1+\left|\left(x-1\right)\left(x+1\right)\right|\)

\(\Leftrightarrow\left|x-1\right|\left|x+1\right|-\left|x-1\right|-\left|x+1\right|+1=0\)

\(\Leftrightarrow\left(\left|x-1\right|-1\right)\left(\left|x+1\right|-1\right)=0\Leftrightarrow\orbr{\begin{cases}\left|x-1\right|=1\\\left|x+1\right|=1\end{cases}}\)

\(\Leftrightarrow x\in\left\{-2,0,2\right\}\)

ĐKXĐ: \(x\ne\pm2\)

\(\dfrac{x+1}{x-2}-\dfrac{5}{x+2}=\dfrac{12}{x^2-4}+1\\ \Leftrightarrow\dfrac{\left(x+1\right)\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}-\dfrac{5\left(x-2\right)}{\left(x+2\right)\left(x-2\right)}=\dfrac{12}{\left(x+2\right)\left(x-2\right)}+\dfrac{\left(x+2\right)\left(x-2\right)}{\left(x+2\right)\left(x-2\right)}\\ \Leftrightarrow\left(x+1\right)\left(x+2\right)-5\left(x-2\right)=12+\left(x+2\right)\left(x-2\right)\\ \Leftrightarrow x^2+x+2x+2-5x+10=12+x^2-4\\ \Leftrightarrow-2x=-4\\ \Leftrightarrow x=2\left(ktm\right)\)

Vậy \(S\in\left\{\varnothing\right\}\)

ĐKXĐ: \(\begin{cases}x-2\ne 0\\x+2\ne 0\end{cases}\leftrightarrow x\ne 2\\x\ne -2\end{cases}\)

\(\dfrac{x+1}{x-2}-\dfrac{5}{x+2}=\dfrac{12}{x^2-4}+1\)

\(\leftrightarrow \dfrac{(x+1)(x+2)}{(x-2)(x+2)}-\dfrac{5(x-2)}{(x+2)(x-2)}=\dfrac{12}{(x-2)(x+2)}+\dfrac{(x-2)(x+2)}{(x-2)(x+2)}\)

\(\to x^2+3x+2-5x+10=12+x^2-4\)

\(\leftrightarrow x^2-2x-x^2=12-12-4\)

\(\leftrightarrow -2x=-4\)

\(\leftrightarrow x=2(\rm KTM)\)

Vậy pt đã cho vô nghiệm \(S=\varnothing\)

1/\(\sqrt{x-4}-\sqrt{1-x}=1\)

Để Pt dc xác định

Thì\(\left\{{}\begin{matrix}x-4\ge0\\1-x\ge0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ge4\\x\le1\end{matrix}\right.\)

Vì xét trên trục số ta thấy nó loại nhau

Nên Pt này vô nghiệm

1)ĐKXĐ: \(-4\le x\le1\)

\(\sqrt{x+4}-\sqrt{1-x}=1\\ \Rightarrow\sqrt{x+4}=\sqrt{1-x}+1\\ \Rightarrow x+4=1-x+2\sqrt{1-x}+1\\ \Rightarrow2x+2=2\sqrt{1-x}\\ \Rightarrow x+1=\sqrt{1-x}\\ \Rightarrow x^2+2x+1=1-x\\ \Rightarrow x^2+3x=0\\ \Rightarrow x\left(x+3\right)=0\\ \Rightarrow x=-3\)

Vậy x = -3

2)ĐKXĐ: \(-\sqrt{10}\le x\le\sqrt{10}\)

Với x = -3 thì:

0=0(luôn đúng)

Với x khác -3 thì:

\(\left(x+3\right)\sqrt{10-x^2}=x^2-x+12\\ \Rightarrow\left(x+3\right)\sqrt{10-x^2}=\left(x+3\right)\left(x-4\right)\\ \Rightarrow\sqrt{10-x^2}=x-4\\ \Rightarrow10-x^2=x^2-8x+16\\ \Rightarrow2x^2-8x+6=0\\ \Rightarrow x^2-4x+3=0\\ \Rightarrow\left(x-1\right)\left(x-3\right)=0\\ \Rightarrow x\in\left\{1;3\right\}\)

Vậy x\(\in\left\{-3;1;3\right\}\)

khó ệ!thằng nào ngu người có khi làm được
 

khong ke nao lam duoc

mk mới hc lớp 5 thui

<=> 12 - 2(x^2-2x+1) = 4x-8 - 2x^2 +11x-15

<=> 10 - 2x^2 + 4x = -2x^2 + 15x -23 

<=> -11x + 33 =0 <=> x = 3 

\(\Leftrightarrow12-2\left(x^2-2x+1\right)-4x+8+\left(x-3\right)\left(2x-5\right)=0\)

\(\Leftrightarrow-4x+20-2x^2+4x-2+2x^2-5x-6x+15=0\)

=>-11x+33=0

hay x=3

\(\Leftrightarrow\)12-2(x²-2x+1)=2x-8-(2x²-5x-6x+15)
\(\Leftrightarrow\)12-2x²+2x-1=2x-8-2x²+5x+6x-15
\(\Leftrightarrow\)-2x²+2x²+2x-2x-5x-6x=-12+1-8-15
\(\Leftrightarrow\)-11x=-34
\(\Leftrightarrow\)x=\(\dfrac{34}{11}\)
Vậy ...
 

a) Ta có: \(\left(x-1\right)^2+2=x^2+3x\)

\(\Leftrightarrow x^2-2x+1+2-x^2-3x=0\)

\(\Leftrightarrow-5x=-3\)

hay \(x=\dfrac{3}{5}\)

câu b sai đề bn

câu c tìm gì

\(\frac{\left(x-2\right)^2}{12}-\frac{\left(x+1\right)^2}{21}=\frac{\left(x-4\right)\left(x-6\right)}{28}\)

\(\Leftrightarrow\frac{7\left(x-2\right)^2}{84}-\frac{4\left(x+1\right)^2}{84}=\frac{3\left(x-4\right)\left(x-6\right)}{84}\)

\(\Leftrightarrow7\left(x-2\right)^2-4\left(x+1\right)^2=3\left(x-4\right)\left(x-6\right)\)

\(\Leftrightarrow7\left(x^2-4x+4\right)-4\left(x^2+2x+1\right)=3\left(x^2-10x+24\right)\)

\(\Leftrightarrow7x^2-28x+28-4x^2-8x-4=3x^2-30x+72\)

\(\Leftrightarrow7x^2-4x^2-3x^2-28x-8x+30+28-4-72=0\)

\(\Leftrightarrow-6x-48=0\)

\(\Leftrightarrow-6x=48\)

\(\Leftrightarrow x=-8\)

Vậy tập nghiệm của PT là : \(S=\left\{-8\right\}\)

Chúc bạn học tốt !!!

\(\(\frac{\left(x-2\right)^2}{12}-\frac{\left(x+1\right)^2}{21}=\frac{\left(x-4\right)\left(x-6\right)}{28}\)\)

\(\Leftrightarrow\frac{7\left(x^2-4x+4\right)}{84}-\frac{4\left(x^2+2x+1\right)}{84}=\frac{3\left(x^2-6x-4x+24\right)}{84}\)

\(\Rightarrow7x^2-28x+28-4x^2-8x-4=3x^2-30x+\frac{72}{ }\)

\(\Leftrightarrow3x^2-36x+24=3x^2-30x+72\)

\(\Leftrightarrow-6x=48\Leftrightarrow x=-8\)