Cho \(\overrightarrow{a}\) = (1; 2), \(\overrightarrow{b}\)= (-1; -3). Tính \(\left(\overrightarrow{a},\overrightarrow{b}\right)\)

Những câu hỏi liên quan
Cho ba véc-tơ overrightarrow{a},overrightarrow{b},overrightarrow{c}thỏa mãn:|overrightarrow{a}| 4, |overrightarrow{b} |1, |overrightarrow{c}| 5 và 5(overrightarrow{b}-overrightarrow{a} ) + 3overrightarrow{c}overrightarrow{0}.Khi đó biểu thức M overrightarrow{a}.overrightarrow{b}+overrightarrow{b}.overrightarrow{c}+overrightarrow{c}.overrightarrow{a} có giá trị là bao nhiêu
Đọc tiếp
Cho ba véc-tơ \(\overrightarrow{a},\overrightarrow{b},\overrightarrow{c}\)thỏa mãn:
|\(\overrightarrow{a}\)| = 4, |\(\overrightarrow{b}\) |=1, |\(\overrightarrow{c}\)| = 5 và 5(\(\overrightarrow{b}-\overrightarrow{a}\) ) + 3\(\overrightarrow{c}\)=\(\overrightarrow{0}\).
Khi đó biểu thức M = \(\overrightarrow{a}.\overrightarrow{b}+\overrightarrow{b}.\overrightarrow{c}+\overrightarrow{c}.\overrightarrow{a}\) có giá trị là bao nhiêu
Cho hai vecto \(\overrightarrow{u}\)=(2;a) và \(\overrightarrow{v}\)=(1;-1). Tính a để \(\overrightarrow{u}\).\(\overrightarrow{v}\)= 1
\(\overrightarrow{u}.\overrightarrow{v}=2.1+a.\left(-1\right)=2-a\)
\(\Rightarrow2-a=1\Rightarrow a=1\)
Đúng 0
Bình luận (0)
Cho hai vectơ cho hai vectơ overrightarrow a ,overrightarrow b và điểm M như hình 3.a) Hãy vẽ vectơ overrightarrow {MN} 3overrightarrow a ,overrightarrow {MP} - 3overrightarrow b b) Cho biết mỗi ô có cạnh bằng 1. Tính: left| {3overrightarrow b } right|,left| { - 3overrightarrow b } right|,left| {2overrightarrow a + 2overrightarrow b } right|.
Đọc tiếp
Cho hai vectơ cho hai vectơ \(\overrightarrow a ,\overrightarrow b \) và điểm M như hình 3.
a) Hãy vẽ vectơ \(\overrightarrow {MN} = 3\overrightarrow a ,\overrightarrow {MP} = - 3\overrightarrow b \)
b) Cho biết mỗi ô có cạnh bằng 1. Tính: \(\left| {3\overrightarrow b } \right|,\left| { - 3\overrightarrow b } \right|,\left| {2\overrightarrow a + 2\overrightarrow b } \right|\).

a) \(\overrightarrow {MN} = 3\overrightarrow a \)có độ dài bằng 3 lần vectơ \(\overrightarrow a \), cùng hướng với vectơ \(\overrightarrow a \)
Suy ra, từ điểm M vẽ vectơ MN với độ dài là 6 ô vuông và có hướng từ trái sang phải
\(\overrightarrow {MP} = - 3\overrightarrow b \)có độ dài bằng 3 lần vectơ \( - \overrightarrow b \), ngược hướng với vectơ \(\overrightarrow b \)
Suy ra, từ điểm M vẽ vectơ MP với độ dài là 3 đường chéo ô vuông và có hướng từ trên xuống dưới chếch sang trái
b) Hình vuông với cạnh bằng 1 thì ta tính được đường chéo có độ dài là \(\sqrt 2 \); \(\left| {\overrightarrow b } \right| = \sqrt 2 \) . Suy ra:
\(\left| {3\overrightarrow b } \right| = 3\left| {\overrightarrow b } \right| = 3\sqrt 2 \); \(\left| { - 3\overrightarrow b } \right| = 3\left| {\overrightarrow { - b} } \right| = 3\sqrt 2 \); \(\left| {2\overrightarrow a + 2\overrightarrow b } \right| = \left| {2\left( {\overrightarrow a + \overrightarrow b } \right)} \right| = 2\left| {\overrightarrow a + \overrightarrow b } \right|\)
Từ điểm cuối của vectơ \(\overrightarrow a \) vẽ một vectơ bằng vectơ \(\overrightarrow b \) ta có \(\overrightarrow c = \overrightarrow a + \overrightarrow b \)
Áp dụng định lý cosin ta tính được độ dài của vectơ \(\overrightarrow c \)là \(\left| {\overrightarrow c } \right| = \sqrt {{{\left| {\overrightarrow a } \right|}^2} + {{\left| {\overrightarrow b } \right|}^2} - 2\left| {\overrightarrow a } \right|\left| {\overrightarrow b } \right|\cos \left( {\widehat {\overrightarrow a ,\overrightarrow b }} \right)} = \sqrt {{2^2} + {{\sqrt 2 }^2} - 2.2.\sqrt 2 .\cos \left( {135^\circ } \right)} = \sqrt {10} \)
\( \Rightarrow \left| {2\overrightarrow a + 2\overrightarrow b } \right| = 2\left| {\overrightarrow a + \overrightarrow b } \right| = 2\left| {\overrightarrow c } \right| = 2\sqrt {10} \)

Đúng 0
Bình luận (0)
Trong không gian Oxyz, cho hai vectooverrightarrow{a} và overrightarrow{b} thỏa |overrightarrow{a}| 2; |overrightarrow{b}|1; (overrightarrow{a},overrightarrow{b})dfrac{pi}{3}. Góc giữa vecto overrightarrow{b} và vecto overrightarrow{a}-overrightarrow{b} bằng
Đọc tiếp
Trong không gian Oxyz, cho hai vecto\(\overrightarrow{a}\) và \(\overrightarrow{b}\) thỏa |\(\overrightarrow{a}\)| =2; |\(\overrightarrow{b}\)|=1; (\(\overrightarrow{a}\),\(\overrightarrow{b}\))=\(\dfrac{\pi}{3}\). Góc giữa vecto \(\overrightarrow{b}\) và vecto \(\overrightarrow{a}\)-\(\overrightarrow{b}\) bằng
\(cos\left(\overrightarrow{b};\overrightarrow{a}-\overrightarrow{b}\right)=\dfrac{\overrightarrow{b}\left(\overrightarrow{a}-\overrightarrow{b}\right)}{\left|\overrightarrow{b}\right|.\left|\overrightarrow{a}-\overrightarrow{b}\right|}=\dfrac{\overrightarrow{a}.\overrightarrow{b}-\overrightarrow{b}^2}{1.\sqrt{3}}=\dfrac{2.1.cos\dfrac{\pi}{3}-1^2}{\sqrt{3}}=0\)
\(\Rightarrow\left(\overrightarrow{b};\overrightarrow{a}-\overrightarrow{b}\right)=90^0\)
Đúng 0
Bình luận (0)
Cho ba vectơ overrightarrow a ,;overrightarrow b ,;overrightarrow u với |overrightarrow a |; ;,|overrightarrow b |; 1 và overrightarrow a bot overrightarrow b . Xét một hệ trục Oxy với các vectơ đơn vị overrightarrow i overrightarrow a ,;overrightarrow j overrightarrow b . Chứng minh rằng:a) Vectơ overrightarrow u có tọa độ là (overrightarrow u ,.,overrightarrow a ,;,overrightarrow u ,.,overrightarrow b )b) overrightarrow u (overrightarrow u ,.,overrightarrow a ,).overrightarrow a +...
Đọc tiếp
Cho ba vectơ \(\overrightarrow a ,\;\overrightarrow b ,\;\overrightarrow u \) với \(|\overrightarrow a |\; = \;\,|\overrightarrow b |\; = 1\) và \(\overrightarrow a \bot \overrightarrow b \). Xét một hệ trục Oxy với các vectơ đơn vị \(\overrightarrow i = \overrightarrow a ,\;\overrightarrow j = \overrightarrow b .\) Chứng minh rằng:
a) Vectơ \(\overrightarrow u \) có tọa độ là \((\overrightarrow u \,.\,\overrightarrow a \,;\,\overrightarrow u \,.\,\overrightarrow b )\)
b) \(\overrightarrow u = (\overrightarrow u \,.\,\overrightarrow a \,).\overrightarrow a + (\,\overrightarrow u \,.\,\overrightarrow b ).\overrightarrow b \)
Tham khảo:
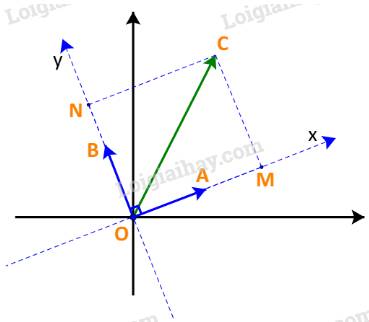
a) Trên mặt phẳng tọa độ, lấy các điểm A, B, C sao cho \(\overrightarrow {OA} = \overrightarrow a ;\;\overrightarrow {OB} = \overrightarrow b ;\;\overrightarrow {OC} = \overrightarrow u \)
Trên hệ trục Oxy với các vectơ đơn vị \(\overrightarrow i = \overrightarrow a ,\;\overrightarrow j = \overrightarrow b \), lấy M, N là hình chiếu của C trên Ox, Oy.
Gọi tọa độ của \(\overrightarrow u \)là \(\left( {x;y} \right)\). Đặt \(\alpha = \left( {\overrightarrow u ,\overrightarrow a } \right)\).
+) Nếu \({0^o} < \alpha < {90^o}\): \(x = OM = \;|\overrightarrow u |.\cos \alpha = \;|\overrightarrow u |.\cos \alpha .\;|\overrightarrow a |\; = \overrightarrow u \,.\,\overrightarrow a \,;\)
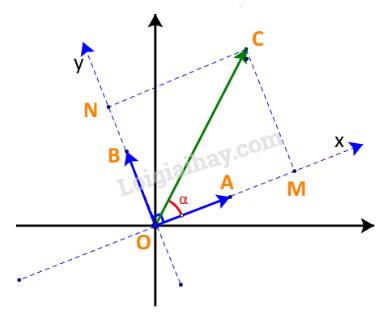
+) Nếu \({90^o} < \alpha < {180^o}\): \(x = - OM = \; - |\overrightarrow u |.\cos ({180^o} - \alpha ) = \;|\overrightarrow u |.\cos \alpha \; = \overrightarrow u \,.\,\overrightarrow a \,;\)

Như vậy ta luôn có: \(x = \overrightarrow u .\overrightarrow a \)
Chứng minh tương tự, ta có: \(y = \overrightarrow u .\overrightarrow b \)
Vậy vectơ \(\overrightarrow u \) có tọa độ là \((\overrightarrow u \,.\,\overrightarrow a \,;\,\overrightarrow u \,.\,\overrightarrow b )\)
b) Trong hệ trục Oxy với các vectơ vectơ đơn vị \(\overrightarrow i = \overrightarrow a ,\;\overrightarrow j = \overrightarrow b \), vectơ \(\overrightarrow u \) có tọa độ là \((\overrightarrow u \,.\,\overrightarrow a \,;\,\overrightarrow u \,.\,\overrightarrow b )\)
\(\begin{array}{l} \Rightarrow \overrightarrow u = (\overrightarrow u \,.\,\overrightarrow a \,).\overrightarrow i + (\,\overrightarrow u \,.\,\overrightarrow b ).\overrightarrow j \\ \Leftrightarrow \overrightarrow u = (\overrightarrow u \,.\,\overrightarrow a \,).\overrightarrow a + (\,\overrightarrow u \,.\,\overrightarrow b ).\overrightarrow b \end{array}\)
Đúng 0
Bình luận (0)
Trong không gian với hệ tọa độ Oxyz, cho overrightarrow{a} (1;-2;3) và overrightarrow{b} (1;1;-1) . Khẳng định nào dưới đây sai?A:left|overrightarrow{a}+overrightarrow{b}right|3B: overrightarrow{a}.overrightarrow{b}-4C: left|overrightarrow{a}-overrightarrow{b}right|5D: left[overrightarrow{a},overrightarrow{b}right]left(-1;-4;3right)
Đọc tiếp
Trong không gian với hệ tọa độ Oxyz, cho \(\overrightarrow{a}\) = (1;-2;3) và \(\overrightarrow{b}\) (1;1;-1) . Khẳng định nào dưới đây sai?
A:\(\left|\overrightarrow{a}+\overrightarrow{b}\right|=3\)
B: \(\overrightarrow{a}.\overrightarrow{b}=-4\)
C: \(\left|\overrightarrow{a}-\overrightarrow{b}\right|=5\)
D: \(\left[\overrightarrow{a},\overrightarrow{b}\right]=\left(-1;-4;3\right)\)
Cho hình vuông ABCD có cạnh bằng 1. Tính độ dài các vectơ sau:
a) \(\overrightarrow a = \left( {\overrightarrow {AC} + \overrightarrow {BD} } \right) + \overrightarrow {CB} ;\)
b) \(\overrightarrow a = \overrightarrow {AB} + \overrightarrow {AD} + \overrightarrow {BC} + \overrightarrow {DA} .\)
a) \(\begin{array}{l}\overrightarrow a = \left( {\overrightarrow {AC} + \overrightarrow {BD} } \right) + \overrightarrow {CB} = \left( {\overrightarrow {AC} + \overrightarrow {CB} } \right) + \overrightarrow {BD} \\ = \overrightarrow {AB} + \overrightarrow {BD} = \overrightarrow {AD}\\ \Rightarrow |{\overrightarrow a}|= \left| {\overrightarrow {AD} } \right| = AD = 1\end{array}\)
b) \(\begin{array}{l}\overrightarrow a = \overrightarrow {AB} + \overrightarrow {AD} + \overrightarrow {BC} + \overrightarrow {DA} = \left( {\overrightarrow {AB} + \overrightarrow {BC} } \right) + \left( {\overrightarrow {AD} + \overrightarrow {DA} } \right)\\ = \overrightarrow {AC} + \overrightarrow {AA} = \overrightarrow {AC} + \overrightarrow 0 = \overrightarrow {AC} \end{array}\)
\(AC = \sqrt {A{B^2} + B{C^2}} = \sqrt {{1^2} + {1^2}} = \sqrt 2 \)
\(\Rightarrow |{\overrightarrow a}|= \left| {\overrightarrow {AC} } \right| = \sqrt 2 \)
Đúng 0
Bình luận (0)
Câu 1: Trong hệ trục (O,overrightarrow{i},overrightarrow{j}), tọa độ overrightarrow{i}-overrightarrow{j}làCâu 2:Cho overrightarrow{a}(3;-4), overrightarrow{b}(-1;2). Tọa độ vecto overrightarrow{a}+2overrightarrow{b}là
Đọc tiếp
Câu 1: Trong hệ trục (O,\(\overrightarrow{i}\),\(\overrightarrow{j}\)), tọa độ \(\overrightarrow{i}\)-\(\overrightarrow{j}\)là
Câu 2:Cho \(\overrightarrow{a}\)(3;-4), \(\overrightarrow{b}\)(-1;2). Tọa độ vecto \(\overrightarrow{a}\)+2\(\overrightarrow{b}\)là
Lời giải:
$\overrightarrow{i}=(1,0), \overrightarrow{j}=(0,1)$
$\Rightarrow \overrightarrow{i}-\overrightarrow{j}=(1-0,0-1)=(1,-1)$
Đúng 1
Bình luận (0)
Bài 2:
$\overrightarrow{a}+2\overrightarrow{b}=(3+2.-1, -4+2.2)=(1, 0)$
Đúng 1
Bình luận (0)
Cho vectơ \(\overrightarrow a \). Hãy xác định độ dài và hướng của hai vectơ \(\overrightarrow a + \overrightarrow a ,\left( { - \overrightarrow a } \right) + \left( { - \overrightarrow a } \right)\): (Hình 1)
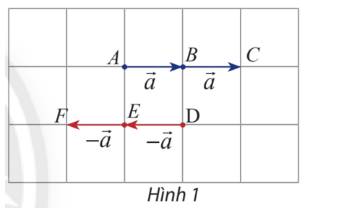
Dựa vào hình 1 ta thấy
Vectơ \(\overrightarrow a + \overrightarrow a = \overrightarrow {AC} \) có độ dài bằng 2 lần vectơ \(\overrightarrow a \)và cùng hướng với vectơ \(\overrightarrow a \)
Vectơ \(\left( { - \overrightarrow a } \right) + \left( { - \overrightarrow a } \right)= \overrightarrow {DF}\) có độ dài bằng 2 lần vectơ \(\left( { - \overrightarrow a } \right)\) và cùng hướng với vectơ \(\left( { - \overrightarrow a } \right)\)
Đúng 0
Bình luận (0)
Cho vectơ \(\overrightarrow a \ne \overrightarrow 0 \). Chứng minh rằng \(\frac{1}{{|\overrightarrow a |}}\;\overrightarrow a \) (hay còn được viết là \(\frac{{\overrightarrow a }}{{|\overrightarrow a |}}\)) là một vectơ đơn vị, cùng hướng với vectơ \(\overrightarrow a \).
Cách 1:
Gọi tọa độ của vectơ \(\overrightarrow a \) là (x; y).
Ta có: \(|\overrightarrow a |\, = \sqrt {{x^2} + {y^2}} \).
Đặt \(\overrightarrow i = \frac{1}{{|\overrightarrow a |}}\;.\overrightarrow a \)
\( \Rightarrow \overrightarrow i = \frac{1}{{\sqrt {{x^2} + {y^2}} }}.(x;y) = \left( {\frac{x}{{\sqrt {{x^2} + {y^2}} }};\frac{y}{{\sqrt {{x^2} + {y^2}} }}} \right)\)
\( \Rightarrow |\overrightarrow i |\, = \sqrt {{{\left( {\frac{x}{{\sqrt {{x^2} + {y^2}} }}} \right)}^2} + {{\left( {\frac{y}{{\sqrt {{x^2} + {y^2}} }}} \right)}^2}} = \sqrt {\frac{{{x^2}}}{{{x^2} + {y^2}}} + \frac{{{y^2}}}{{{x^2} + {y^2}}}} = 1\)
Mặt khác:
\(\overrightarrow i = \frac{1}{{|\overrightarrow a |}}\;.\overrightarrow a = \frac{1}{{\sqrt {{x^2} + {y^2}} }}.\overrightarrow a \) và \(\frac{1}{{\sqrt {{x^2} + {y^2}} }} > 0\) với mọi \(x,y \ne 0\)
Do đó vectơ \(\overrightarrow i \) và \(\overrightarrow a \) cùng hướng.
Vậy \(\frac{1}{{|\overrightarrow a |}}\;\overrightarrow a \) (hay \(\frac{{\overrightarrow a }}{{|\overrightarrow a |}}\)) là một vectơ đơn vị, cùng hướng với vectơ \(\overrightarrow a \).
Cách 2:
Với mọi vectơ \(\overrightarrow a \ne \overrightarrow 0 \), ta có: \(|\overrightarrow a |\; > 0 \Rightarrow k = \frac{1}{{|\overrightarrow a |}} > 0\). Đặt \(\overrightarrow i = \frac{1}{{|\overrightarrow a |}}\;.\overrightarrow a = k.\overrightarrow a \)
\(\begin{array}{l} \Rightarrow |\overrightarrow i |\, = \;|k.\overrightarrow a |\; = \;|k|.|\overrightarrow a |\;\\ \Leftrightarrow \left| {\overrightarrow {\,i} \,} \right| = k.|\overrightarrow a |\; = \frac{1}{{|\overrightarrow a |}}.|\overrightarrow a | = 1\end{array}\)
Mặt khác: \(\overrightarrow i = \frac{1}{{|\overrightarrow a |}}\;.\overrightarrow a = k.\overrightarrow a \) và \(k > 0\)
Do đó vectơ \(\overrightarrow i \) và \(\overrightarrow a \) cùng hướng.
Vậy \(\frac{1}{{|\overrightarrow a |}}\;\overrightarrow a \) (hay \(\frac{{\overrightarrow a }}{{|\overrightarrow a |}}\)) là một vectơ đơn vị, cùng hướng với vectơ \(\overrightarrow a \).
Đúng 0
Bình luận (0)













