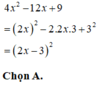dmm

a: \(\left(x+1\right)^3+\left(x-2\right)^3=2x^3+2\left(2x-1\right)^2-9\)

\(\Leftrightarrow x^3+3x^2+3x+1+x^3-6x^2+12x-8=2x^3+2\left(4x^2-4x+1\right)-9\)

\(\Leftrightarrow2x^3-3x^2+15x-7=2x^3+8x^2-8x-7\)

\(\Leftrightarrow-11x^2+23x=0\)

\(\Leftrightarrow x\left(-11x+23\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{23}{11}\end{matrix}\right.\)

1: \(\Leftrightarrow\sqrt{x-3}\left(\sqrt{x+3}+\sqrt{x-3}\right)=0\)

=>căn x-3=0

=>x-3=0

=>x=3

2: =>\(\sqrt{2x-3+2\sqrt{2x-3}+1}+\sqrt{2x-3+2\cdot\sqrt{2x-3}\cdot4+16}=5\)

=>\(\left|\sqrt{2x-3}+1\right|+\left|\sqrt{2x-3}+4\right|=5\)
=>2*căn 2x-3+5=5

=>2x-3=0

=>x=3/2

b: Ta có: \(\left(x-3\right)^3-\left(x+2\right)\left(x^2-2x+4\right)+9\left(x+2\right)^2\)

\(=x^3-9x^2+27x-27-x^3-8+9x^2+36x+36\)

\(=53x+1\)

`@` `\text {Ans}`

`\downarrow`

`a)`

`3x(4x-1) - 2x(6x-3) = 30`

`=> 12x^2 - 3x - 12x^2 + 6x = 30`

`=> 3x = 30`

`=> x = 30 \div 3`

`=> x=10`

Vậy, `x=10`

`b)`

`2x(3-2x) + 2x(2x-1) = 15`

`=> 6x- 4x^2 + 4x^2 - 2x = 15`

`=> 4x = 15`

`=> x = 15/4`

Vậy, `x=15/4`

`c)`

`(5x-2)(4x-1) + (10x+3)(2x-1) = 1`

`=> 5x(4x-1) - 2(4x-1) + 10x(2x-1) + 3(2x-1)=1`

`=> 20x^2-5x - 8x + 2 + 20x^2 - 10x +6x - 3 =1`

`=> 40x^2 -17x - 1 = 1`

`d)`

`(x+2)(x+2)-(x-3)(x+1)=9`

`=> x^2 + 2x + 2x + 4 - x^2 - x + 3x + 3=9`

`=> 6x + 7 =9`

`=> 6x = 2`

`=> x=2/6 =1/3`

Vậy, `x=1/3`

`e)`

`(4x+1)(6x-3) = 7 + (3x-2)(8x+9)`

`=> 24x^2 - 12x + 6x - 3 = 7 + (3x-2)(8x+9)`

`=> 24x^2 - 12x + 6x - 3 = 7 + 24x^2 +11x - 18`

`=> 24x^2 - 6x - 3 = 24x^2 + 18x -11`

`=> 24x^2 - 6x - 3 - 24x^2 + 18x + 11 = 0`

`=> 12x +8 = 0`

`=> 12x = -8`

`=> x= -8/12 = -2/3`

Vậy, `x=-2/3`

`g)`

`(10x+2)(4x- 1)- (8x -3)(5x+2) =14`

`=> 40x^2 - 10x + 8x - 2 - 40x^2 - 16x + 15x + 6 = 14`

`=> -3x + 4 =14`

`=> -3x = 10`

`=> x= - 10/3`

Vậy, `x=-10/3`

Hello các bạn còn đó ko?

a: =>\(\left(\dfrac{2x+1}{9}+1\right)+\left(\dfrac{2x+2}{8}+1\right)+...+\left(\dfrac{2x+9}{1}+1\right)=0\)

=>2x+10=0

=>x=-5

b: \(\Leftrightarrow\left(\dfrac{x-1}{2015}-1\right)+\left(\dfrac{x-2}{2014}-1\right)+...+\left(\dfrac{x-2014}{2}-1\right)+\left(x-2016\right)=0\)

=>x-2016=0

=>x=2016

a: =>|x-3|=4-x

\(\Leftrightarrow\left\{{}\begin{matrix}x< =4\\\left(4-x-x+3\right)\left(4-x+x-3\right)=0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x< =4\\\left(7-2x\right)=0\end{matrix}\right.\Leftrightarrow x=\dfrac{7}{2}\)

b: =>|x-5|=3-19x

\(\Leftrightarrow\left\{{}\begin{matrix}x< =\dfrac{3}{19}\\\left(x-5-3+19x\right)\left(x-5+3-19x\right)=0\end{matrix}\right.\)

\(\Leftrightarrow\left\{{}\begin{matrix}x< =\dfrac{3}{19}\\\left(20x-8\right)\left(-18x-2\right)=0\end{matrix}\right.\Leftrightarrow x\in\left\{-\dfrac{1}{9}\right\}\)

c: =>\(\Leftrightarrow\sqrt{x-3}\left(\sqrt{x+3}+\sqrt{x-3}\right)=0\)

=>căn x-3=0

=>x=3

\(\frac{4}{2x-3}-\frac{1}{2x+3}+\frac{2x+9}{9-4x^2}\)

\(\Leftrightarrow\frac{4}{2x-3}-\frac{1}{2x+3}+\frac{-2x-9}{4x^2-9}\)

\(\Leftrightarrow\frac{4\left(2x+3\right)}{\left(2x-3\right)\left(2x+3\right)}-\frac{2x-3}{\left(2x+3\right)\left(2x-3\right)}+\frac{-2x-9}{\left(2x+3\right)\left(2x-3\right)}\)

\(\Leftrightarrow\frac{8x+12-2x+3+2x-2x-9}{\left(2x-3\right)\left(2x+3\right)}\)

\(\Leftrightarrow\frac{6x+6}{\left(2x-3\right)\left(2x+3\right)}\)

\(\Leftrightarrow\frac{2\left(2x+3\right)}{\left(2x-3\right)\left(2x+3\right)}\)

\(\Leftrightarrow\frac{2}{2x-3}\)

Ta thấy \(\left(x-3\right)\left(2x+3\right)=2x^2-3x-9.\)

\(\left(1\right)\Leftrightarrow\frac{x}{x-3}-\frac{2x^2+9}{\left(x-3\right)\left(2x+3\right)}=\frac{1}{2x+3}\)

ĐK: \(x\ne3\)và \(x\ne-\frac{3}{2}\)

\(\Rightarrow x\left(2x+3\right)-2x^2-9=x-3\)

\(\Leftrightarrow2x^2+3x-2x^2-9=x-3\Leftrightarrow2x=6\Leftrightarrow x=2\)

Thỏa mãn ĐK

Các trường hợp khác làm tương tự

a,\(\left|9+x\right|=2x\)

\(\Leftrightarrow\left[{}\begin{matrix}9+x=2x\\9x+x=-2x\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}9=x\\9=-3x\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=9\\x=-3\end{matrix}\right.\)

Vậy...

Trường hợp 2 chưa chắc chắn lắm!!!

a) \(\left|9+x\right|=2x\)

Xét trường hợp 1:

\(9+x=2x\)

\(\Leftrightarrow9+x-2x=0\)

\(\Leftrightarrow9-x=0\)

\(\Leftrightarrow x=9\)

Xét trường hợp 2:

\(9+x=-2x\)

\(\Leftrightarrow9+x-\left(-2x\right)=0\)

\(\Leftrightarrow9+x+2x=0\)

\(\Leftrightarrow9+3x=0\)

\(\Leftrightarrow3x=-9\)

\(\Leftrightarrow x=-9:3\)

\(\Leftrightarrow x=-3\)

Vậy x=9 hoặc x=-3

b) \(\left|x+6\right|-9=2x\)

\(\Leftrightarrow\left|x+6\right|=2x+9\)

Xét trường hợp 1:

\(x+6=2x+9\)

\(\Leftrightarrow x+6-\left(2x+9\right)=0\)

\(\Leftrightarrow x+6-2x-9=0\)

\(\Leftrightarrow-3-x=0\)

\(\Leftrightarrow x=-3\)

Xét trường hợp 2:

\(x+6=-\left(2x+9\right)\)

\(\Leftrightarrow x+6-\left[-\left(2x+9\right)\right]=0\)

\(\Leftrightarrow x+6+\left(2x+9\right)=0\)

\(\Leftrightarrow x+6+2x+9=0\)

\(\Leftrightarrow3x+15=0\)

\(\Leftrightarrow3x=-15\)

\(\Leftrightarrow x=-15:3\)

\(\Leftrightarrow x=-5\)

Vậy x=-3 hoặc x=-5

ở câu b,bạn Nguyễn Nam phải thêm điều kiện cho mỗi trường hợp

TH1: phải đi với ĐK x phải lớn hơn hoặc bằng -6

TH2:phải đi với Đk x phải bé hơn -6 nên kết quả x=-5(KTM)

Vậy x=-3