Tìm nghiệm của phương trình sin5x+ cos 2 x - sin 2 x = 0
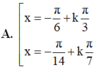
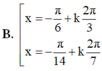
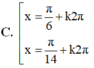
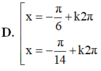
Tìm nghiệm của phương trình: sin5x + cos^2 - sin^2 =0
\(\Leftrightarrow sin5x+cos2x=0\)
\(\Leftrightarrow cos2x=-sin5x=sin\left(-5x\right)\)
\(\Leftrightarrow cos2x=cos\left(\frac{\pi}{2}+5x\right)\)
\(\Rightarrow\left[{}\begin{matrix}5x+\frac{\pi}{2}=2x+k2\pi\\5x+\frac{\pi}{2}=-2x+k2\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{6}+\frac{k2\pi}{3}\\x=\frac{\pi}{14}+\frac{k2\pi}{7}\end{matrix}\right.\)
Giải các phương trình sau :
a) \(2\cos x-\sin x=2\)
b) \(\sin5x+\cos5x=-1\)
c) \(8\cos^4x-4\cos2x+\sin4x-4=0\)
d) \(\sin^6x+\cos^6x+\dfrac{1}{2}\sin4x=0\)
Tìm số nghiệm của phương trình
cos
2
x
+
sin
x
=
0
trong khoảng ![]()
A. Vô nghiệm
B. 1
C. 2
D. 3
\(\Leftrightarrow1-2sin^2x+\left(2m-3\right)sinx+m-2=0\)
\(\Leftrightarrow2sin^2x-\left(2m-3\right)sinx-m+1=0\)
\(\Leftrightarrow2sin^2x+sinx-2\left(m-1\right)sinx-\left(m-1\right)=0\)
\(\Leftrightarrow sinx\left(2sinx+1\right)-\left(m-1\right)\left(2sinx+1\right)=0\)
\(\Leftrightarrow\left(2sinx+1\right)\left(sinx-m+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=-\dfrac{1}{2}\\sinx=m-1\end{matrix}\right.\)
Pt có đúng 2 nghiệm thuộc khoảng đã cho khi và chỉ khi:
\(\left\{{}\begin{matrix}m-1\ne-\dfrac{1}{2}\\-1\le m-1\le1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}m\ne\dfrac{1}{2}\\0\le m\le2\end{matrix}\right.\)
Giải phương trình sau :
a) \(1+\sin x-\cos x-\sin2x+2\cos2x=0\)
b) \(\sin x-\dfrac{1}{\sin x}=\sin^2x-\dfrac{1}{\sin^2x}\)
c) \(\cos x\tan3x=\sin5x\)
d) \(2\tan^2x+3\tan x+2cot^2x+3cotx+2=0\)
Giải PT
a1) \(\dfrac{\left(1-2\sin x\right)\cos x}{\left(1+2\sin x\right)\left(1-\sin x\right)}=\sqrt{3}\)
a2) \(2\sin17x+\sqrt{3}\cos5x+\sin5x=0\)
a3) \(\)\(\cos7x-\sin5x=\sqrt{3}\left(\cos5x-\sin7x\right)\)
a4) \(\sqrt{3}\cos5x-2\sin3x\cos2x-\sin x=0\)
a5) \(\tan x+\cot x=2\left(\sin2x+\cos2x\right)\)
Nghiệm của phương trình \(sin^4x+cos^4x+cos\left(x-\dfrac{\pi}{4}\right).sin\left(3x-\dfrac{\pi}{4}\right)-\dfrac{3}{2}=0\)
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^2-2sin^2x.cos^2x+\dfrac{1}{2}sin\left(4x-\dfrac{\pi}{2}\right)+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow1-\dfrac{1}{2}sin^22x-\dfrac{1}{2}cos4x+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow1-\dfrac{1}{2}\left(\dfrac{1-cos4x}{2}\right)-\dfrac{1}{2}cos4x+\dfrac{1}{2}sin2x-\dfrac{3}{2}=0\)
\(\Leftrightarrow-\dfrac{3}{4}-\dfrac{1}{4}cos4x+\dfrac{1}{2}sin2x=0\)
\(\Leftrightarrow-\dfrac{3}{4}-\dfrac{1}{4}\left(1-2sin^22x\right)+\dfrac{1}{2}sin2x=0\)
\(\Leftrightarrow...\)
Số nghiệm của phương trình sin x . sin 2 x + 2 . sin x . cos 2 x + sin x + cos x sin x + cos x = 3 . cos 2 x trong khoảng - π , π là:
A. 2
B. 4
C. 3
D. 5
Trong các phương trình sau: cos x = 5 - 3 (1); sin x = 1 - 2 (2); sin x + cos x = 2 (3), phương trình nào vô nghiệm?
A. (2)
B. (1)
C. (3)
D. (1) và (2)
Trong các phương trình sau: cos x = 5 - 3 (1); sin x = 1 - 2 (2); sin x + cos x = 2 (3), phương trình nào vô nghiệm?
A. (2).
B. (1).
C. (3).
D. (1) và (2).
Chọn C
Ta có: ![]() nên (1) và (2) có nghiệm.
nên (1) và (2) có nghiệm.
Cách 1:
Xét: ![]()
![]() nên (3) vô nghiệm.
nên (3) vô nghiệm.
Cách 2:
Điều kiện có nghiệm của phương trình: sin x + cos x = 2 là: 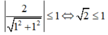
(vô lý) nên (3) vô nghiệm.
Cách 3:
Vì 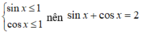
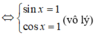
nên (3) vô nghiệm.