Giải bất phương trình sau f ' ( x ) < 0,với f ( x ) = 1 3 x 3 − 5 2 x 2 + 6 x
A. S=(2 ; 3)
B. S= ( 1; 2)
C. S= (3; 4)
D. S = (2; 4)
cho hàm số \(f\left(x\right)=x^3-3x^2+2\)
a, giải bất phương trình \(f'\left(x\right)\le0\)
b, giải phương trình \(f'=\left(x^2-3x+2\right)=0\)
c, đặt \(g\left(x\right)=f\left(1-2x\right)+x^2-x+2022\) giải bất phương trình\(g'\left(x\right)\ge0\)
\(a,f'\left(x\right)=3x^2-6x\\ f'\left(x\right)\le0\Leftrightarrow3x^2-6x\le0\\ \Leftrightarrow3x\left(x-2\right)\le0\Leftrightarrow0\le x\le2\)
Lời giải:
a. $f'(x)\leq 0$
$\Leftrightarrow 3x^2-6x\leq 0$
$\Leftrightarrow x(x-2)\leq 0$
$\Leftrightarrow 0\leq x\leq 2$
b.
$f'(x)=x^2-3x+2=0$
$\Leftrightarrow 3x^2-6x=x^2-3x+2=0$
$\Leftrightarrow 3x(x-2)=(x-1)(x-2)=0$
$\Leftrightarrow x-2=0$
$\Leftrightarrow x=2$
c.
$g(x)=f(1-2x)+x^2-x+2022$
$g'(x)=(1-2x)'f(1-2x)'_{1-2x}+2x-1$
$=-2[3(1-2x)^2-6(1-2x)]+2x-1$
$=-24x^2+2x+5$
$g'(x)\geq 0$
$\Leftrightarrow -24x^2+2x+5\geq 0$
$\Leftrightarrow (5-12x)(2x-1)\geq 0$
$\Leftrightarrow \frac{-5}{12}\leq x\leq \frac{1}{2}$
giải bất phương trình sau f(x)=(3x-4)(2x-3)/(x2-5x+6)(5-x)>0
\(f\left(x\right)=\dfrac{\left(3x-4\right)\left(2x-3\right)}{\left(x^2-5x+6\right)\left(5-x\right)}>0\)
\(\Leftrightarrow\dfrac{\left(3x-4\right)\left(2x-3\right)}{\left(x-2\right)\left(x-3\right)\left(5-x\right)}>0\)
Bảng xét dấu:
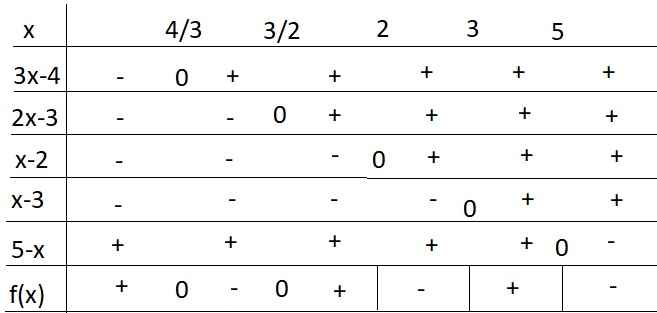
Từ bảng xét dấu ta thấy nghiệm của BPT là: \(\left[{}\begin{matrix}x< 5\\\dfrac{3}{2}< x< 2\\3< x< 5\end{matrix}\right.\)
Giải bất phương trình: 2 x f ' ( x ) ≥ 0 với f ( x ) = x + x 2 + 1
A. x ≥ 1 3
B. x > 1 3
C. x < 1 3
D. x ≥ 2 3
- TXĐ: D = R
- Ta có:
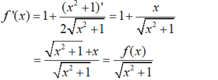
- Mặt khác:
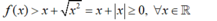
- Nên:
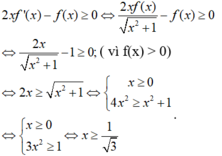
Chọn A.
Giải bất phương trình f'(x) < 0 với f ( x ) = - 2 x 4 + 4 x 2 + 1
A. - 1 < x < 0 x > 1
B. -1 < x < 0
C. x > 1
D. x < 0
Giải bất phương trình f ' ( x ) ≥ 0 với f ( x ) = 2 x 3 - 3 x 2 + 1
A. x ≤ 0 x ≥ 1
B. x ≤ 1
C. x ≥ 0
D. 0 ≤ x ≤ 1
Giải bất phương trình f'(x) < 0 với f ( x ) = - 2 x 4 + 4 x 2 + 1
A. - 1 < x < 0 x > 1
B. -1 < x < 0
C. x > 1
D. x < 0
Giải bất phương trình f ' ( x ) ≥ 0 với f ( x ) = 2 x 3 − 3 x 2 + 1
A. x ≤ 0 h o ặ c x ≥ 1
B. x ≤ 1
C. x ≥ 0
D. 0 ≤ x ≤ 1
Chọn A
Ta có: f ' ( x ) = 6 x 2 − 6 x
Để
f ' ( x ) ≥ 0 ⇔ 6 x 2 − 6 x ≥ 0 ⇔ x ≤ 0 x ≥ 1
Cho hàm số f ( x ) = x - 2 x 2 + 12 . Giải bất phương trình f'(x) ≤ 0.
Cho: f ( x ) = 2 x ; g ( x ) = x 2 2 - x 3 3 Giải bất phương trình f(x) ≤ g'(x).