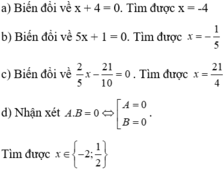a: \(0< =cos^23x< =1\)

=>\(9< =cos^23x+9< =10\)

=>9<=y<=10

\(y_{min}=9\) khi \(cos^23x=0\)

=>\(cos3x=0\)

=>3x=pi/2+kpi

=>x=pi/6+kpi/3

\(y_{max}=10\) khi \(cos^23x=0\)

=>\(sin^23x=0\)

=>3x=kpi

=>x=kpi/3

b: \(0< =sin^2x< =1\)

=>\(-3< =y< =-2\)

\(y_{min}=-3\) khi \(sin^2x=0\)

=>x=kpi

\(y_{max}=-2\) khi \(sin^2x=1\)

=>\(cos^2x=0\)

=>x=pi/2+kpi

c: \(0< =sin^25x< =1\)

=>12<=y<=13

y min=12 khi sin²5x=0

=>sin 5x=0

=>5x=kpi

=>x=kpi/5

y max=13 khi sin²5x=0

=>cos²5x=0

=>cos5x=0

=>5x=pi/2+kpi

=>x=pi/10+kpi/5

\(1,x^2+4x-2=\left(x+2\right)^2-6\ge6\)

Dấu \("="\Leftrightarrow x=-2\)

\(2.x^2+7x+1=\left(x+\dfrac{7}{2}\right)^2-\dfrac{45}{4}\ge-\dfrac{45}{4}\)

Dấu \("="\Leftrightarrow x=-\dfrac{7}{2}\)

\(3,25x^2+30x+11=\left(5x+3\right)^2+2\ge2\)

Dấu \("="\Leftrightarrow x=-\dfrac{3}{5}\)

23x+14y-8x+y

=(23x-8x)+(14y+y)

=15x+15y

=15(x+y)

\(=15\cdot100=1500\)

Bài 1 : 

\(49\left(x-2\right)^2-25\left(2x+1\right)^2=0\)

\(\Leftrightarrow\left[7\left(x-2\right)-5\left(2x+1\right)\right]\left[7\left(x-2\right)+5\left(2x+1\right)\right]=0\)

\(\Leftrightarrow\left(7x-14-10x-5\right)\left(7x-14+10x+5\right)=0\)

\(\Leftrightarrow\left(-3x-19\right)\left(17x-9\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}-3x=19\\17x=9\end{cases}\Leftrightarrow\orbr{\begin{cases}x=\frac{-19}{3}\\x=\frac{9}{17}\end{cases}}}\)

Bài 2 : 

+) \(9x^2-6xy+y^2-21x+7y\)

\(=\left(3x-y\right)^2-7\left(3x-y\right)\)

\(=\left(3x-y\right)\left(3x-y-7\right)\)

+) \(x^2+2x-35\)

\(=x^2+2x+1-36\)

\(=\left(x+1-6\right)\left(x+1+6\right)\)

\(=\left(x-5\right)\left(x+7\right)\)

+) \(2x^2+9x-5\)

\(=2x^2-x+10x-5\)

\(=x\left(2x-1\right)+5\left(2x-1\right)\)

\(=\left(2x-1\right)\left(x+5\right)\)

+) \(6x^2+23x+15\)

\(=6x^2+18x+5x+15\)

\(=6x\left(x+3\right)+5\left(x+3\right)\)

\(=\left(x+3\right)\left(6x+5\right)\)

x giữa 21 và ( là biến x hay dấu x nhân vậy bạn?

`G(x)+H(x)=(21x^2+1+17x)+(-2+6x^3-12x^2-8)`

`=21x^2+1+17x-2+6x^3-12x^2-8`

`= 6x^3+(21x^2-12x^2)+17x+(1-2-8)`

`= 6x^3+9x^2+17x-9`

`G(x)-H(x)=(21x^2+1+17x)-(-2+6x^3-12x^2-8)`

`= 21x^2+1+17x+2-6x^3+12x^2+8`

`= -6x^3+(21x^2+12x^2)+17x+(1+2+8)`

`= -6x^3+33x^2+17x+11`

`----`

`M(x)+N(x)=(7x^5 + 1 + 17x^4 - 2)+(6x^4 - 12x^2 - 23x^4 + x)`

`= 7x^5 + 1 + 17x^4 - 2+6x^4 - 12x^2 - 23x^4 + x`

`= 7x^5+(17x^4+6x^4-23x^4)-12x^2+x+(1-2)`

`= 7x^5-12x^2+x-1`

`M(x)-N(x)=(7x^5 + 1 + 17x^4 - 2)-(6x^4 - 12x^2 - 23x^4 + x)`

`= 7x^5 + 1 + 17x^4 - 2-6x^4 + 12x^2 + 23x^4 - x`

`= 7x^5+(17x^4-6x^4+23x^4)+12x^2-x+(1-2)`

`= 7x^5+34x^4+12x^2-x-1`

Mình đã trl rồi nha!

(https://hoc24.vn/cau-hoi/tinh-tong-avf-hieu-cac-da-thuc-saugx-21x2-1-17x-va-hx-2-6x3-12x2-8mx-7x5-1-17x4-2-va-nx-6x4-12x2-23x4-x.7858748287383)