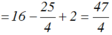tính giá trị biểu thức 0,04x 0,8x 1,25x 2x 25x 1000+ 4

Những câu hỏi liên quan
Bài 1 (2 điểm) Cho các biểu thức A = (2x - 5)/(x + 4 )và B = 1/(x + 4) - 3x/ (4 - x )- (25x - 4)/(x ^ 2 - 16 ) a) Rút gọn biểu thức B. b) Tính giá trị của B khi |3 - 2x| = 5 c) Tìm các giá trị của x để A nhỏ hơn hoặc bằng 2 / 3 B
Tính giá trị biểu thức
C= x^4 - 25x^3 + 25x^2 - 25x + 30 với x = 24
x=24 nên x+1=25
C=x^4-x^3(x+1)+x^2(x+1)-x(x+1)+30
=x^4-x^4-x^3+x^3+x^2-x^2-x+30
=-x+30
=30-24
=6
Đúng 1
Bình luận (0)
Cho x=\(\sqrt{48-24\sqrt{3}}\). Tính giá trị biểu thức
P(x)=\(\frac{2x^4-25x^3+61x^2-36x+6078}{2x^4-25x^3+61x^2-36x+27}\)
Cho 5x 4 hãy tính giá trị của biểu thức
T
25
x
-
5
2
-
x
+
5
x
2
A. T 14 B. T 47/4 C. T 118 D. T 6
Đọc tiếp
Cho 5x = 4 hãy tính giá trị của biểu thức T = 25 x - 5 2 - x + 5 x 2
A. T = 14
B. T = 47/4
C. T = 118
D. T = 6
Tìm x biết 4 - 25x^2 =0 Tính giá trị của biểu thức A=x^3 - 3x^2 + 3x - 1
1) \(4-25x^2=0\)
\(\Rightarrow\left(2-5x\right)\left(2+5x\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}5x=2\\5x=-2\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{2}{5}\\x=-\dfrac{2}{5}\end{matrix}\right.\)
2) Tính thì phải cho giá trị của x.
Đúng 1
Bình luận (2)
\(A=x^3-3x^2+3x-1\)
\(=\left(x-1\right)^3\)
\(=\left[{}\begin{matrix}\left(\dfrac{5}{2}-1\right)^3=\dfrac{27}{8}\\\left(-\dfrac{5}{2}-1\right)^3=-\dfrac{343}{8}\end{matrix}\right.\)
Đúng 1
Bình luận (0)
Câu 1: Pttnta) 25x^2(x-3y)-15(3y-x) b) x^4-5x^2+4Bài2 cho biểu thức a) Tìm điều kiện xác định của biểu thức Ab) Rút gọn biểu thức Ac) Tính giá trị của biểu thức A khi x2024
Đọc tiếp
Câu 1: Pttnt
a) 25x^2(x-3y)-15(3y-x) b) x^4-5x^2+4
Bài2 cho biểu thức 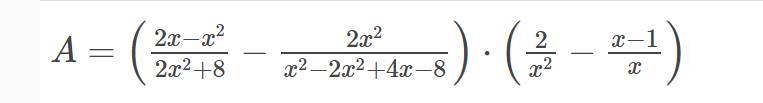
a) Tìm điều kiện xác định của biểu thức A
b) Rút gọn biểu thức A
c) Tính giá trị của biểu thức A khi x=2024
Câu 2:
a: ĐKXĐ: \(x\notin\left\{0;2\right\}\)
b: Sửa đề: \(A=\left(\dfrac{2x-x^2}{2x^2+8}-\dfrac{2x^2}{x^3-2x^2+4x-8}\right)\cdot\left(\dfrac{2}{x^2}-\dfrac{x-1}{x}\right)\)
\(=\left(\dfrac{2x-x^2}{2\left(x^2+4\right)}-\dfrac{2x^2}{\left(x-2\right)\left(x^2+4\right)}\right)\cdot\dfrac{2-x\left(x-1\right)}{x^2}\)
\(=\left(\dfrac{\left(2x-x^2\right)\left(x-2\right)-4x^2}{2\left(x^2+4\right)\left(x-2\right)}\right)\cdot\dfrac{2-x^2+x}{x^2}\)
\(=\dfrac{\left(x^2-2x\right)\left(x-2\right)+4x^2}{2\left(x^2+4\right)\left(x-2\right)}\cdot\dfrac{x^2-x-2}{x^2}\)
\(=\dfrac{x^3-2x^2-2x^2+4x+4x^2}{2\left(x^2+4\right)\left(x-2\right)}\cdot\dfrac{\left(x-2\right)\left(x+1\right)}{x^2}\)
\(=\dfrac{x^3+4x}{2\left(x^2+4\right)}\cdot\dfrac{x+1}{x^2}\)
\(=\dfrac{x\left(x^2+4\right)\left(x+1\right)}{2\left(x^2+4\right)\cdot x^2}=\dfrac{x+1}{2x}\)
c: Khi x=2024 thì \(A=\dfrac{2024+1}{2\cdot2024}=\dfrac{2025}{4048}\)
Câu 1:
a: \(25x^2\left(x-3y\right)-15\left(3y-x\right)\)
\(=25x^2\left(x-3y\right)+15\left(x-3y\right)\)
\(=\left(x-3y\right)\left(25x^2+15\right)\)
\(=\left(x-3y\right)\cdot5\cdot\left(5x^2+3\right)\)
b: \(x^4-5x^2+4\)
\(=x^4-x^2-4x^2+4\)
\(=\left(x^4-x^2\right)-\left(4x^2-4\right)\)
\(=x^2\left(x^2-1\right)-4\left(x^2-1\right)\)
\(=\left(x^2-1\right)\left(x^2-4\right)=\left(x-1\right)\left(x+1\right)\left(x-2\right)\left(x+2\right)\)
Đúng 1
Bình luận (0)
Tính giá trị biểu thức 4.(-1+2-3+4-5+6-7+.....+1000)
tìm giá trị nhỏ nhất của biểu thức
C = \(\sqrt{25x^2-20x+4}+\sqrt{25x^2}\)
\(C=\sqrt{25x^2-20x+4}+\sqrt{25x^2}\)
\(C=\sqrt{\left(5x-2\right)^2}+\sqrt{\left(5x\right)^2}\)
\(C=\left|5x-2\right|+\left|5x\right|=\left|2-5x\right|+\left|5x\right|\)
\(C\ge\left|2-5x+5x\right|=2\)
Dấu " = " xảy ra \(\Leftrightarrow\)( 2 - 5x ) . 5x \(\ge\)0
\(\Leftrightarrow\)\(\hept{\begin{cases}x\ge0\\2-5x\ge0\end{cases}}\)hoặc \(\hept{\begin{cases}x\le0\\2-5x\le0\end{cases}}\)
\(\Leftrightarrow\)\(0\le x\le\frac{2}{5}\)
Vậy GTNN của C là 2 \(\Leftrightarrow\)\(0\le x\le\frac{2}{5}\)
Đúng 0
Bình luận (0)
\(C=\sqrt{25x^2-20x+4}+\sqrt{25x^2}\)
\(C=\sqrt{\left(5x-2\right)^2}+\sqrt{\left(5x\right)^2}\)
\(C=\left|5x-2\right|+\left|5x\right|\)
\(C=\left|2-5x\right|+\left|5x\right|\ge\left|2-5x+5x\right|=2\)
Dấu "=" xảy ra \(\Leftrightarrow\hept{\begin{cases}2-5x\ge0\\5x\ge0\end{cases}\Leftrightarrow\hept{\begin{cases}x\le\frac{2}{5}\\x\ge0\end{cases}\Leftrightarrow0\le}x\le\frac{2}{5}}\)
Đúng 0
Bình luận (0)
giá trị nhỏ nhất của biểu thức 25x^2+3y^2-10x+3y+4
TL
3y2+3y+25x2-10x+4
HT
TL:
3y2 + 3y + 25x2 - 10x + 4
~HT~
= 25x2 - 10x + 1 + 3y2 + 3y + \(\frac{3}{4}\)+ \(\frac{9}{4}\)
= (5x - 1)2 + 3(y + \(\frac{1}{2}\))2 + \(\frac{9}{4}\)> \(\frac{9}{4}\) (Vì (5x - 1)2 >= 0 với mọi x; 3(y + \(\frac{1}{2}\))2 >= 0 với mọi y)
Dấu '=' xảy ra khi
5x - 1 = 0 và y+ \(\frac{1}{2}\) = 0
x = \(\frac{1}{5}\) và y = \(-\frac{1}{2}\)
Vậy ......
(Nếu sai thì mình xin lỗi)