0,5x-2/3(x-1)=1/6
a) Ta có: \(7-\left(2x+4\right)=-\left(x+4\right)\)
\(\Leftrightarrow7-2x-4=-x-4\)
\(\Leftrightarrow-2x+3+x+4=0\)
\(\Leftrightarrow-x+7=0\)
\(\Leftrightarrow-x=-7\)
hay x=7
Vậy: S={7}
b) Ta có: \(\dfrac{2+x}{5}-0.5x=\dfrac{1-2x}{4}+0.25\)
\(\Leftrightarrow\dfrac{4\left(2+x\right)}{20}-\dfrac{0.5x\cdot20}{20}=\dfrac{5\left(1-2x\right)}{20}+\dfrac{20\cdot0.25}{20}\)
\(\Leftrightarrow4\left(2+x\right)-10x=5\left(1-2x\right)+5\)
\(\Leftrightarrow8+4x-10x=5-10x+5\)
\(\Leftrightarrow-6x+8=-10x+10\)
\(\Leftrightarrow-6x+8+10x-10=0\)
\(\Leftrightarrow4x-2=0\)
\(\Leftrightarrow4x=2\)
hay \(x=\dfrac{1}{2}\)
Vậy: \(S=\left\{\dfrac{1}{2}\right\}\)
d) Ta có: \(\dfrac{x-1}{59}+\dfrac{x-2}{58}+\dfrac{x-3}{57}=\dfrac{x-59}{1}+\dfrac{x-58}{2}+\dfrac{x-57}{3}\)
\(\Leftrightarrow\dfrac{x-1}{59}-1+\dfrac{x-2}{58}-1+\dfrac{x-3}{57}-1=\dfrac{x-59}{1}-1+\dfrac{x-58}{2}-1+\dfrac{x-57}{3}-1\)
\(\Leftrightarrow\dfrac{x-60}{59}+\dfrac{x-60}{58}+\dfrac{x-60}{57}=\dfrac{x-60}{1}+\dfrac{x-60}{2}+\dfrac{x-60}{3}\)
\(\Leftrightarrow\left(x-60\right)\left(\dfrac{1}{59}+\dfrac{1}{58}+\dfrac{1}{57}\right)-\left(x-60\right)\left(1+\dfrac{1}{2}+\dfrac{1}{3}\right)=0\)
\(\Leftrightarrow\left(x-60\right)\left(\dfrac{1}{59}+\dfrac{1}{58}+\dfrac{1}{57}-1-\dfrac{1}{2}-\dfrac{1}{3}\right)=0\)
mà \(\dfrac{1}{59}+\dfrac{1}{58}+\dfrac{1}{57}-1-\dfrac{1}{2}-\dfrac{1}{3}\ne0\)
nên x-60=0
hay x=60
Vậy: S={60}
Câu1
(3-x):0,16 = -9:(x-3)
Câu2
x+1/2x+1=0,5x+2/x+3
Câu3
(x+y-1/2)^2+(x-y+1/6)^2=0
Câu4
x.(x-1/4)>0
Tìm các cặp đường thẳng song song trong các đường thẳng cho sau đây :
a, 3y-6x+1=0
b, y= -0,5x-4
c, y= 3+ \(\frac{x}{2}\)
d, 2y+x=6
e, 2x-y=1
f, y=0,5x+1
a: =>3y=6x-1
=>y=2x-1/3
Vậy: (a)//(e)
b: y=-0,5x-4
c: y=1/2x+3
d: =>2y=6-x
=>2y=(6-x)/2=-0,5x+3
f: =>y=0,5x+1=1/2x+1
Vậy: (c)//(f), (d)//(b)
Tìm GTNN của các biểu thức:
a) A = (x + 8)4 + (x + 6)4
b) B = (0,5x2 + x)2 - 3 |0,5x2 + x|
c) C = (x - 1)(x + 3)(x2 - 4x + 5)
d) D = x4 - 2x3 + 3x2 - 2x + 1
e) E = x4 - 6x3 + 10x2 - 6x + 9
g) G = (x2 + x - 6) (x2 + x + 2)
b)Ta có:\(B=\left(0,5x^2+x\right)^2-3\left|0,5x^2+x\right|\)
\(B=\left|0,5x^2+x\right|^2-3\left|0,5x^2+x\right|+\dfrac{9}{4}-\dfrac{9}{4}\)
\(B=\left(\left|0,5x^2+x\right|-\dfrac{3}{2}\right)^2-\dfrac{9}{4}\ge-\dfrac{9}{4}\)
"="<=>\(\left|0,5x^2+x\right|=\dfrac{3}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-3\end{matrix}\right.\)
g)Ta có:\(G=\left(x^2+x-6\right)\left(x^2+x+2\right)\)
Đặt \(x^2+x-2=t\)
\(\Rightarrow G=\left(t-4\right)\left(t+4\right)\)
\(G=t^2-16\ge-16\)
"="<=>\(x^2+x-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
E=\(x^4-6x^3+9x^2+x^2-6x+9\)
\(=x^2\left(x^2-6x+9\right)+x^2-6x+9\\ =x^2\left(x-3\right)^2+\left(x-3\right)^2\ge0\forall x\\ E_{min}=0\Leftrightarrow x=3\)
1\(|0,5x|=0,5x\)
2\(|\frac{1}{3}x|=\frac{1}{3}x\)
3\(|-\frac{2}{3}|=\frac{2}{3}x\)
4\(|-\frac{1}{5}|=\frac{1}{5}x\)
\(\hept{\begin{cases}\text{|}0,5x\text{|}=0,5x\\\sqrt{\left(0,5x\right)^2}=0,5x\\\left(0,5x\right)^2=\left(0,5x\right)^2\end{cases}}\)
2, tương tự
\(\hept{\begin{cases}\text{|}-\frac{2}{3}x\text{|}=\frac{2}{3}x\\\sqrt{\left(-\frac{2}{3}x\right)^2}=\frac{2}{3}x\\\left(-\frac{2}{3}x\right)^2=\left(\frac{2}{3}x\right)^2\end{cases}}\)
4, tương tự
x-3/4=1/2
-5/6-x=7/12+(-1)/3
0,5x-2/3x=7/12
x-25%x=1/2
-5/6+8/3+29/-6 nhỏ hơn hoặc bằng x nhỏ hơn hoặc bằng -1/2+2+5/2
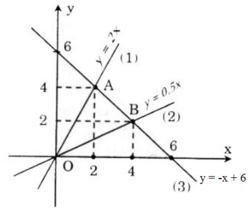
Vẽ đồ thị các hàm số sau trên cùng một mặt phẳng tọa độ:
y = 2x (1); y = 0,5x (2); y = -x + 6 (3)
– Vẽ đồ thị y = 2x (1):
Cho x= 0 ⇒ y= 0 ta được O (0, 0)
Cho x= 2 ⇒ y = 4 ta được điểm (2; 4)
- Vẽ đồ thị y = 0,5x (2):
Cho x= 0 ⇒ y = 0 ta được O (0; 0)
Cho x = 4 ⇒ y = 2 ta được điểm (4; 2)
- Vẽ đồ thị y = -x + 6 (3):
Cho x = 0 ⇒ y = 6 được điểm (0; 6)
Cho y = 0 ⇒ x = 6 được điểm (6; 0)
0,5x -2/3 (x+1)=-1/12
\(0,5x-\dfrac{2}{3}\left(x+1\right)=-\dfrac{1}{12}\\ \Leftrightarrow\dfrac{1}{2}x-\dfrac{2}{3}x-\dfrac{2}{3}=-\dfrac{1}{12}\\ \Leftrightarrow\dfrac{-1}{6}x=\dfrac{7}{12}\\ \Leftrightarrow x=-\dfrac{7}{2}\)
Vậy x = -\(\dfrac{7}{2}\)
x+1/2x+1 = 0,5x+2/x+3