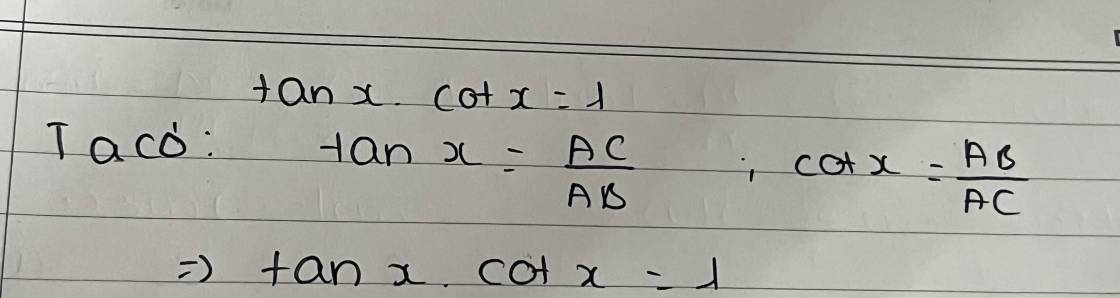Cho tam giác ABC. Với tan A 2 ,tan B 2 ,tan C 2 lập thành cấp số cộng nếu và chỉ nếu
A. sinA; sinB; sinC lập thành cấp số cộng.
B. sinA; sinB; sinC lập thành cấp số nhân
C. cosA; cosB; cosC lập thành cấp số cộng.
D. cosA; cosB; cosC lập thành cấp số nhân.