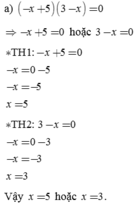bạn đăng tách ra cho mn giúp nhé 

a, Để pt có 2 nghiệm pb 

\(\Delta'=1-m\ge0\Leftrightarrow m\le1\)

Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=-2\left(1\right)\\x_1x_2=m\left(2\right)\end{matrix}\right.\)

\(x_1-3x_2=0\)(3) 

Từ (1) ; (3) ta có hệ \(\left\{{}\begin{matrix}x_1+x_2=-2\\x_1-3x_2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x_1=-2\\x_2=-2-x_1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1=-\dfrac{1}{2}\\x_2=-\dfrac{3}{2}\end{matrix}\right.\)

Thay vào (2) ta được \(m=\left(-\dfrac{1}{2}\right)\left(-\dfrac{3}{2}\right)=\dfrac{3}{4}\)

\(b,\Delta=\left(m+5\right)^2-4\left(-m+6\right)\ge0\Leftrightarrow\left[{}\begin{matrix}m\le-7-4\sqrt{3}\\m\ge-7+4\sqrt{3}\end{matrix}\right.\)

\(\Rightarrow\left\{{}\begin{matrix}x1+x2=m+5\\2x1+3x2=13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x1+2x2=2m+10\\2x1+3x2=13\end{matrix}\right.\)\(\)

\(\Rightarrow x2=13-2m-10=3-2m\Rightarrow x1=m+5-x2=m+5-3+2m=3m+2\)

\(x1x2=6-m\Rightarrow\left(3-2m\right)\left(3m+2\right)=6-m\Leftrightarrow\left[{}\begin{matrix}m=0\left(tm\right)\\m=1\left(tm\right)\end{matrix}\right.\)

\(c,\Delta'=\left(m+1\right)^2-\left(m^2-2m+29\right)\ge0\Leftrightarrow m\ge7\)

\(\Rightarrow\left\{{}\begin{matrix}x1+x2=2m+2\\x1=2x2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x2=\dfrac{2m+2}{3}\\x1=\dfrac{2\left(2m+2\right)}{3}\end{matrix}\right.\)

\(\Rightarrow x1.x2=\dfrac{\left(2m+2\right).2\left(2m+2\right)}{9}=m^2-2m+29\Leftrightarrow\left[{}\begin{matrix}m=11\left(tm\right)\\m=23\left(tm\right)\end{matrix}\right.\)

Ta có (1)  ⇔ x 4 + x 2 + 20 = y 2 + y

Ta thấy:  x 4 + x 2 < x 4 + x 2 + 20 ≤ x 4 + x 2 + 20 + 8 x 2 ⇔ x 2 ( x 2 + 1 ) < y ( y + 1 ) ≤ ( x 2 + 4 ) ( x 2 + 5 )

Vì x, y ∈ Z nên ta xét các trường hợp sau

+ TH1.  y ( y + 1 ) = ( x 2 + 1 ) ( x 2 + 2 ) ⇔ x 4 + x 2 + 20 = x 4 + 3 x 2 + 2 ⇔ 2 x 2 = 18 ⇔ x 2 = 9 ⇔ x = ± 3

Với  x 2 = 9   ⇒ y 2 + y = 9 2 + 9 + 20 ⇔ y 2 + y − 110 = 0 ⇔ y = 10 ; y = − 11 ( t . m )

+ TH2  y ( y + 1 ) = ( x 2 + 2 ) ( x 2 + 3 ) ⇔ x 4 + x 2 + 20 = x 4 + 5 x 2 + 6 ⇔ 4 x 2 = 14 ⇔ x 2 = 7 2   ( l o ạ i )

+ TH3: y ( y + 1 ) = ( x 2 + 3 ) ( x 2 + 4 ) ⇔ 6 x 2 = 8 ⇔ x 2 = 4 3   ( l o ạ i )

+ TH4:  y ( y + 1 ) = ( x 2 + 4 ) ( x 2 + 5 ) ⇔ 8 x 2 = 0 ⇔ x 2 = 0 ⇔ x = 0

Với  x 2 = 0  ta có  y 2 + y = 20 ⇔ y 2 + y − 20 = 0 ⇔ y = − 5 ; y = 4

Vậy PT đã cho có nghiệm nguyên (x;y) là :

(3;10), (3;-11), (-3; 10), (-3;-11), (0; -5), (0;4).

x(x – 1)(x + 1) + x 2 – 1 = 0

ó x(x – 1)(x + 1) + ( x 2 – 1) = 0

ó x(x – 1)(x + 1) + (x – 1)(x + 1) = 0

ó (x + 1)(x – 1)(x + 1) = 0

ó ( x   +   1 ) 2 (x – 1) = 0

Vậy x = -1 hoặc x = 1

Tổng các giá trị của x là 1 + (-1) = 0

Đáp án cần chọn là: D

\(1,\Leftrightarrow x^2-10x+25=0\Leftrightarrow\left(x-5\right)^2=0\Leftrightarrow x=5\left(B\right)\\ 2,Giống.1\\ 3,=5x^2\left(B\right)\\ 4,x^3=x\Leftrightarrow x^3-x=0\\ \Leftrightarrow x\left(x^2-1\right)=0\\ \Leftrightarrow x\left(x-1\right)\left(x+1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\x=-1\end{matrix}\right.\\ \Leftrightarrow A\)

\(x^2+3x+5=xy+2y\\ \Leftrightarrow x^2+3x-xy-2y+5=0\\ \Leftrightarrow x\left(x+2\right)-y\left(x+2\right)+\left(x+2\right)+3=0\\ \Leftrightarrow\left(x+2\right)\left(x-y+1\right)=-3=\left(-1\right)\cdot3=\left(-3\right)\cdot1\)

\(TH_1:\left\{{}\begin{matrix}x+2=-3\\x-y+1=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-5\\y=-5\end{matrix}\right.\to\left(-5;-5\right)\\ TH_2:\left\{{}\begin{matrix}x+2=3\\x-y+1=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=3\end{matrix}\right.\to\left(1;3\right)\\ TH_3:\left\{{}\begin{matrix}x+2=1\\x-y+1=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=3\end{matrix}\right.\to\left(-1;3\right)\\ TH_4:\left\{{}\begin{matrix}x+2=-1\\x-y+1=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-3\\y=-5\end{matrix}\right.\to\left(-3;-5\right)\)

Vậy \(\left(x;y\right)=\left(-5;-5\right);\left(1;3\right);\left(-1;3\right);\left(-3;-5\right)\)