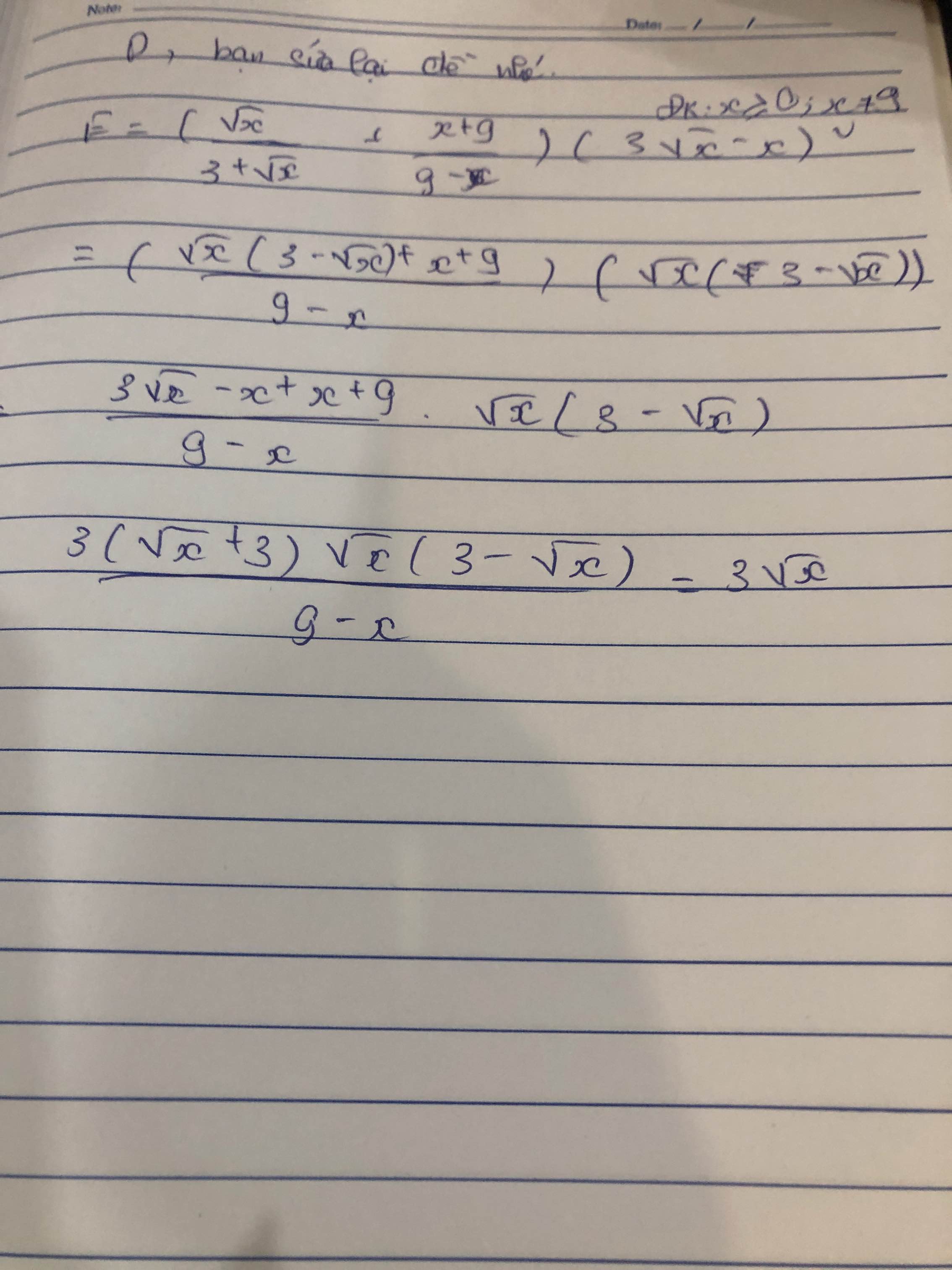Rút gọn biểu thức:\(\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\)

Những câu hỏi liên quan
Rút gọn biểu thức M = \(\dfrac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}:2\sqrt{1+\dfrac{2x}{3-x}}\)
\(M=\dfrac{\left(x+2\right)\left(x+3\right)+x\sqrt{\left(3-x\right)\left(3+x\right)}}{x\left(3-x\right)+\left(x+2\right)\sqrt{\left(3-x\right)\left(3+x\right)}}:2\sqrt{\dfrac{3-x+2x}{3-x}}\left(-3\le x< 3;x\ne-1\right)\\ M=\dfrac{\sqrt{x+3}\left(x+2+x\sqrt{3-x}\right)}{\sqrt{3-x}\left[x+\left(x+2\right)\sqrt{3+x}\right]}:2\sqrt{\dfrac{x+3}{3-x}}\\ M=\dfrac{\sqrt{x+3}\left(x+2+x\sqrt{3-x}\right)}{\sqrt{3-x}\left[x+\left(x+2\right)\sqrt{3+x}\right]}\cdot\dfrac{3-x}{2\sqrt{\left(3-x\right)}\sqrt{\left(x+3\right)}}\)
\(M=\dfrac{x+2+x\sqrt{3-x}}{x+\left(x+2\right)\sqrt{3-x}}\cdot\dfrac{\sqrt{3-x}}{2\sqrt{3-x}}\\ M=\dfrac{\left(x+2\right)\sqrt{3-x}+x\left(3-x\right)}{2x\sqrt{3-x}+2\left(x+2\right)\sqrt{3-x}}\\ M=\dfrac{\sqrt{3-x}\left(2x+2\right)}{\sqrt{3-x}\left(2x+2x+4\right)}=\dfrac{2\left(x+1\right)}{4\left(x+1\right)}=\dfrac{1}{2}\)
Đúng 1
Bình luận (0)
cho biểu thức M=\(\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\) : \(2\sqrt{1+\frac{2x}{3-x}}\)
rút gọn M
a chứng minh rằng: \(\dfrac{x+3+2.\sqrt{x^2-9}}{2x-6+\sqrt{x^2-9}}=\dfrac{\sqrt{x^2-9}}{x-3}\)
b rút gọn biểu thức T = \(\dfrac{x^2+5x+6+x.\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\)
Rút gọn biểu thức
A = \(\frac{x+3+2\sqrt{x^2-9}}{2x-6+\sqrt{x^2-9}}\)
B = \(\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\)
Giúp mk vs , mk đang cần gấp
Rút gọn:
\(A=\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right).\sqrt{9-x^2}}\)
\(B=\frac{x^2-5x+6+3\sqrt{x^2-6x+8}}{3x-12+\left(x-3\right).\sqrt{x^2-6x+8}}\)
\(C=\frac{\sqrt{2\sqrt{4-x^2}}.\left(\sqrt{\left(2+x\right)^3}-\sqrt{\left(2-x\right)^3}\right)}{4+\sqrt{4-x^2}}\)
Rút gọn:
\(A=\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right).\sqrt{9-x^2}}\)
\(B=\frac{x^2-5x+6+3\sqrt{x^2-6x+8}}{3x-12+\left(x-3\right).\sqrt{x^2-6x+8}}\)
\(C=\frac{\sqrt{2\sqrt{4-x^2}}.\left(\sqrt{\left(2+x\right)^3}-\sqrt{\left(2-x\right)^3}\right)}{4+\sqrt{4-x^2}}\)
\(A=\frac{x^2+5x+6+x\sqrt{9-x^2}}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\)
\(=\frac{\left(x+2\right)\left(x+3\right)+x\sqrt{\left(3-x\right)\left(3+x\right)}}{x\left(3-x\right)+\left(x+2\right)\sqrt{\left(3-x\right)\left(3+x\right)}}\)
\(=\frac{\left(x+2\right)\left(x+3\right)+x\sqrt{\left(3-x\right)\left(3+x\right)}}{x\left(3-x\right)+\left(x+2\right)\sqrt{\left(3-x\right)\left(3+x\right)}}\)
\(=\frac{\sqrt{3+x}\left(\left(x+2\right)\sqrt{x+3}+x\sqrt{3-x}\right)}{\sqrt{3-x}\left(\left(x+2\right)\sqrt{x+3}+x\sqrt{3-x}\right)}\)
\(=\frac{\sqrt{3+x}}{\sqrt{3-x}}\)
Đúng 0
Bình luận (0)
\(B=\frac{x^2-5x+6+3\sqrt{x^2-6x+8}}{3x-12+\left(x-3\right)\sqrt{x^2-6x+8}}\)
\(=\frac{\left(x-3\right)\left(x-2\right)+3\sqrt{\left(x-4\right)\left(x-2\right)}}{3\left(x-4\right)+\left(x-3\right)\sqrt{\left(x-4\right)\left(x-2\right)}}\)
\(=\frac{\sqrt{x-2}\left(\left(x-3\right)\sqrt{x-2}+3\sqrt{x-4}\right)}{\sqrt{x-4}\left(3\sqrt{x-4}+\left(x-3\right)\sqrt{x-2}\right)}\)
\(=\frac{\sqrt{x-2}}{\sqrt{x-4}}\)
Đúng 0
Bình luận (0)
\(C=\frac{\sqrt{2\sqrt{4-x^2}}.\left(\sqrt{\left(2+x\right)^3}-\sqrt{\left(2-x\right)^3}\right)}{4+\sqrt{4-x^2}}\)
Đặt \(\hept{\begin{cases}\sqrt{2+x}=a\\\sqrt{2-x}=b\end{cases}\Rightarrow}a^2+b^2=4\)
\(\Rightarrow C=\frac{\sqrt{2ab}.\left(a^3-b^3\right)}{a^2+b^2+ab}=\frac{\sqrt{2ab}.\left(a-b\right)\left(a^2+b^2+ab\right)}{a^2+b^2+ab}\)
\(=\sqrt{2ab}.\left(a-b\right)=\sqrt{2\sqrt{4-x^2}}.\left(\sqrt{2+x}-\sqrt{2-x}\right)\)
Đúng 0
Bình luận (0)
Rút gọn các biểu thức sau:
\(D=\left(\frac{5\sqrt{x-6}}{x-9}-\frac{2}{\sqrt{x}+3}\right):\left(1+\frac{6}{x-9}\right)\)
\(E=\left(\frac{\sqrt{x}}{3+\sqrt{x}}+\frac{9+x}{9-x}\right).\left(3\sqrt{x}-x\right)\)
Cho biểu thức: \(P=\left(\frac{\sqrt{x}-3}{2-\sqrt{x}}+\frac{\sqrt{x}+2}{3+\sqrt{x}}-\frac{9-x}{x+\sqrt{x}-6}\right):\left(1-\frac{3\sqrt{x}-9}{x-9}\right)\)
a)Rút gọn biểu thức
b)Tính P với \(x=\frac{\sqrt{4+2\sqrt{3}}\left(\sqrt{x}-1\right)}{\sqrt{6+2\sqrt{5}-\sqrt{5}}}\)
Mình ghi nhầm. \(x=\frac{\sqrt{4+2\sqrt{3}}.\left(\sqrt{3}-1\right)}{\sqrt{6+2\sqrt{5}}-\sqrt{5}}\)nhé
rút gọn hộ mình con này với
\(\frac{x^2+5x+x\sqrt{9-x^2}+6}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\)
\(\frac{x^2+5x+x\sqrt{9-x^2}+6}{3x-x^2+\left(x+2\right)\sqrt{9-x^2}}\left(DK:-3\le x< 3\right)\)
\(=\frac{\left(x+2\right)\left(x+3\right)+x\sqrt{\left(3-x\right)\left(3+x\right)}}{x\left(3-x\right)+\left(x+2\right)\sqrt{\left(3-x\right)\left(3+x\right)}}\)
\(=\frac{\sqrt{x+3}\left[\sqrt{x+3}\left(x+2\right)+x\sqrt{3-x}\right]}{\sqrt{3-x}\left[x\sqrt{3-x}+\left(x+2\right)\sqrt{x+3}\right]}=\frac{\sqrt{x+3}\left(x\sqrt{x+3}+2\sqrt{x+3}+x\sqrt{3-x}\right)}{\sqrt{3-x}\left(x\sqrt{x+3}+2\sqrt{x+3}+x\sqrt{3-x}\right)}=\frac{\sqrt{x+3}}{\sqrt{3-x}}=\sqrt{\frac{x+3}{3-x}}\)
Đúng 0
Bình luận (0)