Tìm x, y ∈ Z biết
2x2 + xy - 2x - y - 3 = 0
Những câu hỏi liên quan
Tìm x,y thuộc Z biết :
1) xy-x-y-1=0
2) xy-x-y+1=0
3) xy+2x+y+11=0
1 , sai đề
2/ xy-x-y+1=0
x(y-1)-(y-1)=0
(y-1)(x-1)=0
->y-1=o hoặc x-1=0
y-1=0 y=1
x-1=0 x=1
vậy x=y=1
3,
Đúng 0
Bình luận (0)
tìm x,y thuộc Z biết:
xy + x - 2x - 3 = 0
xy + x - 2x - 3 = 0
=> x(y + 1) - 2x = 3
=> x(y + 1) = 2x + 3
=> 2x + 3 chia hết cho x
=> 3 chia hết cho x
=> x thuộc {-1; 1; -3; 3}
Ta có bảng:
| x | 1 | -1 | 3 | -3 |
| y + 1 | 5 | -1 | 6 | 1 |
| y | 4 | -2 | 5 | 0 |
Vậy...
Đúng 0
Bình luận (0)
Tìm x,y € Z biết:
a) x+y-xy=4
b) x-y-xy=0
c) 2xy+6x-y=15
d) xy-2x+3y-1=0
Bài 1: Tìm x € Z a)1−3x chia hết cho x−2 b)3x+2 chia hết cho 2x+1 Bài 2: Tìm các số nguyên a)x(3−y)−y=0 b)xy+2x+2y=0 c)xy−2x+4y=1 d)x(y+1)+y=0
Bài 1:a) Ta có: \(1-3x⋮x-2\)
\(\Leftrightarrow-3x+1⋮x-2\)
\(\Leftrightarrow-3x+6-5⋮x-2\)
mà \(-3x+6⋮x-2\)
nên \(-5⋮x-2\)
\(\Leftrightarrow x-2\inƯ\left(-5\right)\)
\(\Leftrightarrow x-2\in\left\{1;-1;5;-5\right\}\)
hay \(x\in\left\{3;1;7;-3\right\}\)
Vậy: \(x\in\left\{3;1;7;-3\right\}\)
b) Ta có: \(3x+2⋮2x+1\)
\(\Leftrightarrow2\left(3x+2\right)⋮2x+1\)
\(\Leftrightarrow6x+4⋮2x+1\)
\(\Leftrightarrow6x+3+1⋮2x+1\)
mà \(6x+3⋮2x+1\)
nên \(1⋮2x+1\)
\(\Leftrightarrow2x+1\inƯ\left(1\right)\)
\(\Leftrightarrow2x+1\in\left\{1;-1\right\}\)
\(\Leftrightarrow2x\in\left\{0;-2\right\}\)
hay \(x\in\left\{0;-1\right\}\)
Vậy: \(x\in\left\{0;-1\right\}\)
Đúng 4
Bình luận (1)
Bài 1 :
a, Có : \(1-3x⋮x-2\)
\(\Rightarrow-3x+6-5⋮x-2\)
\(\Rightarrow-3\left(x-2\right)-5⋮x-2\)
- Thấy -3 ( x - 2 ) chia hết cho x - 2
\(\Rightarrow-5⋮x-2\)
- Để thỏa mãn yc đề bài thì : \(x-2\inƯ_{\left(-5\right)}\)
\(\Leftrightarrow x-2\in\left\{1;-1;5;-5\right\}\)
\(\Leftrightarrow x\in\left\{3;1;7;-3\right\}\)
Vậy ...
b, Có : \(3x+2⋮2x+1\)
\(\Leftrightarrow3x+1,5+0,5⋮2x+1\)
\(\Leftrightarrow1,5\left(2x+1\right)+0,5⋮2x+1\)
- Thấy 1,5 ( 2x +1 ) chia hết cho 2x+1
\(\Rightarrow1⋮2x+1\)
- Để thỏa mãn yc đề bài thì : \(2x+1\inƯ_{\left(1\right)}\)
\(\Leftrightarrow2x+1\in\left\{1;-1\right\}\)
\(\Leftrightarrow x\in\left\{0;-1\right\}\)
Vậy ...
Đúng 1
Bình luận (1)
TÌM X,Y THUỘC Z BIẾT
xy+x-2y=3
3x+5y+175
3xy+6x+y_32=0
2x+5y+3xy=8
4xy-3(x+y)=59
xy-x-y=2
Tìm x,y thuộc Z biết :
a) xy+3x-2y=11
b) xy+2x+y+11=0
a) \(xy+3x-2y-11=0\)
\(x\left(y+3\right)-2y-6-5=0\)
\(x\left(y+3\right)-2\left(y+3\right)=5\)
\(\left(x-2\right)\left(y+3\right)=5\)
\(x-2;y+3\in U\left(5\right)\)
| x-2 | 1 | -1 | 5 | -5 |
| y+3 | 5 | -5 | 1 | -1 |
| x | 3 | 1 | 7 | -3 |
| y | 2 | -8 | -2 | -4 |
b) \(xy+2x+y+11=0\)
\(x\left(y+2\right)+y+2+9=0\)
\(x\left(y+2\right)+\left(y+2\right)=-9\)
\(\left(x+1\right)\left(y+2\right)=-9\)
\(x+1;y+2\in U\left(-9\right)\)
| x+1 | 1 | -1 | 3 | -3 | 9 | -9 |
| y+2 | -9 | 9 | -3 | 3 | -1 | 1 |
| x | 0 | -2 | 2 | -4 | 8 | -10 |
| y | -11 | 7 | -5 | 1 | -3 | -1 |
Đúng 0
Bình luận (0)
a) $xy+3x-2y-11=0$$x\left(y+3\right)-2y-6-5=0$$x\left(y+3\right)-2\left(y+3\right)=5$$\left(x-2\right)\left(y+3\right)=5$$x-2;y+3\in U\left(5\right)$
b) $xy+2x+y+11=0$
$x\left(y+2\right)+y+2+9=0$$x\left(y+2\right)+\left(y+2\right)=-9$$\left(x+1\right)\left(y+2\right)=-9$$x+1;y+2\in U\left(-9\right)$
| x-2 | 1 | -1 | 5 | -5 | ||
| y+3 | 5 | -5 | 1 | -1 | ||
| x | 3 | 1 | 7 | -3 | ||
| y | 2 | -8 | -2 | -4 | ||
| x+1 | 1 | -1 | 3 | -3 | 9 | -9 |
| y+2 | -9 | 9 | -3 | 3 | -1 | 1 |
| x | 0 | -2 | 2 | -4 | 8 | -10 |
| y | -11 | 7 | -5 | 1 |
Đúng 0
Bình luận (0)
a) Phân tích nhân tử
i
)
x
y
-
6
y
+
2
x
-
12
i
i
)
2
x
(
y
-
z
)
+
(
z
-
y
)
(
x
+
y
)
b
)
T
ì
m...
Đọc tiếp
a) Phân tích nhân tử
i ) x y - 6 y + 2 x - 12 i i ) 2 x ( y - z ) + ( z - y ) ( x + y ) b ) T ì m x b i ế t : x + 3 = x + 3 2
i) xy - 6y + 2x - 12
= (xy - 6y) + (2x - 12)
= y(x - 6) + 2(x - 6)
= (x - 6)(y + 2)
ii) 2x(y - z) + (z - y)(x + y)
= 2x(y - z) - (y - z)(x + y)
= (y - z)(2x - x - y)
= (y - z)(x - y)
b) x + 3 = (x + 3)2 ⇔ (x + 3)2 - (x + 3) = 0 ⇔ (x + 3)(x + 3 - 1) = 0
⇔ (x + 3)(x + 2) = 0
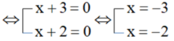
Vậy x = -3; x = -2
Đúng 0
Bình luận (0)
2.Tìm x biết:
6x^2-11x+3=0
3.Tìm x,y thuộc Z biết:
a) xy-4y+x=-1
b) 6xy+2x-9y-7=0
Tìm x,y thuộc Z, biết:
a) xy+x=3
b) 5+xy=y
c) 2x+3+y=xy
xy+x=3
x(y+1)=3
=> x,y+1 € U(3)
x€ {-1,1,-3,3}
y+1 € { -1,1,-3,3}
y €{-2,0,-4,2}
Đúng 0
Bình luận (0)
a,Ta có:\(xy+x=3\)
\(\Leftrightarrow x\left(y+1\right)=3\)
Vì x,y thuộc Z \(\hept{\begin{cases}x\\y+1\end{cases}}\in Z\)
\(\Rightarrow x;y+1\inƯ\left(3\right)\)
\(\Rightarrow x;y+1\in\left\{\pm1;\pm3\right\}\)
\(\Rightarrow\orbr{\begin{cases}x=1\\y+1=3\Rightarrow y=2\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=-1\\y+1=-3\Rightarrow y=-4\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=3\\y+1=1\Rightarrow y=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=-3\\y+1=-1\Rightarrow y=-2\end{cases}}\)
Tìm x,y biết x,y thuộc Z:
1> (x-2).(2y+1)=17
2> x.(y-3)=-12
3> (x-1).(y+2)=7
4>xy+2x+2y=-16
5> xy-3x-y=0