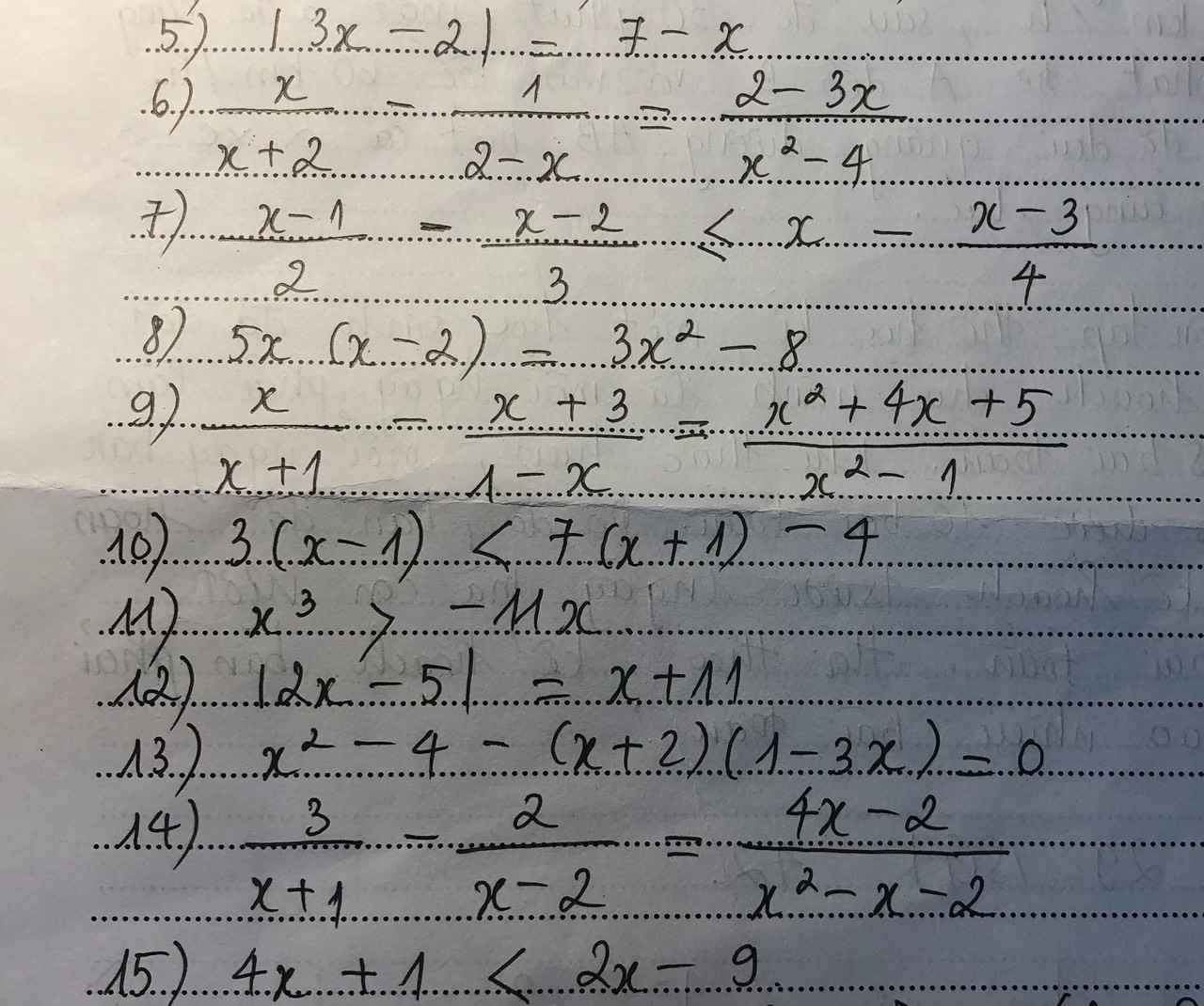`5)|3x-2|=7-x` (ĐKXĐ:`x>=2/3`)
`<=>(3x-2)=7-x`
`<=>3x-2=7-x`
`<=>3x+x=7+2`
`<=>4x=9`
`<=>x=9/4`
`6) x/{x+2} -1/{2-x}={2-3x}/{x^2 -4}` (ĐKXĐ:`x ne 2;-2`)
`<=>{x(x-2)}/{(x+2)(x-2)}+{x+2}/{(x-2)(x+2)}={2-3x}/{(x-2)(x+2)}`
`<=>x^2 -2x+x+2=2-3x`
`<=>x^2 -2x+x+2-2+3x=0`
`<=>x^2 +2x=0`
`<=> x(x+2)=0`
`<=>` \(\left[{}\begin{matrix}x=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-2\end{matrix}\right.\)
`7){x-1}/2-{x-2}/3<=- {x-3}/4`
`<=>6x-6-4x+8-12x+3x-9<=0`
`<=>-7x-7<=0`
`<=>x+1>=0`
`<=>x>=-1`
11) \(x^3>-11x\Leftrightarrow x^3+11x>0\Leftrightarrow x\left(x+11\right)>0\Leftrightarrow\left[{}\begin{matrix}x< -11\\x>0\end{matrix}\right.\)
12) \(\left|2x-5\right|=x+11\)
\(\Leftrightarrow\left\{{}\begin{matrix}x+11\ge0\\\left[{}\begin{matrix}2x-5=x+1\\2x-5=-x-1\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge-11\\\left[{}\begin{matrix}x=6\\x=\dfrac{4}{3}\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=6\\x=\dfrac{4}{3}\end{matrix}\right.\)
13) \(x^2-4-\left(x+2\right)\left(1-3x\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+2\right)-\left(x+2\right)\left(1-3x\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-2-1+3x\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(4x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=0\\4x-3=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{3}{4}\end{matrix}\right.\)
15) \(4x+1< 2x-9\)
\(\Leftrightarrow2x< -8\)
\(\Leftrightarrow x< -4\)
5: |3x-2|=7-x
=>\(\left\{{}\begin{matrix}7-x>=0\\\left(3x-2\right)^2=\left(7-x\right)^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =7\\\left(3x-2-7+x\right)\left(3x-2+7-x\right)=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =7\\\left(4x-9\right)\left(2x+5\right)=0\end{matrix}\right.\Leftrightarrow x\in\left\{\dfrac{9}{4};-\dfrac{5}{2}\right\}\)
6:
ĐKXĐ: \(x\notin\left\{2;-2\right\}\)
\(\dfrac{x}{x+2}-\dfrac{1}{2-x}=\dfrac{2-3x}{x^2-4}\)
=>\(\dfrac{x}{x+2}+\dfrac{1}{x-2}=\dfrac{2-3x}{\left(x-2\right)\left(x+2\right)}\)
=>\(\dfrac{x\left(x-2\right)+x+2}{\left(x-2\right)\left(x+2\right)}=\dfrac{2-3x}{\left(x-2\right)\left(x+2\right)}\)
=>\(x^2-2x+x+2-2+3x=0\)
=>\(x^2+2x=0\)
=>x(x+2)=0
=>\(\left[{}\begin{matrix}x=0\left(nhận\right)\\x=-2\left(loại\right)\end{matrix}\right.\)
7: \(\dfrac{x-1}{2}-\dfrac{x-2}{3}< =x-\dfrac{x-3}{4}\)
=>\(\dfrac{3\left(x-1\right)-2\left(x-2\right)}{6}< =\dfrac{4x-\left(x-3\right)}{4}\)
=>\(\dfrac{3x-3-2x+4}{6}< =\dfrac{4x-x+3}{4}\)
=>\(\dfrac{x+1}{6}< =\dfrac{3x+3}{4}\)
=>\(2\left(x+1\right)< =3\left(3x+3\right)\)
=>2x+2<=9x+9
=>-7x<=7
=>x>=-1
8:
\(5x\left(x-2\right)=3x^2-8\)
=>\(5x^2-10x-3x^2+8=0\)
=>\(2x^2-10x+8=0\)
=>\(x^2-5x+4=0\)
=>(x-1)(x-4)=0
=>\(\left[{}\begin{matrix}x-1=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=4\end{matrix}\right.\)
9: ĐKXĐ: \(x\notin\left\{1;-1\right\}\)
\(\dfrac{x}{x+1}-\dfrac{x+3}{1-x}=\dfrac{x^2+4x+5}{x^2-1}\)
=>\(\dfrac{x}{x+1}+\dfrac{x+3}{x-1}=\dfrac{x^2+4x+5}{\left(x-1\right)\left(x+1\right)}\)
=>\(\dfrac{x\left(x-1\right)+\left(x+3\right)\left(x+1\right)}{\left(x+1\right)\left(x-1\right)}=\dfrac{x^2+4x+5}{\left(x-1\right)\left(x+1\right)}\)
=>\(x^2-x+x^2+4x+3=x^2+4x+5\)
=>\(2x^2+3x+3-x^2-4x-5=0\)
=>\(x^2-x-2=0\)
=>(x-2)(x+1)=0
=>\(\left[{}\begin{matrix}x=2\left(nhận\right)\\x=-1\left(loại\right)\end{matrix}\right.\)
10: \(3\left(x-1\right)< 7\left(x+1\right)-4\)
=>\(3x-3< 7x+7-4\)
=>3x-3<7x+3
=>-4x<6
=>\(x>-\dfrac{6}{4}=-\dfrac{3}{2}\)
11: \(x^3>-11x\)
=>\(x^3+11x>0\)
=>\(x\cdot\left(x^2+11\right)>0\)
mà \(x^2+11>=11>0\forall x\)
nên x>0
12: \(\left|2x-5\right|=x+11\)
=>\(\left\{{}\begin{matrix}x+11>=0\\\left(2x-5\right)^2=\left(x+11\right)^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=-11\\\left(2x-5-x-11\right)\left(2x-5+x+11\right)=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=-11\\\left(x-16\right)\left(3x+6\right)=0\end{matrix}\right.\Leftrightarrow x\in\left\{16;-2\right\}\)
13: \(x^2-4-\left(x+2\right)\left(1-3x\right)=0\)
=>\(\left(x-2\right)\left(x+2\right)+\left(x+2\right)\left(3x-1\right)=0\)
=>\(\left(x+2\right)\left(x-2+3x-1\right)=0\)
=>\(\left(x+2\right)\left(4x-3\right)=0\)
=>\(\left[{}\begin{matrix}x+2=0\\4x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{3}{4}\end{matrix}\right.\)
14 ĐKXĐ: \(x\notin\left\{2;-1\right\}\)
\(\dfrac{3}{x+1}-\dfrac{2}{x-2}=\dfrac{4x-2}{x^2-x-2}\)
=>\(\dfrac{3\left(x-2\right)-2\left(x+1\right)}{\left(x+1\right)\left(x-2\right)}=\dfrac{4x-2}{\left(x-2\right)\left(x+1\right)}\)
=>3x-6-2x-2=4x-2
=>x-8=4x-2
=>x-4x=-2+8
=>-3x=6
=>x=-2(nhận)
15: 4x+1<2x-9
=>\(4x-2x< -9-1\)
=>2x<-10
=>x<-5