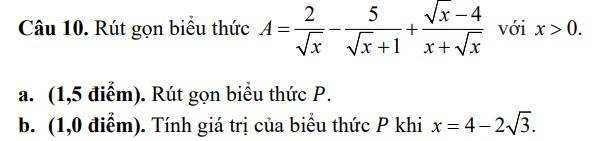Sao đề bài là biểu thức \(A\) mà câu hỏi là biểu thức \(P\) vậy bạn?
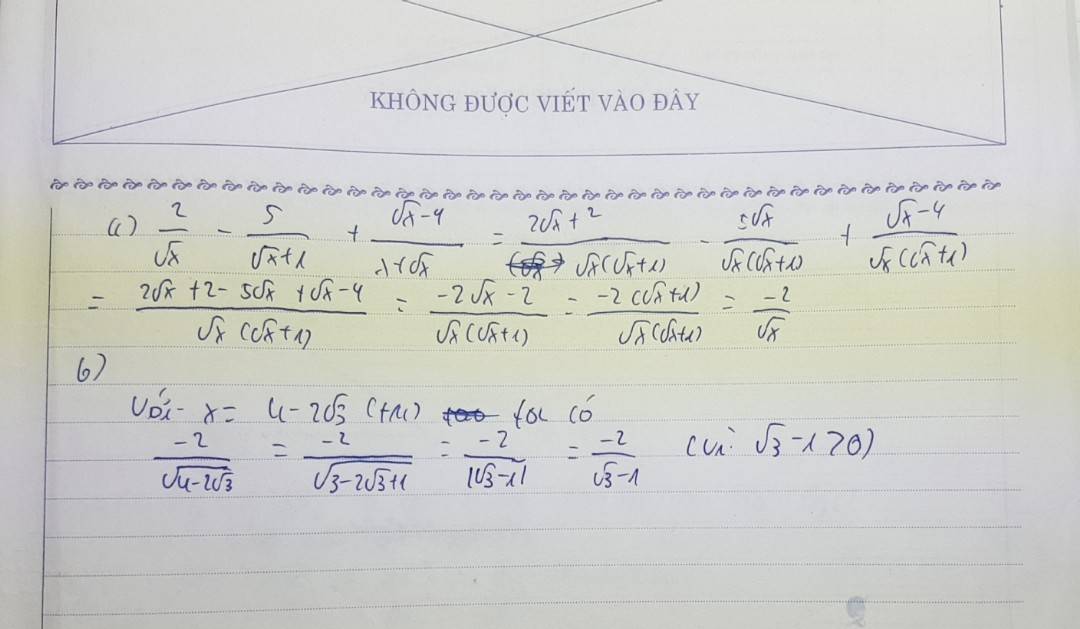
\(a,A=\dfrac{2}{\sqrt{x}}-\dfrac{5}{\sqrt{x}+1}+\dfrac{\sqrt{x}-4}{x+\sqrt{x}}\left(dk:x>0\right)\)
\(=\dfrac{2\cdot\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}-\dfrac{5\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+1\right)}+\dfrac{\sqrt{x}-4}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(=\dfrac{2\sqrt{x}+2-5\sqrt{x}+\sqrt{x}-4}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-2\sqrt{x}-2}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-2\cdot\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(=\dfrac{-2}{\sqrt{x}}\)
\(---\)
\(b,\) Ta có:
\(x=4-2\sqrt{3}\)
\(=\left(\sqrt{3}\right)^2-2\cdot\sqrt{3}\cdot1+1^2\)
\(=\left(\sqrt{3}-1\right)^2\)
Thay \(x=\left(\sqrt{3}-1\right)^2\) vào \(A\), ta có:
\(A=\dfrac{-2}{\sqrt{\left(\sqrt{3}-1\right)^2}}\)
\(=\dfrac{-2}{\left|\sqrt{3}-1\right|}\)
\(=\dfrac{-2}{\sqrt{3}-1}\)
\(Toru\)