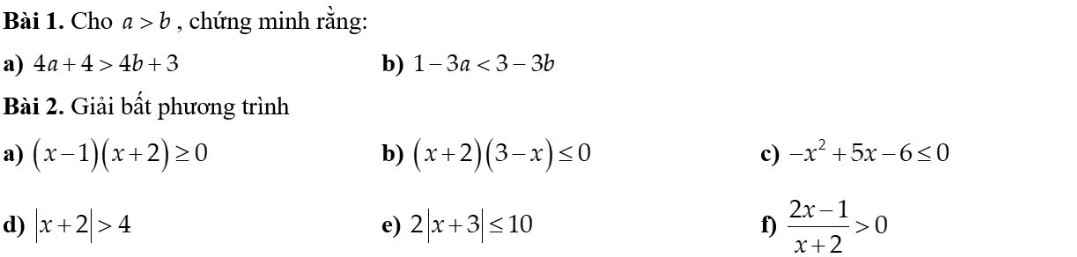Bài 1:
a: a>b
=>4a>4b
=>4a+4>4b+4
mà 4b+4>4b+3
nên 4a+4>4b+3
b: a>b
=>-3a<-3b
=>-3a+1<-3b+1
mà -3b+1<-3b+3
nên -3a+1<-3b+3
Bài 2:
a: (x-1)(x+2)>=0
TH1: \(\left\{{}\begin{matrix}x-1>=0\\x+2>=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=1\\x>=-2\end{matrix}\right.\)
=>x>=1
TH2: \(\left\{{}\begin{matrix}x-1< =0\\x+2< =0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =1\\x< =-2\end{matrix}\right.\)
=>x<=-2
b: (x+2)(3-x)<=0
=>(x+2)(x-3)>=0
TH1: \(\left\{{}\begin{matrix}x+2>=0\\x-3>=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=-2\\x>=3\end{matrix}\right.\)
=>x>=3
TH2: \(\left\{{}\begin{matrix}x+2< =0\\x-3< =0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =-2\\x< =3\end{matrix}\right.\)
=>x<=-2
c: \(-x^2+5x-6< =0\)
=>\(-\left(x-2\right)\left(x-3\right)< =0\)
=>(x-2)(x-3)>=0
TH1: \(\left\{{}\begin{matrix}x-2>=0\\x-3>=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>=2\\x>=3\end{matrix}\right.\)
=>x>=3
TH2: \(\left\{{}\begin{matrix}x-2< =0\\x-3< =0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =2\\x< =3\end{matrix}\right.\)
=>x<=2
d: |x+2|>4
=>\(\left[{}\begin{matrix}x+2>4\\x+2< -4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x>2\\x< -6\end{matrix}\right.\)
e: 2|x+3|<=10
=>|x+3|<=5
=>\(-5< =x+3< =5\)
=>-8<=x<=2
f: ĐKXĐ: x<>-2
\(\dfrac{2x-1}{x+2}>0\)
TH1: \(\left\{{}\begin{matrix}2x-1>0\\x+2>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>\dfrac{1}{2}\\x>-2\end{matrix}\right.\Leftrightarrow x>\dfrac{1}{2}\)
TH2: \(\left\{{}\begin{matrix}2x-1< 0\\x+2< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< \dfrac{1}{2}\\x< -2\end{matrix}\right.\Leftrightarrow x< -2\)