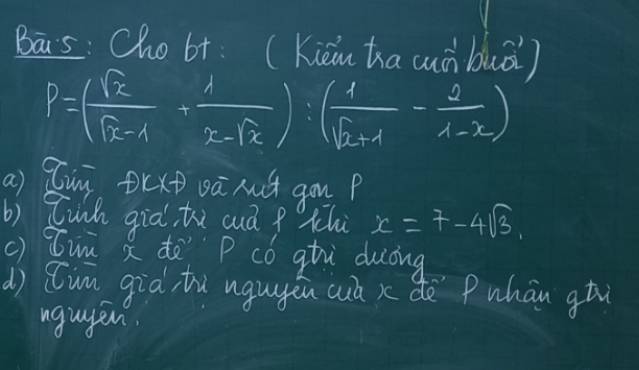a) ĐKXĐ: \(x>0;x\ne1\)
\(P=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}+\dfrac{1}{x-\sqrt{x}}\right):\left(\dfrac{1}{\sqrt{x}+1}-\dfrac{2}{1-x}\right)\)
\(=\left[\dfrac{x}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right]:\left[\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right]\)
\(=\dfrac{x+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{1}{\sqrt{x}-1}\)
\(=\dfrac{x+1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\left(\sqrt{x}-1\right)=\dfrac{x+1}{\sqrt{x}}\)
b) Với \(x=7-4\sqrt{3}=\left(\sqrt{3}\right)^2-2\cdot\sqrt{3}\cdot2+2^2=\left(\sqrt{3}-2\right)^2\), thay vào P, ta được:
\(P=\dfrac{7-4\sqrt{3}+1}{\sqrt{\left(\sqrt{3}-2\right)^2}}=\dfrac{8-4\sqrt{3}}{\left|\sqrt{3}-2\right|}=\dfrac{4\left(2-\sqrt{3}\right)}{2-\sqrt{3}}=4\)
c) Để P có giá trị dương thì \(\dfrac{x+1}{\sqrt{x}}>0\)
\(\Leftrightarrow x+1>0\) (vì \(\sqrt{x}>0;\forall x>0\))
\(\Leftrightarrow x>-1\)
Kết hợp với ĐKXĐ của x, ta được: \(x>0;x\ne1\)
d) Có: \(P=\dfrac{x+1}{\sqrt{x}}\)
Để P nhận giá trị nguyên thì \(x+1⋮\sqrt{x}\)
\(\Rightarrow x+1-\sqrt{x}\cdot\sqrt{x}⋮\sqrt{x}\)
\(\Rightarrow1⋮\sqrt{x}\)
\(\Rightarrow\sqrt{x}\inƯ\left(1\right)\)
\(\Rightarrow\sqrt{x}\in\left\{1;-1\right\}\)
Mà \(\sqrt{x}>0;\forall x>0\) nên \(\sqrt{x}=1\Leftrightarrow x=1\) (ktm ĐKXĐ)
Vậy không tìm được giá trị nguyên nào của x để P nhận giá trị nguyên
\(\text{#}Toru\)