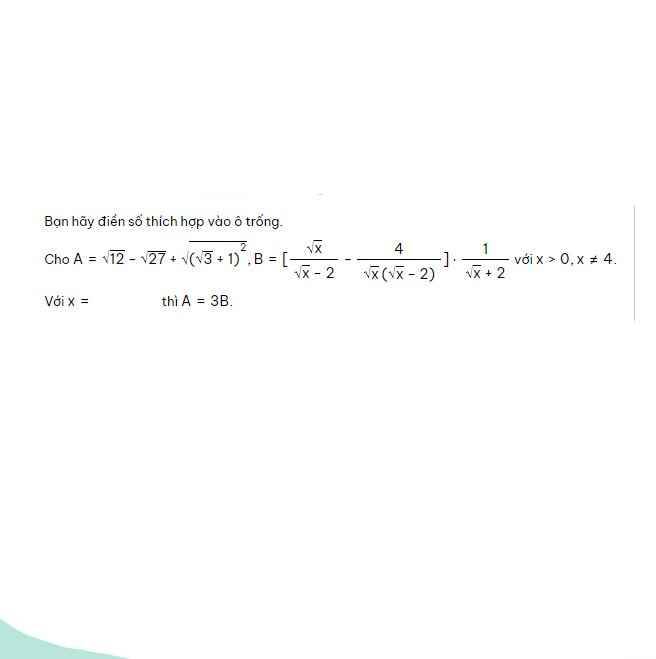
\(A=\sqrt{12}-\sqrt{27}+\sqrt{\left(\sqrt{3}+1\right)^2}\)
\(=2\sqrt{3}-3\sqrt{3}+\left|\sqrt{3}+1\right|\)
\(=-\sqrt{3}+\sqrt{3}+1=1\)
\(B=\left(\dfrac{\sqrt{x}}{\sqrt{x}-2}-\dfrac{4}{\sqrt{x}\left(\sqrt{x}-2\right)}\right)\cdot\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{x-4}{\sqrt{x}\left(\sqrt{x}-2\right)}\cdot\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\sqrt{x}\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{1}{\sqrt{x}}\)
A=3B
=>\(1=\dfrac{3}{\sqrt{x}}\)
=>\(\sqrt{x}=3\)
=>x=9
Đúng 0
Bình luận (0)
