\(10)a)\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{x\sqrt{x}-1}\right)\dfrac{3\sqrt{x}-3}{x+\sqrt{x}}.ĐKXĐ:x>0;x\ne1\)
\(=\left(\dfrac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{1}{\left(\sqrt{x}\right)^3-1}\right)\dfrac{3\sqrt{x}-3}{x+\sqrt{x}}\)
\(=\dfrac{x+\sqrt{x}+1-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}.\dfrac{3\left(\sqrt{x}-1\right)}{x+\sqrt[]{x}}\)
\(=\dfrac{x+\sqrt{x}}{x+\sqrt{x}+1}.\dfrac{3}{x+\sqrt{x}}\)
\(=\dfrac{3}{x+\sqrt{x}+1}\)
b) Ta thấy \(M=\dfrac{3}{x+\sqrt{x}+1}>0\) với mọi x >0
Lại có \(M< 3\Leftrightarrow\dfrac{3}{x+\sqrt{x}+1}< 3\Leftrightarrow x+\sqrt{x}+1>1\)
\(\Leftrightarrow x+\sqrt{x}>0\Leftrightarrow\sqrt{x}\left(\sqrt{x}+1\right)>0\) (luôn đúng) \(.ĐểM\in Z\Leftrightarrow0< M< 3\Rightarrow M\in\left\{1;2\right\}\)
\(TH1:\dfrac{3}{x+\sqrt{x}+1}=1\)
\(\Leftrightarrow x+\sqrt{x}+1=3\Leftrightarrow x+\sqrt{x}-2=0\)
\(\Leftrightarrow\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)=0\)
\(\Leftrightarrow\sqrt{x}-1=0\)(Vì x>0 nên căn x+2>0)
\(\Leftrightarrow x=1\left(tm\right)\)
\(TH2:\dfrac{3}{x+\sqrt{x}+1}=2\)
\(\Leftrightarrow2x+2\sqrt{x}+2=3\Leftrightarrow2x+2\sqrt{x}-1=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{-1+\sqrt{3}}{2}< 0\left(loại\right)\\x=\dfrac{-1-\sqrt{3}}{2}< 0\left(l\right)\end{matrix}\right.\)
Vậy, với x=1 thì M thuộc Z
9:
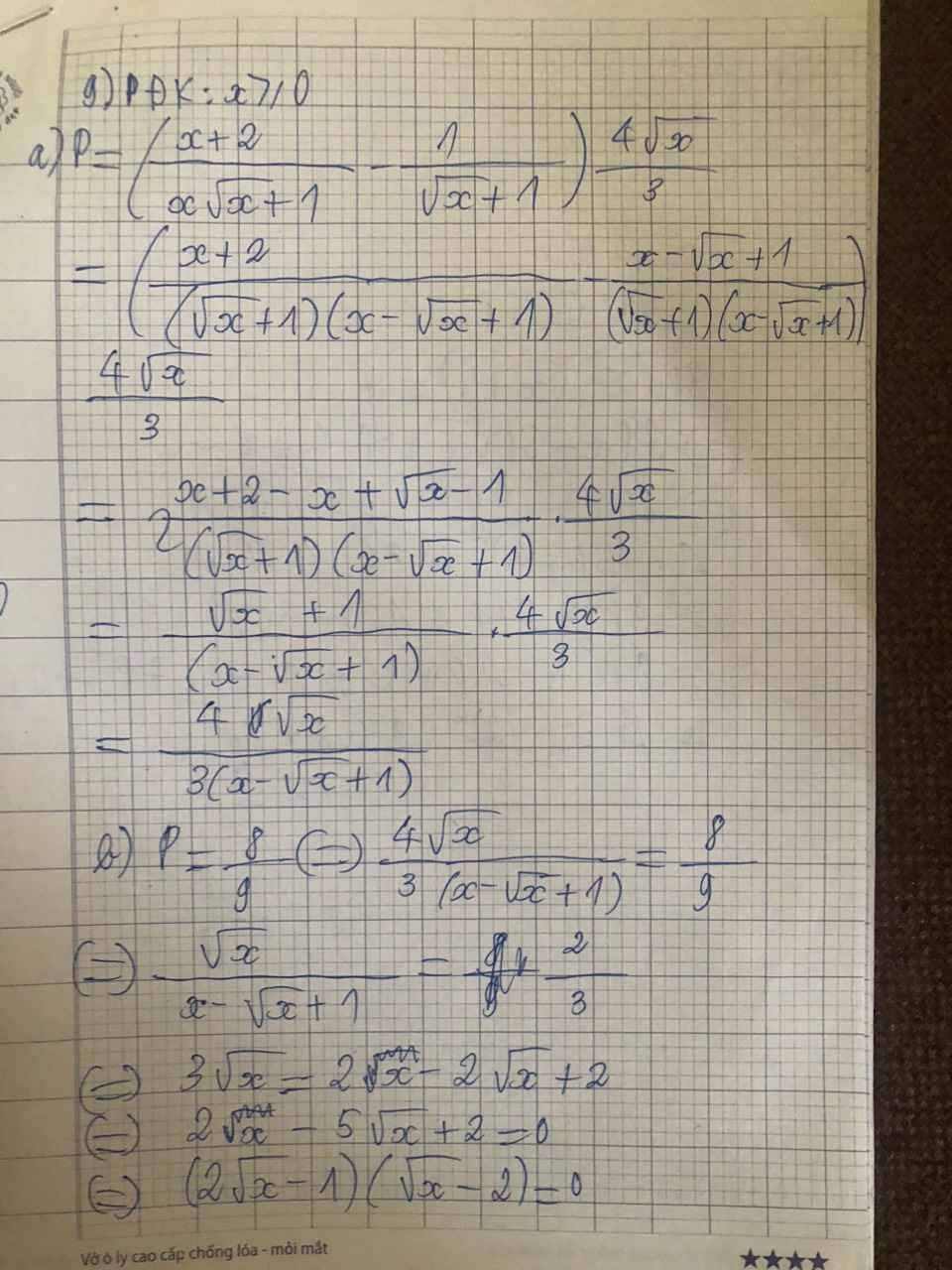
a: \(P=\dfrac{x+2-x+\sqrt{x}-1}{\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}\cdot\dfrac{4\sqrt{x}}{3}\)
\(=\dfrac{4\sqrt{x}}{3\left(x-\sqrt{x}+1\right)}\)
10:
a: \(M=\dfrac{x+\sqrt{x}+1-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{3\left(\sqrt{x}-1\right)}{x+\sqrt{x}}\)
\(=\dfrac{3}{x+\sqrt{x}+1}\)
b: M nguyên
=>x+căn x+1 thuộc Ư(3)
=>x+căn x+1=1 hoặc x+căn x+1=3
=>x+căn x-2=0
=>(căn x-1)(căn x+2)=0
=>căn x-1=0
=>x=1(loại)