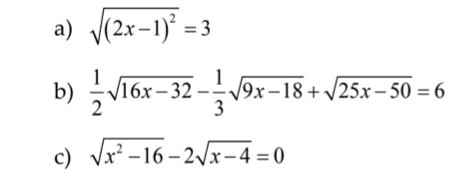a, \(\sqrt{\left(2x-1\right)^2}=3\Leftrightarrow\left|2x-1\right|=3\)
TH1 : 2x - 1 = 3 <=> x = 2
TH2 : 2x - 1 = -3 <=> x = -1
b, đk : x>= 2
PT <=> \(2\sqrt{x-2}-\sqrt{x-2}+5\sqrt{x-2}=6\)
\(\Leftrightarrow6\sqrt{x-2}=6\Leftrightarrow\sqrt{x-2}=1\Leftrightarrow x-2=1\Leftrightarrow x=3\)( tm )
c, \(\sqrt{x^2-16}-2\sqrt{x-4}=0\)đk : x >= 4 ; x =< -4
\(\Leftrightarrow\sqrt{x-4}\left(\sqrt{x+4}-2\right)=0\)
TH1 : x = 4( tm )
TH2 : \(x+4=4\Leftrightarrow x=0\)( loại )
\(a,=6\sqrt{2}-7\sqrt{2}+8\sqrt{2}=7\sqrt{2}\\ b,=\sqrt{\left(\sqrt{5}-\sqrt{3}\right)^2}+\sqrt{\left(\sqrt{3}+1\right)^2}\\ =\sqrt{5}-\sqrt{3}+\sqrt{3}+1=\sqrt{5}-1\\ c,=6\sqrt{3}-4\sqrt{3}+3\sqrt{3}=5\sqrt{3}\\ d,=\dfrac{2\sqrt{5}+2\sqrt{2}+2\sqrt{5}-2\sqrt{2}}{\left(\sqrt{5}-\sqrt{2}\right)\left(\sqrt{5}+\sqrt{2}\right)}=\dfrac{4\sqrt{5}}{3}\)
\(2,\\ a,\Leftrightarrow\left|2x-1\right|=3\Leftrightarrow\left[{}\begin{matrix}2x-1=3\\2x-1=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\\ b,ĐK:x\ge2\\ PT\Leftrightarrow\dfrac{1}{2}\cdot4\sqrt{x-2}-\dfrac{1}{3}\cdot3\sqrt{x-2}+5\sqrt{x-2}=6\\ \Leftrightarrow6\sqrt{x-2}=6\Leftrightarrow\sqrt{x-2}=1\\ \Leftrightarrow x-2=1\Leftrightarrow x=3\left(tm\right)\\ c,ĐK:x\ge4\\ PT\Leftrightarrow\sqrt{x-4}\left(\sqrt{x+4}-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-4=0\\x-4=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=4\left(tm\right)\\x=8\left(tm\right)\end{matrix}\right.\)