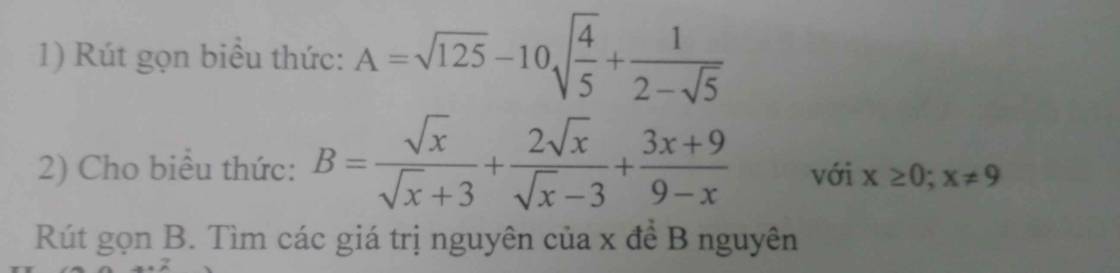1: \(A=\sqrt{125}-10\sqrt{\dfrac{4}{5}}+\dfrac{1}{2-\sqrt{5}}\)
\(=5\sqrt{5}-10\cdot\dfrac{2}{\sqrt{5}}-\dfrac{1}{\sqrt{5}-2}\)
\(=5\sqrt{5}-4\sqrt{5}-\left(\sqrt{5}+2\right)\)
\(=\sqrt{5}-\sqrt{5}-2=-2\)
2: \(B=\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{2\sqrt{x}}{\sqrt{x}-3}+\dfrac{3x+9}{9-x}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{2\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)+2\sqrt{x}\left(\sqrt{x}+3\right)-3x-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{x-3\sqrt{x}+2x+6\sqrt{x}-3x-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}=\dfrac{3\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{3}{\sqrt{x}+3}\)
Để B nguyên thì \(3⋮\sqrt{x}+3\)
=>\(\sqrt{x}+3\in\left\{1;-1;3;-3\right\}\)
=>\(\sqrt{x}=0\)
=>x=0(nhận)