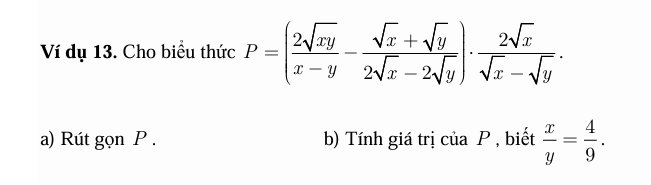
a, \(P=\left(\dfrac{2\sqrt{xy}}{x-y}-\dfrac{\sqrt{x}+\sqrt{y}}{2\sqrt{x}-2\sqrt{y}}\right).\dfrac{2\sqrt{x}}{\sqrt{x}-\sqrt{y}}\)
đk x;y >= 0
\(P=\left(\dfrac{4\sqrt{xy}-\left(\sqrt{x}+\sqrt{y}\right)^2}{2\left(x-y\right)}\right).\dfrac{2\sqrt{x}}{\sqrt{x}-\sqrt{y}}\)
\(=\left(\dfrac{-x-y+2\sqrt{xy}}{2\left(x-y\right)}\right).\dfrac{2\sqrt{x}}{\sqrt{x}-\sqrt{y}}=\dfrac{-2\sqrt{x}\left(\sqrt{x}-\sqrt{y}\right)^2}{2\left(x-y\right)\left(\sqrt{x}-\sqrt{y}\right)}=\dfrac{-\sqrt{x}}{\sqrt{x}+\sqrt{y}}\)
Ta có \(\dfrac{x}{y}=\dfrac{4}{9}\Rightarrow y=\dfrac{9x}{4}\)
\(P=\dfrac{-\sqrt{x}}{\sqrt{x}+\dfrac{3}{2}\sqrt{x}}=\dfrac{-1}{1+\dfrac{3}{2}}=-\dfrac{2}{5}\)