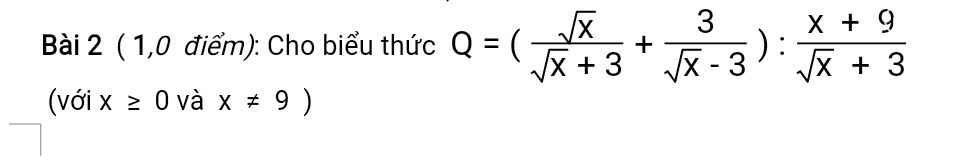`b)(x+1)/(x^2+x+1)-(x-1)/(x^2-x+1)=(2(x+2)^2)/(x^6-1)` `(ĐK:x<>1)`
`<=>((x+1)(x-1))/((x-1)(x^2+x+1))-((x-1)(x+1))/((x+1)(x^2-x+1))=(2(x+2)^2)/(x^6-1)`
`<=>(x^2-1)/(x^3-1)-(x^2-1)/(x^3+1)=(2(x+2)^2)/(x^6-1)`
`<=>((x^2-1)(x^3+1))/(x^6-1)-((x^2-1)(x^3-1))/(x^6-1)=(2(x+2)^2)/(x^6-1)`
`<=>(x^2-1)(x^3+1)-(x^2-1)(x^3-1)=2(x+2)^2`
`<=>x^5+x^2-x^3-1-x^5+x^2+x^3-1=2(x^2+4x+4)`
`<=>2x^2-2=2x^2+8x+8`
`<=>-2=8x+8`
`<=>8x=-10`
`<=>x=-10/8=-5/4(tm)`
\(a,\dfrac{1}{3-x}-\dfrac{1}{x+1}=\dfrac{x}{x-3}-\dfrac{\left(x-1\right)^2}{x^2-2x-3}\left(ĐKXĐ:x\ne3;x\ne-1\right)\\ \dfrac{1}{3-x}-\dfrac{1}{x+1}=\dfrac{x}{x-3}-\dfrac{\left(x-1\right)^2}{\left(x-3\right)\left(x+1\right)}\\ \dfrac{1}{\left(3-x\right)\left(x+1\right)}-\dfrac{3-x}{\left(3-x\right)\left(x+1\right)}=\dfrac{-x\left(x+1\right)}{\left(3-x\right)\left(x+1\right)}+\dfrac{\left(x-1\right)^2}{\left(3-x\right)\left(x+1\right)}\\ 1-3+x=-x^2-x+x^2-2x+1\\ x-2=1-3x\\ x+3x=1+2\\ 4x=3\\ x=\dfrac{3}{4}\left(T/m\right)\)
Vậy phương trình có nghiệm `x=3/4`