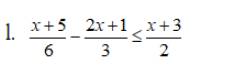\(\dfrac{x+5}{6}-\dfrac{2x+1}{3}\le\dfrac{x+3}{2}\\ \Leftrightarrow\dfrac{x+5}{6}-\dfrac{\left(2x+1\right)2}{3.2}\le\dfrac{\left(x+3\right)3}{2.3}\\ \Leftrightarrow x+5-4x-2\le3x+9\\ \Leftrightarrow x-4x-3x\le9-5+2\\ \Leftrightarrow-6x\le6\\ \Leftrightarrow x\ge-1\)
Vậy bất phương trình có tập nghiệm là: \(S=\left\{x|x\ge1\right\}\)
Biểu diễn
\(\dfrac{x+5}{6}-\dfrac{2x+1}{3}\le\dfrac{x+3}{2}\)
\(\Leftrightarrow\dfrac{x+5}{6}-\dfrac{2\left(2x+1\right)}{6}\le\dfrac{3\left(x+3\right)}{6}\)
\(\Leftrightarrow x+5-2\left(2x+1\right)\le3\left(x+3\right)\)
\(\Leftrightarrow x+5-4x-2\le3x+9\)
\(\Leftrightarrow x+5-4x-2-3x-9\le0\)
\(\Leftrightarrow-6x-6\le0\)
\(\Leftrightarrow-6x\le6\)
\(\Leftrightarrow x\ge-1\)
\(S=\left\{x/x\ge-1\right\}\)
Trục số:

x+5/6 -2x+1/3≤x+5/2
⇔x+5/6 -(2x+1)2/6 ≤ (x+5)3/6
⇔x+5-4x+2 ≤ 3x+15
⇔x-4x-3x≤15-5-2
⇔-6x≤ 8
⇔x≥8:(-6)
⇔x≥-4/3
vậy S={x]x≥-4/3}
biểu diễn tập nghiệm trên trục số
////////-4/3-------------0--------------------------------------------->