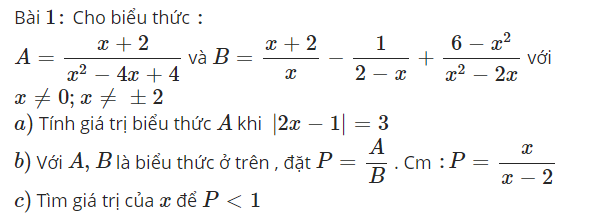Question

Answer 1

a: |2x-1|=3

=>2x-1=3 hoặc 2x-1=-3

=>2x=4 hoặc 2x=-2

=>x=2(loại) hoặc x=-1(nhận)

Thay x=-1 vào A, ta được: 

\(A=\dfrac{-1+2}{\left(-1-2\right)^2}=\dfrac{1}{9}\)

b: \(P=\dfrac{x+2}{\left(x-2\right)^2}:\dfrac{x^2-4+x+6-x^2}{x\left(x-2\right)}\)

\(=\dfrac{x+2}{\left(x-2\right)^2}\cdot\dfrac{x\left(x-2\right)}{x+2}=\dfrac{x}{x-2}\)

c: Để P<1 thì P-1<0

=>(x-x+2)/(x-2)<0

=>x-2<0

hay x<2