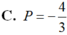\(m^2x-m=4x-2\)
\(\Leftrightarrow m^2x-4x=-2+m\)
\(\Leftrightarrow x\left(m^2-4\right)=-2+m-vô-nghiệm\)
\(\Leftrightarrow\left\{{}\begin{matrix}m^2-4=0\\-2+m\ne0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m=\pm2\\m\ne2\end{matrix}\right.\Rightarrow m=-2\)
\(x^2-\left(2m+5\right)x+m^2+4=0\)
\(a,có-nghiệm\Leftrightarrow\Delta\ge0\) \(\Leftrightarrow\left(2m+5\right)^2-4\left(m^2+4\right)\ge0\Leftrightarrow m\ge-\dfrac{9}{20}\)
\(b,\Leftrightarrow\left\{{}\begin{matrix}\Delta>0\\S>0\\P>0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m>-\dfrac{9}{20}\\x1+x2>0\\x1x2>0\\\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}m>-\dfrac{9}{20}\\2m+5>0\Leftrightarrow m>-\dfrac{5}{2}\\m^2+4>0\left(luôn-đúng\right)\\\end{matrix}\right.\)
\(\Rightarrow m>-\dfrac{9}{20}\)
\(c,x1^2+x2^2=\left(x1+x2\right)^2-2x1x2=\left(2m+5\right)^2-2\left(m^2+4\right)=.......\)
Bài 3: Biến đổi pt ta được : ( m2 - 4 )x = m -2.
Xét m2 - 4 = 0 => m=2, m=-2 .
Th1: m = 2 => pt có vô số nghiệm ( loại) .
Th2: m =-2 => 0x = -4 => pt vô nghiệm (tm)
Vậy m = -2 thì pt vô nghiệm.
Bài 3:
\(PT\Leftrightarrow x\left(m^2-4\right)=m-2\)
PT vô nghiệm \(\Leftrightarrow\left\{{}\begin{matrix}m^2-4=0\\m-2\ne0\end{matrix}\right.\Leftrightarrow m=-2\)
Bài 4:
\(a,\) PT có nghiệm \(\Leftrightarrow\Delta=\left(2m+5\right)^2-4\left(m^2+4\right)\ge0\)
\(\Leftrightarrow20m+9\ge0\\ \Leftrightarrow m\ge-\dfrac{9}{20}\)
\(b,\) Viét: \(\left\{{}\begin{matrix}x_1+x_2=2m+5\\x_1x_2=m^2+4\end{matrix}\right.\)
PT có 2 nghiệm dương pb \(\Leftrightarrow\left\{{}\begin{matrix}\Delta>0\\2m+5>0\\m^2+4>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m>-\dfrac{9}{20}\\2m+5>0\\m\in R\end{matrix}\right.\Leftrightarrow m>-\dfrac{5}{2}\)
\(c,x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\\ =\left(2m+5\right)^2-2\left(m^2+4\right)\\ =4m^2+20m+25-2m^2-8\\ =2m^2+20m+17\)