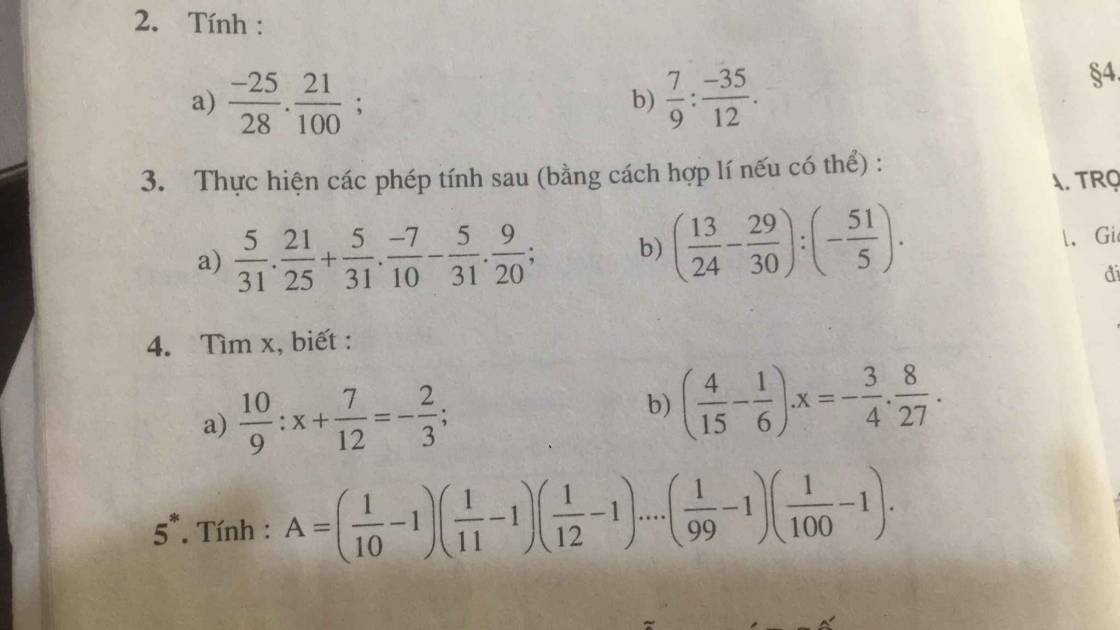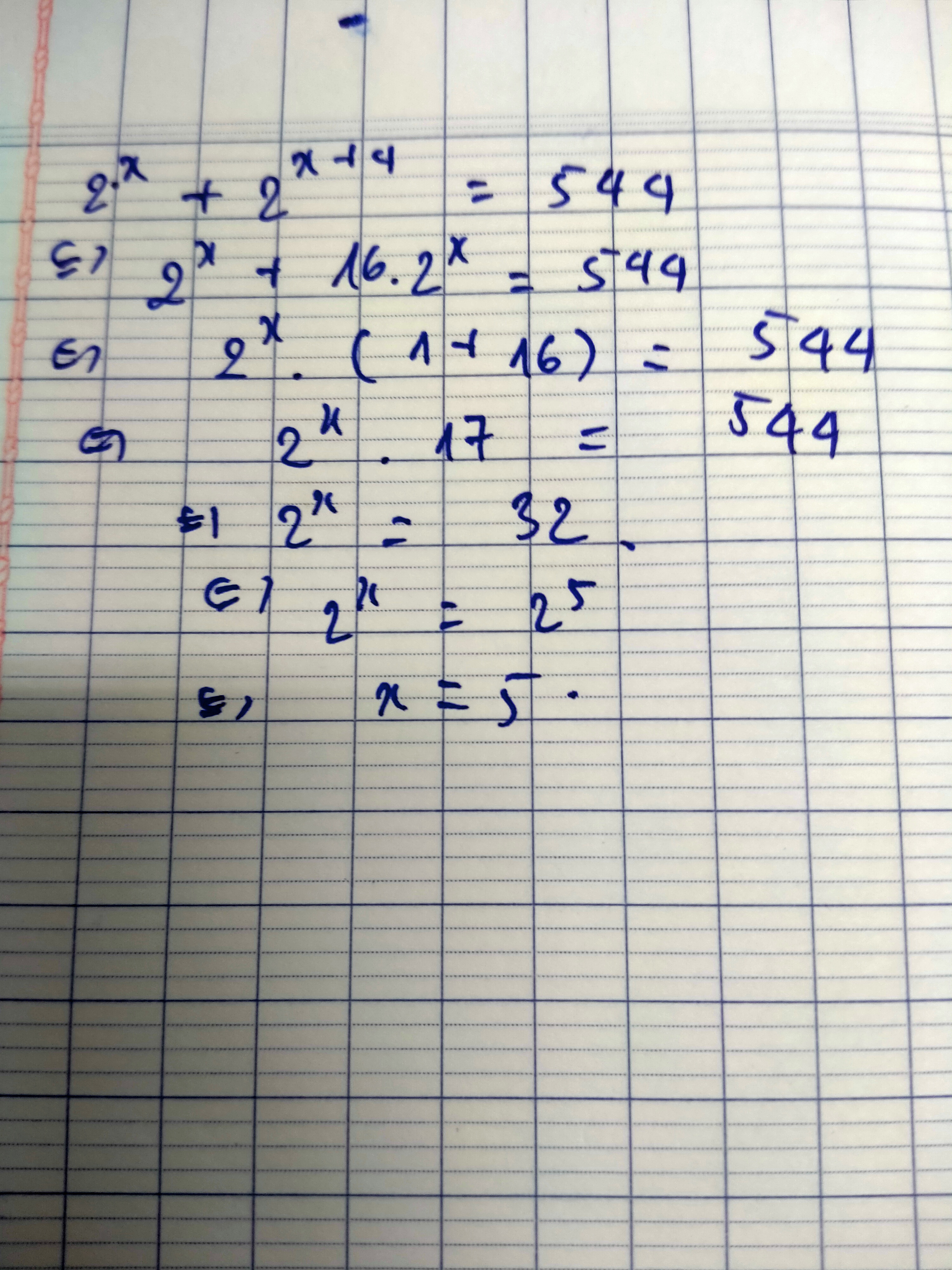\(\left(4x^2-3\right)^3+8=0\)
Bài 3: Nhân, chia số hữu tỉ
\(\left(4x^2-3\right)^3+8=0\)
\(\left(4x^2-3\right)^3=-8\\ =>4x^2-3=-2\)
\(4x^2=1\\ x^2=\dfrac{1}{4}=>\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Đúng 2
Bình luận (0)
Bài 2:
a)
\(\dfrac{-25}{28}\)x\(\dfrac{21}{100}\)
=\(\dfrac{-525}{2800}\)=\(\dfrac{-3}{16}\)
b)
\(\dfrac{7}{9}\):\(\dfrac{-35}{12}\)
=\(\dfrac{7}{9}\)x\(\dfrac{-12}{35}\)
=\(\dfrac{-84}{315}\)=\(\dfrac{-4}{15}\)
Đúng 6
Bình luận (0)
A= 52/x+3
B=x-2/x+3
C=2x+1/x-3
Si giúp mình với ạ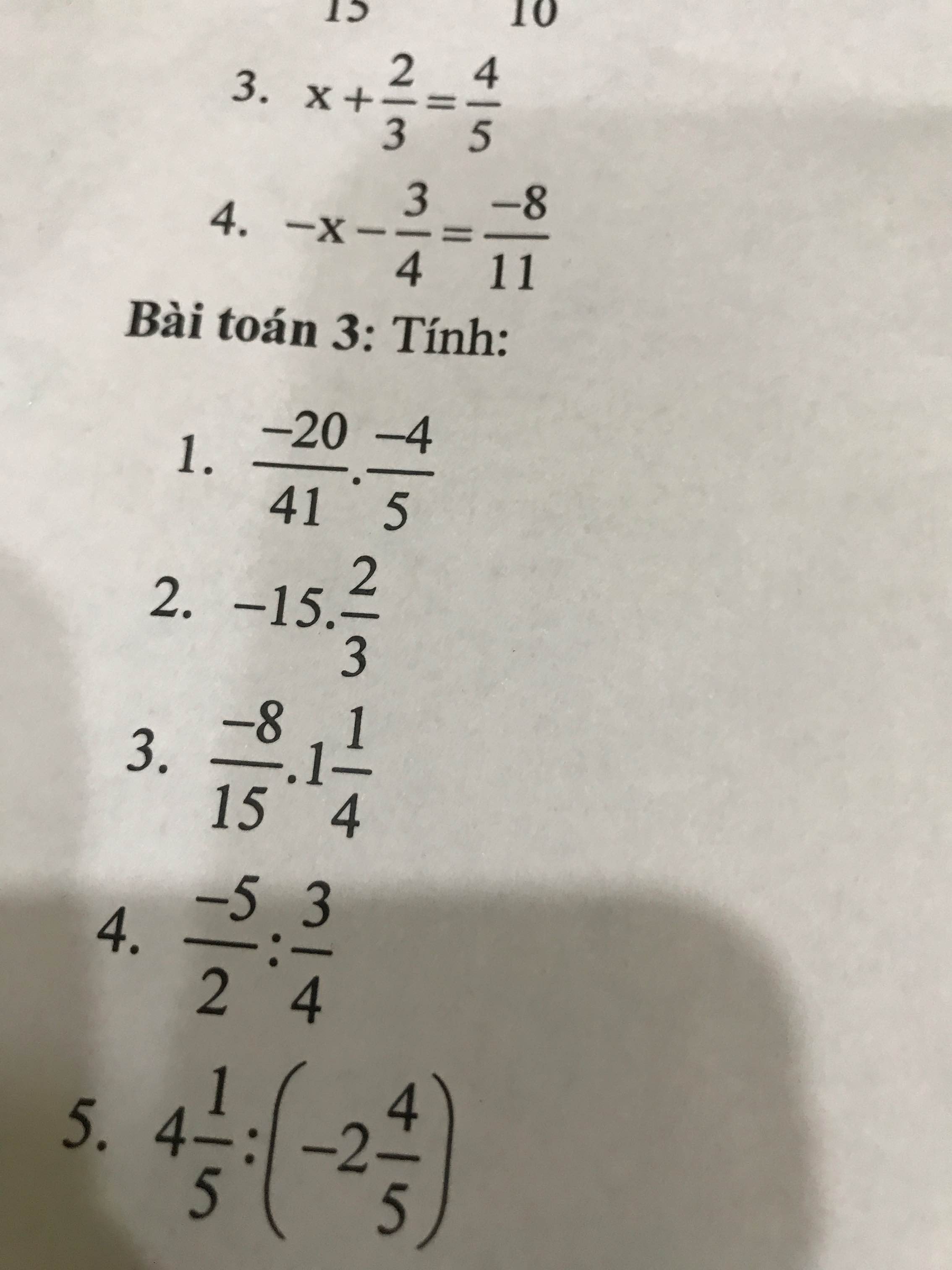

\(25\dfrac{3}{19}:\left(-\dfrac{5}{4}\right)-35\dfrac{3}{19}:\left(-\dfrac{5}{4}\right)\)
=-4/5(25+3/19-35-3/19)
=-4/5*(-10)
=8
Đúng 2
Bình luận (0)
\(25\dfrac{3}{19}:\left(-\dfrac{5}{4}\right)-35\dfrac{3}{19}:\left(-\dfrac{5}{4}\right)\\ =\left(25\dfrac{3}{19}-35\dfrac{3}{19}\right):\left(-\dfrac{5}{4}\right)\\ =-10:\left(-\dfrac{5}{4}\right)\\ =8\)
Đúng 2
Bình luận (0)
2^x + 2^x+4 =544
\(2^x+2^{x+4}=544\\\Rightarrow2^x\cdot1+2^x\cdot2^4=544\\\Rightarrow2^x\cdot(1+2^4)=544\\\Rightarrow2^x\cdot(1+16)=544\\\Rightarrow2^x\cdot17=544\\\Rightarrow2^x=544:17\\\Rightarrow2^x=32\\\Rightarrow2^x=2^5\\\Rightarrow x=5\)
Đúng 2
Bình luận (0)
\(2^x+2^{x+4}=544\)
=>\(2^x+2^x\cdot2^4=544\)
=>\(2^x\left(1+2^4\right)=544\)
=>\(2^x\cdot17=544\)
=>\(2^x=\dfrac{544}{17}=32\)
=>\(2^x=2^5\)
=>x=5
Đúng 2
Bình luận (0)
(2x-3)x(3/4x+1)=0
(x-5) mũ 2 nhân x = x-5
(x-5)^2*x=(x-5)
=>(x-5)[x(x-5)-1]=0
=>(x-5)(x^2-5x-1)=0
=>x=5 hoặc x^2-5x-1=0
=>\(x\in\left\{5;\dfrac{5\pm\sqrt{29}}{2}\right\}\)
Đúng 1
Bình luận (0)
tìm xϵZ để biểu thức Aϵz,BϵZ
A=\(\dfrac{x+3}{x-2}\) B=\(\dfrac{1-2x}{x+3}\)
giải chi tiết giúp m ạ
a> Để A là số nguyên thì x-2+5 chia hết cho x-2
=>\(x-2\in\left\{1;-1;5;-5\right\}\)
hay \(x\in\left\{3;1;7;-3\right\}\)
b: Để B là số nguyên thì -2x-6+7 chia hết cho x+3
=>\(x+3\in\left\{1;-1;7;-7\right\}\)
hay \(x\in\left\{-2;-4;4;-10\right\}\)
Đúng 1
Bình luận (0)
C = 2/(-2021).(-2019) + 2/(-2019).(-2017) + 2/(-2017).(-2015) +...+ 2/(-5).(-3) + 2/(-3).(-1)
`C=1/(-2021) -1/(-2019) +1/(-2019) -1/(-2017) +...+1/(-3) -1/(-1)`
`C=(-1)/2021 +1=2022/2021`
Đúng 1
Bình luận (0)