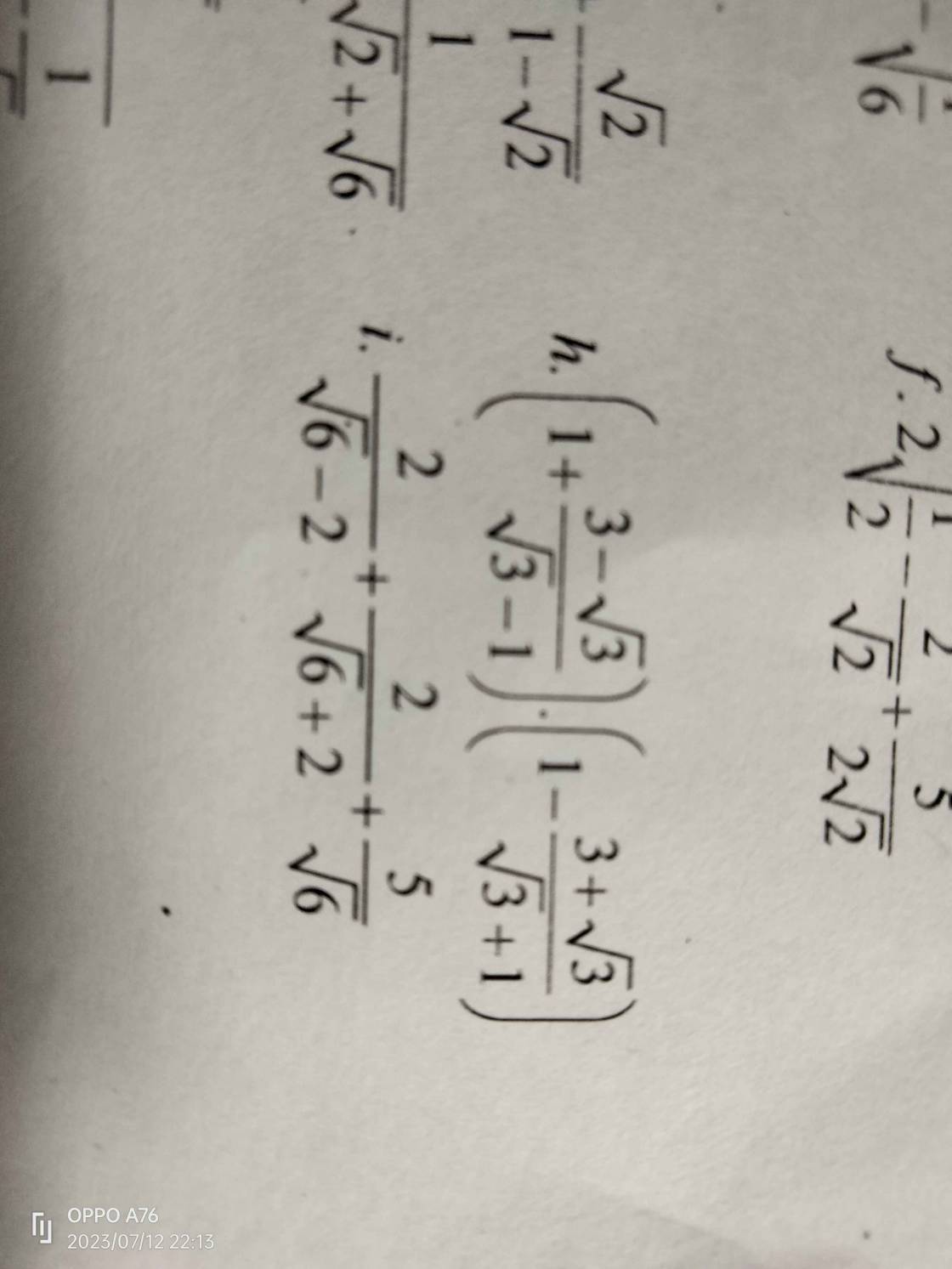\(c,\left\{{}\begin{matrix}\dfrac{2}{x-2}+\dfrac{1}{y+1}=3\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{4}{x-2}+\dfrac{2}{y+1}=6\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2}{y+1}+\dfrac{3}{y+1}=5\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{5}{y+1}=5\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y+1=1\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=0\left(2\right)\\\dfrac{4}{x-2}-\dfrac{3}{y+1}=1\left(1\right)\end{matrix}\right.\)
Thay \(\left(2\right)\) vào \(\left(1\right)\) :
\(\dfrac{4}{x-2}-\dfrac{3}{0+1}=1\)
\(\Rightarrow\dfrac{4}{x-2}-3=1\)
\(\Rightarrow\dfrac{4}{x-2}=4\)
\(\Rightarrow x-2=1\)
\(\Rightarrow x=3\)
Vậy hệ phương trình có nghiệm duy nhất \(\left(x;y\right)=\left(3;0\right)\)
c: =>4/x-2+2/y+1=6 và 4/x-2-3/y+1=1
=>5/y+1=5 và 2/x-2+1/y+1=3
=>y+1=1 và 2/x-2+1=3
=>y=0 và x-2=1
=>x=3 và y=0