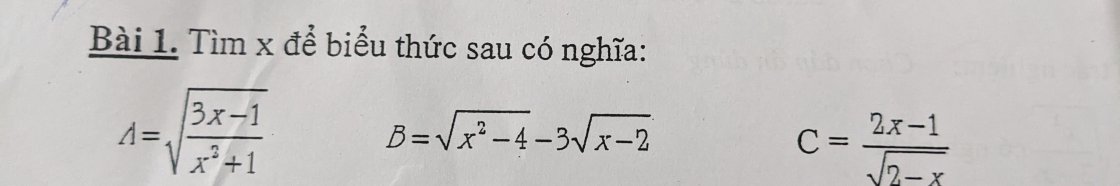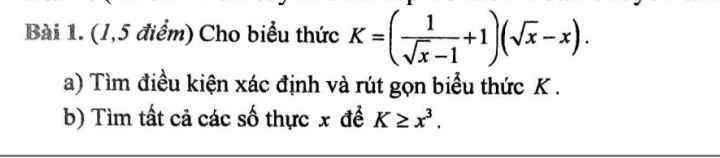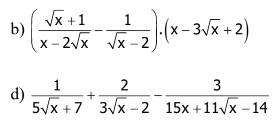\(A=\sqrt{\dfrac{3x-1}{x^2+1}}\)
Có nghĩa khi:
\(\dfrac{3x-1}{x^2+1}\ge0\)
\(\Leftrightarrow3x-1\ge0\)
\(\Leftrightarrow3x\ge1\)
\(\Leftrightarrow x\ge\dfrac{1}{3}\)
Vậy A có nghĩa khi \(x\ge\dfrac{1}{3}\)
_________________________
\(B=\sqrt{x^2-4}-3\sqrt{x-2}\)
Có nghĩa khi:
\(\left\{{}\begin{matrix}x^2-4\ge0\\x-2\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2\ge4\\x\ge2\end{matrix}\right.\)
\(\Leftrightarrow x\ge2\)
Vậy B có nghĩa khi \(x\ge2\)
______________________
\(C=\dfrac{2x-1}{\sqrt{2-x}}\)
Có nghĩa khi:
\(\left\{{}\begin{matrix}2-x\ge0\\2-x\ne0\end{matrix}\right.\)
\(\Leftrightarrow2-x>0\)
\(\Leftrightarrow x< 2\)
Vậy C có nghĩa khi \(x< 2\)
a: ĐKXĐ: (3x-1)/(x^2+1)>=0
=>3x-1>=0
=>x>=1/3
b: ĐKXĐ: x^2-4>=0 và x-2>=0
=>x>=2
c: ĐKXĐ: 2-x>0
=>x<2