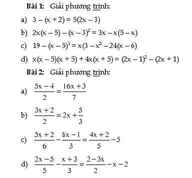a/
\(\Leftrightarrow3-x-2-\left(10x-15\right)=0\)
\(\Leftrightarrow3-x-2-10x+15=0\)
\(16-11x=0\)
\(\Leftrightarrow x=\dfrac{16}{11}\)
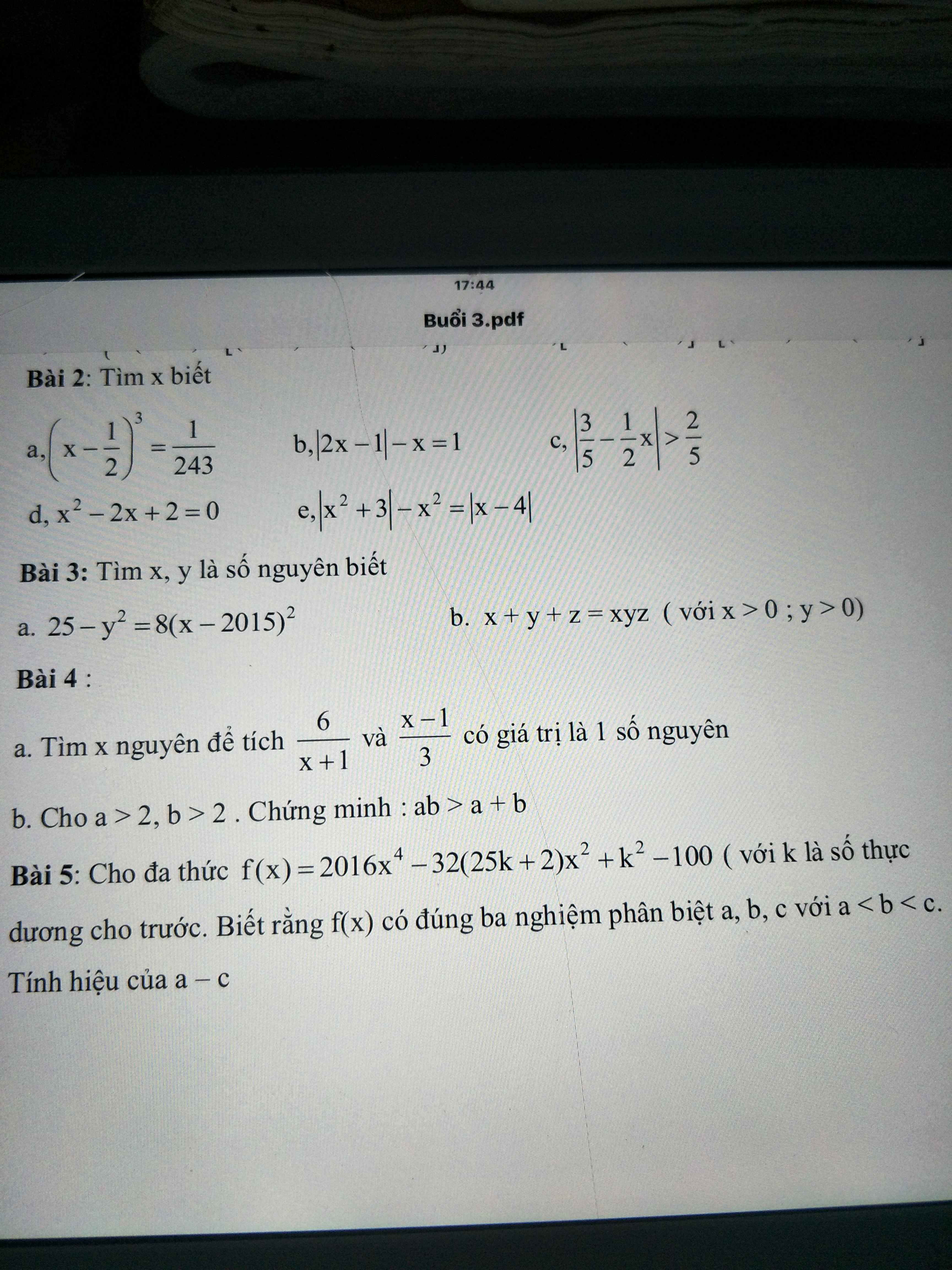
d: \(\Leftrightarrow2x^2-10x-x^2+6x-9-3x+5x-x^2=0\)
=>-2x-9=0
=>-2x=9
hay x=-9/2
c: \(\Leftrightarrow19-x^3+15x^2-75x+125=x\left(3-x^2-24x+144\right)\)
\(\Leftrightarrow-x^3+15x^2-75x+144-3x+x^3+24x^2-144x=0\)
\(\Leftrightarrow39x^2-222x+144=0\)
\(\Delta=\left(-222\right)^2-4\cdot39\cdot144=26820>0\)
Do đó: Phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{222-6\sqrt{745}}{78}=\dfrac{37-\sqrt{745}}{13}\\x_2=\dfrac{37+\sqrt{145}}{13}\end{matrix}\right.\)
b/
\(\Leftrightarrow2x\left(x-5\right)-\left(x-3\right)^2-3x-x\left(x-5\right)=0\)
\(\Leftrightarrow2x\left(x-5\right)-x\left(x-5\right)-\left(x-3\right)^2-3x=0\)
\(\Leftrightarrow x^2-5x-\left(x^2-6x+9\right)-3x=0\)
\(\Leftrightarrow x^2-5x-x^2+6x-9-3x=0\)
\(\Leftrightarrow-2x-9=0\)
\(\Leftrightarrow x=-\dfrac{9}{2}\)