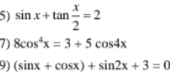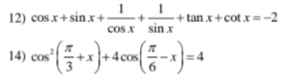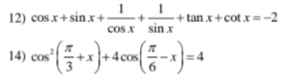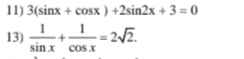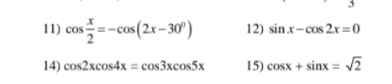a.
\(sinx=2sin\dfrac{x}{2}cos\dfrac{x}{2}=\dfrac{2sin\dfrac{x}{2}.cos\dfrac{x}{2}}{sin^2\dfrac{x}{2}+cos^2\dfrac{x}{2}}=\dfrac{2tan\dfrac{x}{2}}{1+tan^2\dfrac{x}{2}}\)
Pt tương đương: \(\dfrac{2tan\dfrac{x}{2}}{1+tan^2\dfrac{x}{2}}+tan\dfrac{x}{2}=2\)
Đặt \(tan\dfrac{x}{2}=t\) ta được:
\(\dfrac{2t}{1+t^2}+t=2\Leftrightarrow2t+t\left(1+t^2\right)=2+2t^2\)
\(\Leftrightarrow t^3-2t^2+3t-2=0\)
\(\Leftrightarrow\left(t-1\right)\left(t^2-t+2\right)=0\)
\(\Leftrightarrow t=1\)
\(\Leftrightarrow tan\dfrac{x}{2}=1\)
\(\Leftrightarrow\dfrac{x}{2}=\dfrac{\pi}{4}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{2}+k2\pi\)
7.
\(\Leftrightarrow2\left(2cos^2x\right)^2=3+5\left(2cos^22x-1\right)\)
\(\Leftrightarrow2\left(cos2x+1\right)^2=10cos^22x-2\)
\(\Leftrightarrow2cos^22x-cos2x-1=0\)
Đặt \(cos2x=t\Rightarrow2t^2-t-1=0\)
\(\Rightarrow\left[{}\begin{matrix}t=1\\t=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\\cos2x=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=k2\pi\\2x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=\pm\dfrac{\pi}{3}+k\pi\end{matrix}\right.\)
9.
Đặt \(sinx+cosx=t\) với \(\left|t\right|\le\sqrt{2}\)
\(\Rightarrow t^2=sin^2x+cos^2x+2sinx.cosx=1+sin2x\)
\(\Rightarrow sin2x=t^2-1\)
Phương trình trở thành:
\(t+t^2-1+3=0\)
\(\Leftrightarrow t^2+t+2=0\) (vô nghiệm)