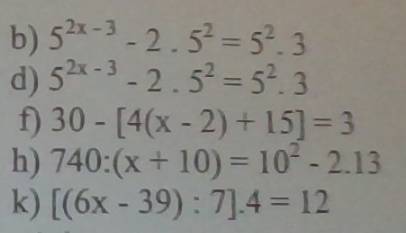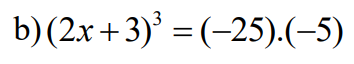b) \(5^{2x-3}-2\cdot5^2=5^2\cdot3\)
\(\Rightarrow5^{2x-3}=5^2\cdot3+5^2\cdot2\)
\(\Rightarrow5^{2x-3}=5^2\cdot5\)
\(\Rightarrow5^{2x-3}=5^3\)
\(\Rightarrow2x-3=3\)
\(\Rightarrow2x=6\)
\(\Rightarrow x=\dfrac{6}{2}\)
\(\Rightarrow x=3\)
f) \(30-\left[4\left(x-2\right)+15\right]=3\)
\(\Rightarrow4\left(x-2\right)+15=30-3\)
\(\Rightarrow4\left(x-2\right)+15=27\)
\(\Rightarrow4\left(x-2\right)=12\)
\(\Rightarrow x-2=3\)
\(\Rightarrow x=2+3\)
\(\Rightarrow x=5\)
h) \(740:\left(x+10\right)=10^2-2\cdot13\)
\(\Rightarrow740:\left(x+10\right)=100-26\)
\(\Rightarrow740:\left(x+10\right)=74\)
\(\Rightarrow x+10=740:74\)
\(\Rightarrow x+10=10\)
\(\Rightarrow x=0\)
k) \(\left[\left(6x-39\right):7\right].4=12\)
\(\Rightarrow\left(6x-39\right):7=3\)
\(\Rightarrow6x-39=3\cdot7\)
\(\Rightarrow6x-39=21\)
\(\Rightarrow6x=60\)
\(\Rightarrow x=\dfrac{60}{6}\)
\(\Rightarrow x=10\)
b: \(5^{2x-3}-2\cdot5^2=5^2\cdot3\)
=>\(5^{2x-3}=5^2\cdot3+5^2\cdot2=5^2\cdot5=5^3\)
=>2x-3=3
=>2x=6
=>x=3
d:
\(5^{2x-3}-2\cdot5^2=5^2\cdot3\)
=>\(5^{2x-3}=5^2\cdot3+5^2\cdot2=5^2\cdot5=5^3\)
=>2x-3=3
=>2x=6
=>x=3
f: \(30-\left[4\left(x-2\right)+15\right]=3\)
=>4(x-2)+15=30-3=27
=>4(x-2)=12
=>x-2=3
=>x=3+2=5
h: \(\dfrac{740}{x+10}=10^2-2\cdot13\)
=>\(\dfrac{740}{x+10}=100-26=74\)
=>x+10=10
=>x=0
k: \(\left[\dfrac{\left(6x-39\right)}{7}\right]\cdot4=12\)
=>\(\dfrac{\left(6x-39\right)}{7}=3\)
=>6x-39=3*7=21
=>6x=60
=>x=60/6=10